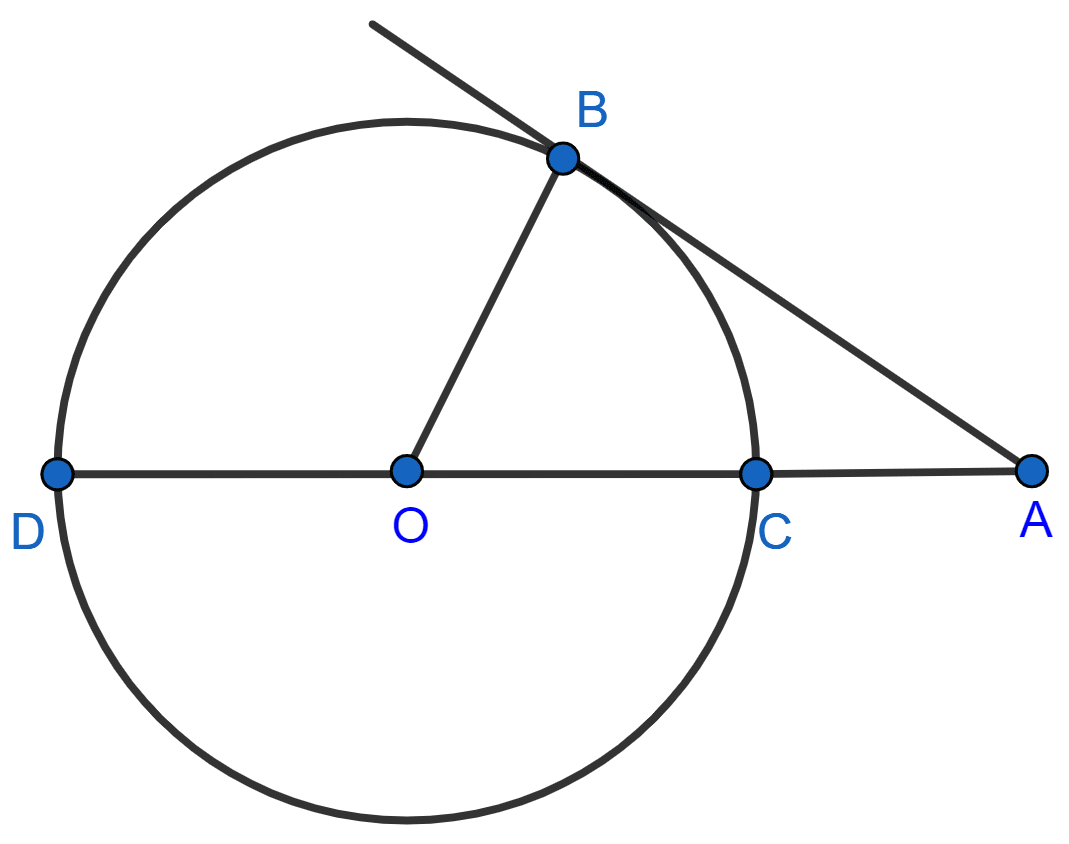
PA and PB are tangents to a circle with center O. If angle BPA = 70°, the angle ACB is :

1. 70°

2. 105°

3. 140°

4. 55°

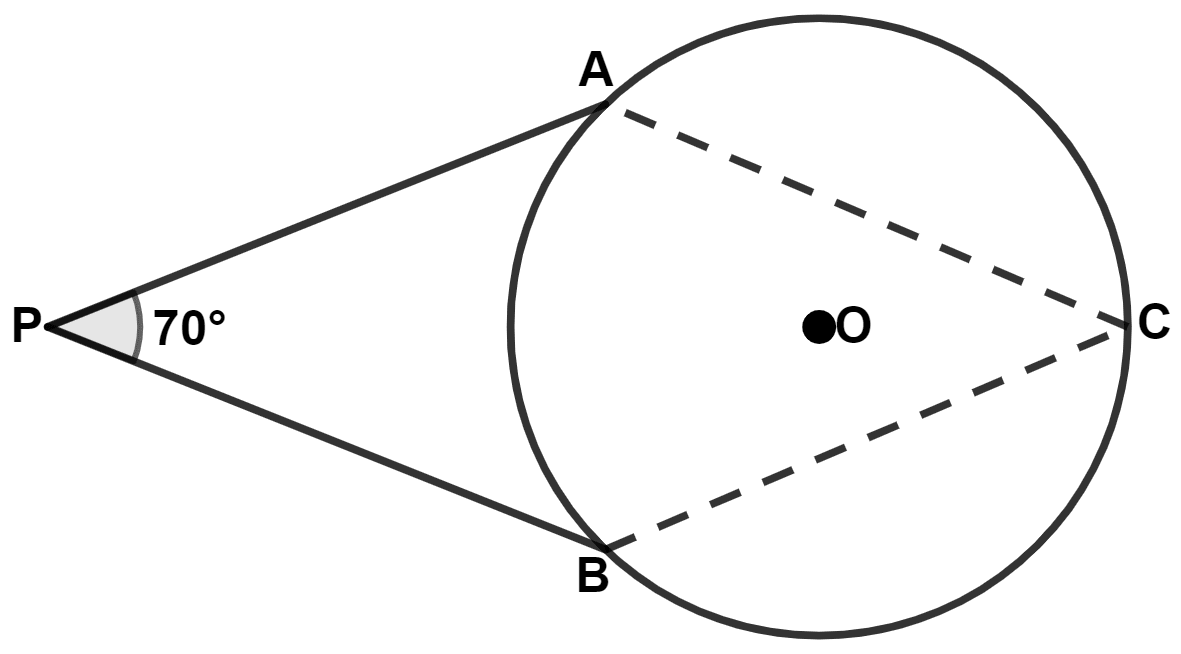
Join OA and OB.

We know that,

Tangent at any point of a circle and the radius through this point are perpendicular to each other.

∴ ∠OAP = 90° and ∠OBP = 90°

In quadrilateral OAPB,

⇒ ∠OAP + ∠APB + ∠PBO + ∠BOA = 360°

⇒ 90° + 70° + 90° + ∠BOA = 360°

⇒ ∠BOA + 250° = 360°

⇒ ∠BOA = 360° - 250° = 110°.

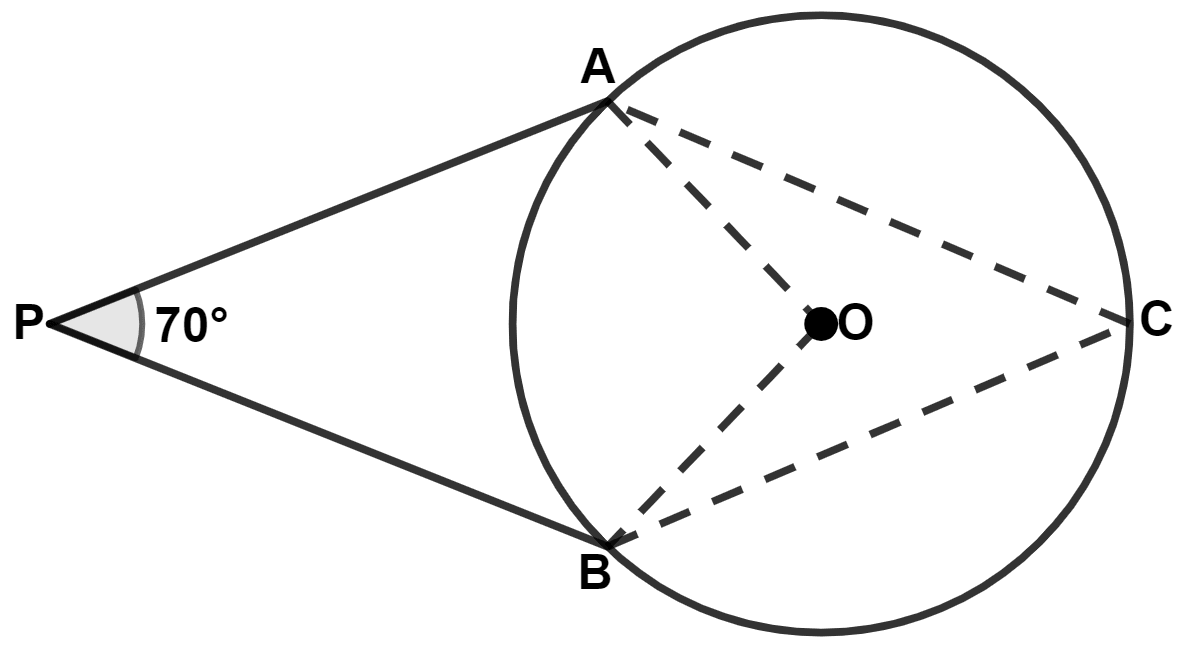
We know that,

The angle which an arc of a circle subtends at the center is double that which it subtends at any point on the remaining part of the circumference.

∴ ∠AOB = 2∠ACB

⇒ ∠ACB = $\dfrac{1}{2}$ ∠AOB

⇒ ∠ACB = $\dfrac{1}{2} \times 110°$ = 55°.

Hence, Option 4 is the correct option.