Mathematics
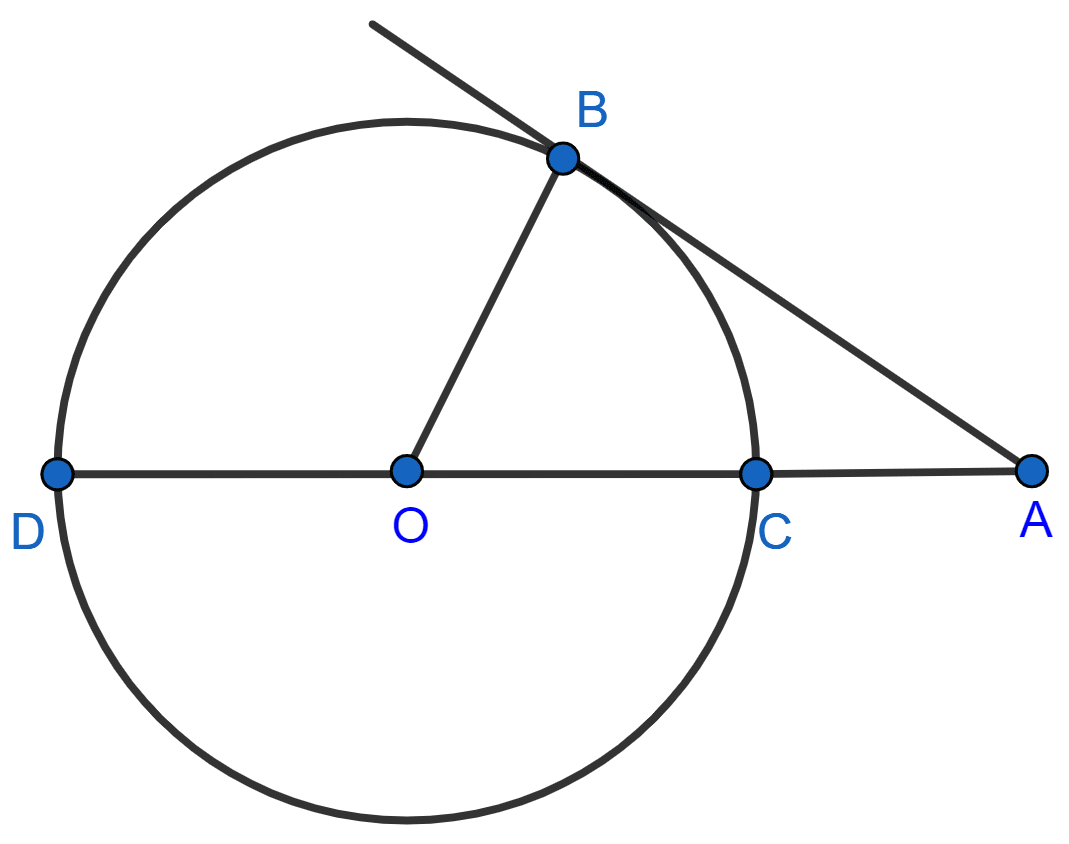
In the given figure, O is the centre of the circle and AB is a tangent to the circle at B. If AB = 15 cm and AC = 7.5 cm, calculate the radius of the circle.
Circles
23 Likes
Answer
Let the radius of the circle to be r cm.
So, AO = AC + OC = (7.5 + r) cm.
In right ∆AOB, we have
⇒ AO2 = AB2 + OB2 [By Pythagoras Theorem]
⇒ (7.5 + r)2 = 152 + r2
⇒ 56.25 + r2 + 15r = 225 + r2
⇒ 15r = 225 - 56.25
⇒ 15r = 168.75
⇒ r = = 11.25 cm.
Hence, radius of circle = 11.25 cm.
Answered By
13 Likes
Related Questions
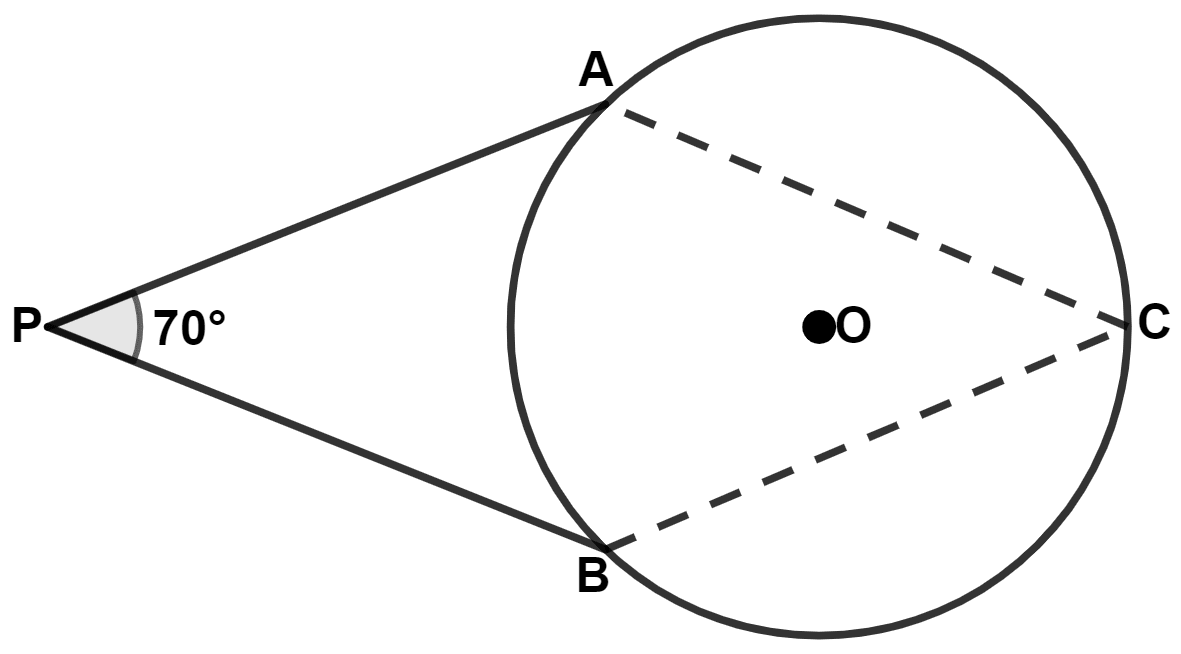
PA and PB are tangents to a circle with center O. If angle BPA = 70°, the angle ACB is :
70°
105°
140°
55°
The radius of a circle is 8 cm. Calculate the length of a tangent drawn to this circle from a point at a distance of 10 cm from its centre.
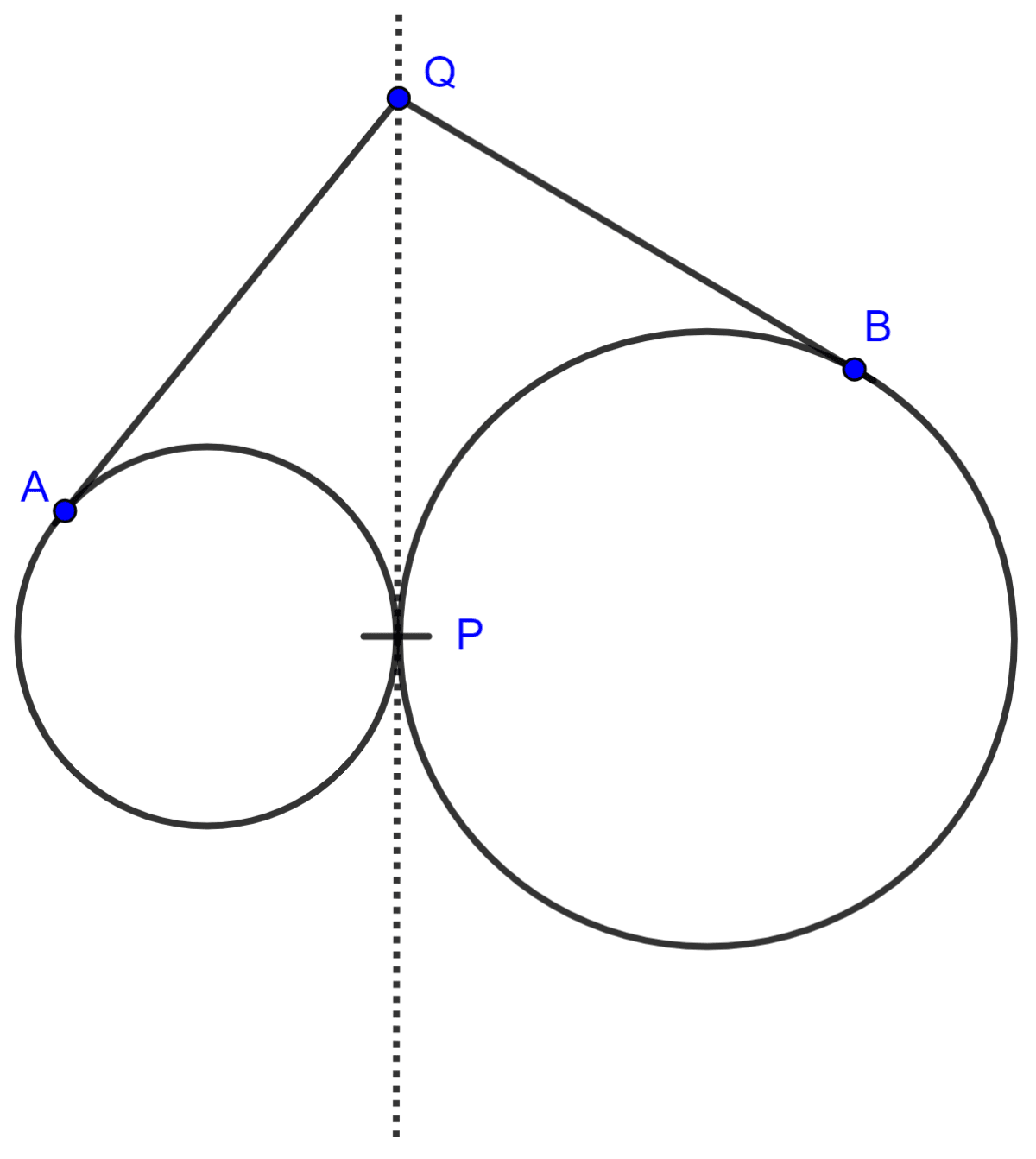
Two circles touch each other externally at point P. Q is a point on the common tangent through P. Prove that the tangents QA and QB are equal.
Two circles of radii 5 cm and 3 cm are concentric. Calculate the length of a chord of the outer circle which touches the inner.