Mathematics
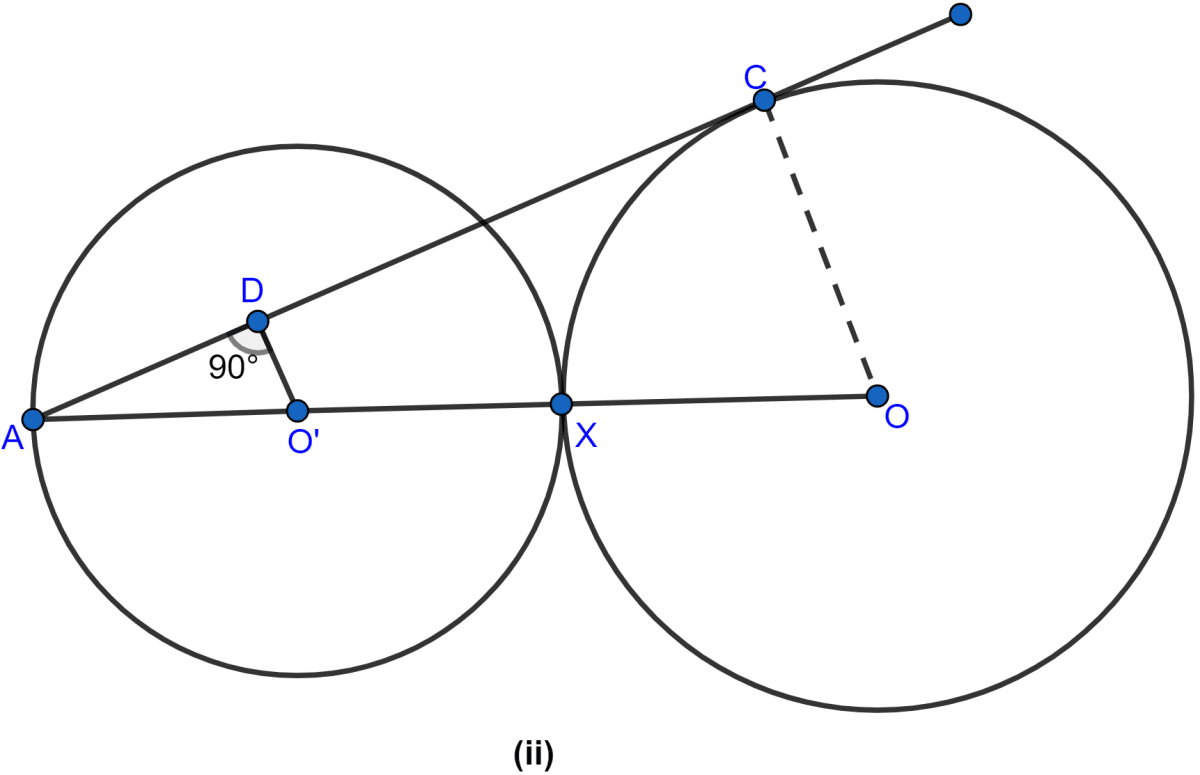
In the figure (ii) given below, equal circles with centres O and O' touch each other at X. OO' is produced to meet a circle O' at A. AC is tangent to the circle whose centre is O. O'D is perpendicular to AC. Find the value of
(i)
(ii)
Circles
31 Likes
Answer
From figure,
OC is radius and AC is tangent, then OC ⊥ AC.
Let radius of each equal circle = r.
(i) From figure,
AO = AO' + O'X + XO and AO' = O'X = XO = r (radius of circle)
AO = r + r + r = 3r.
Hence, the value of .
(ii) Considering △ADO' and △ACO
∠A = ∠A (Common angles)
∠D = ∠C (Both are equal to 90°)
∴ By AA axiom △ADO' ~ △ACO.
Since triangles are similar hence the ratio of their areas is equal to the ratio of the square of the corresponding sides.
Hence, the value of
Answered By
18 Likes
Related Questions
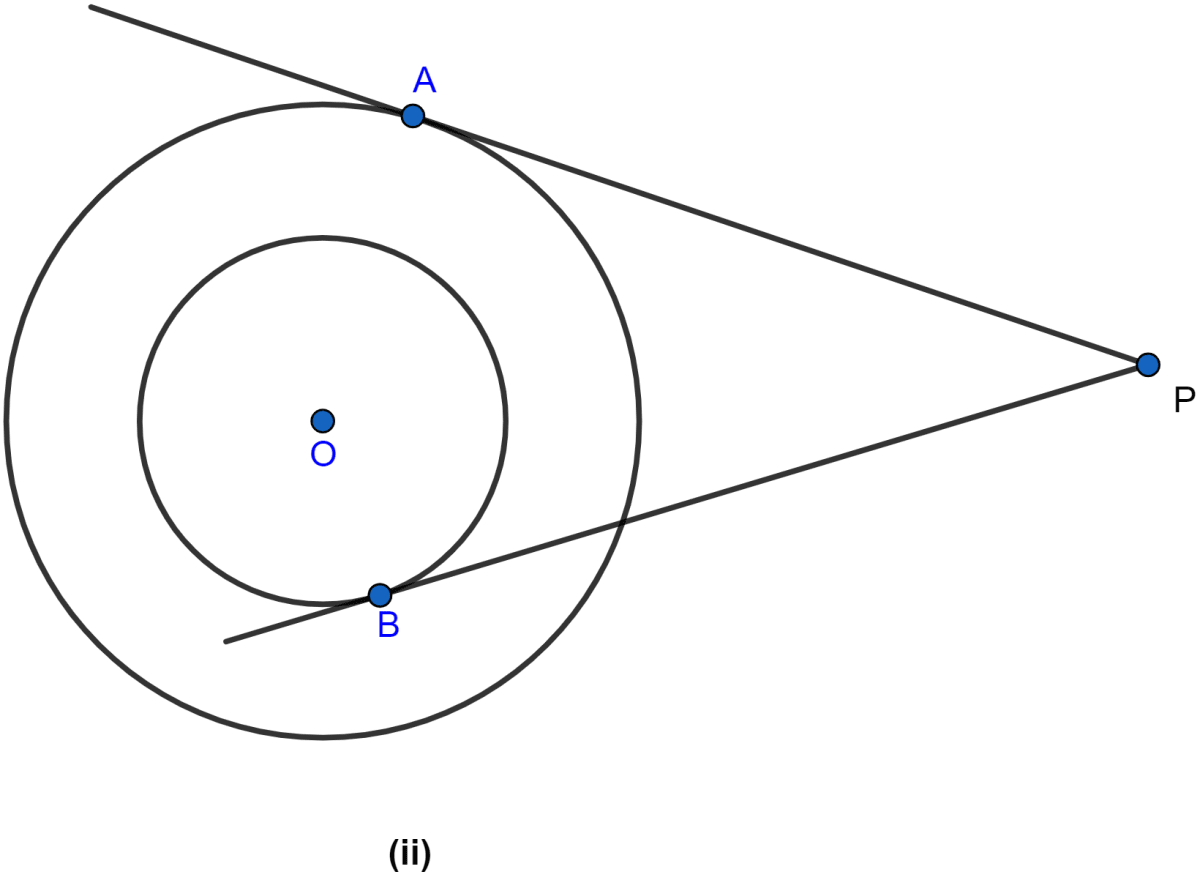
In the figure (ii) given below, two concentric circles with centre O are of radii 5 cm and 3 cm. From an external point P, tangents PA and PB are drawn to these circles. If AP = 12 cm, find BP.
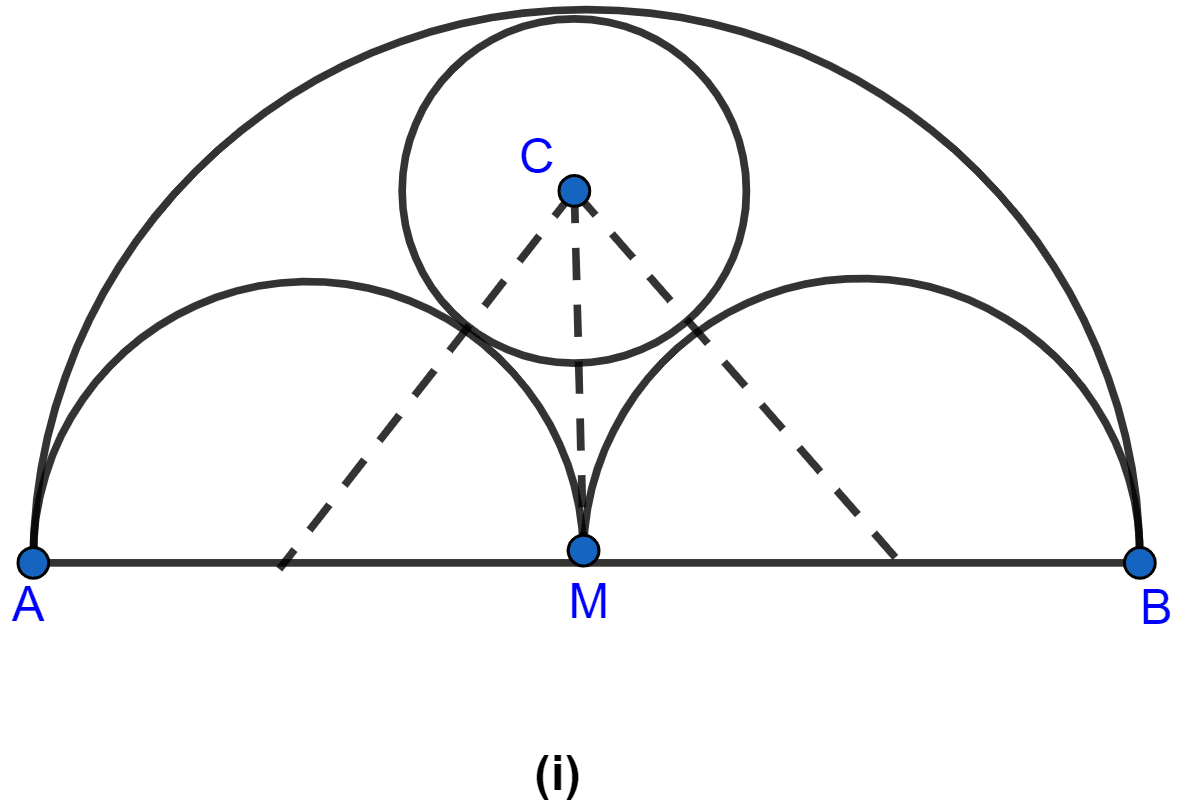
In the figure (i) given below, AB = 8 cm and M is mid-point of AB. Semicircles are drawn on AB, AM and MB as diameters. A circle with centre C touches all three semicircles as shown, find its radius.
The length of the direct common tangent to two circles of radii 12 cm and 4 cm is 15 cm. Calculate the distance between their centres.
Calculate the length of a direct common tangent to two circles of radii 3 cm and 8 cm with their centres 13 cm apart.