Mathematics
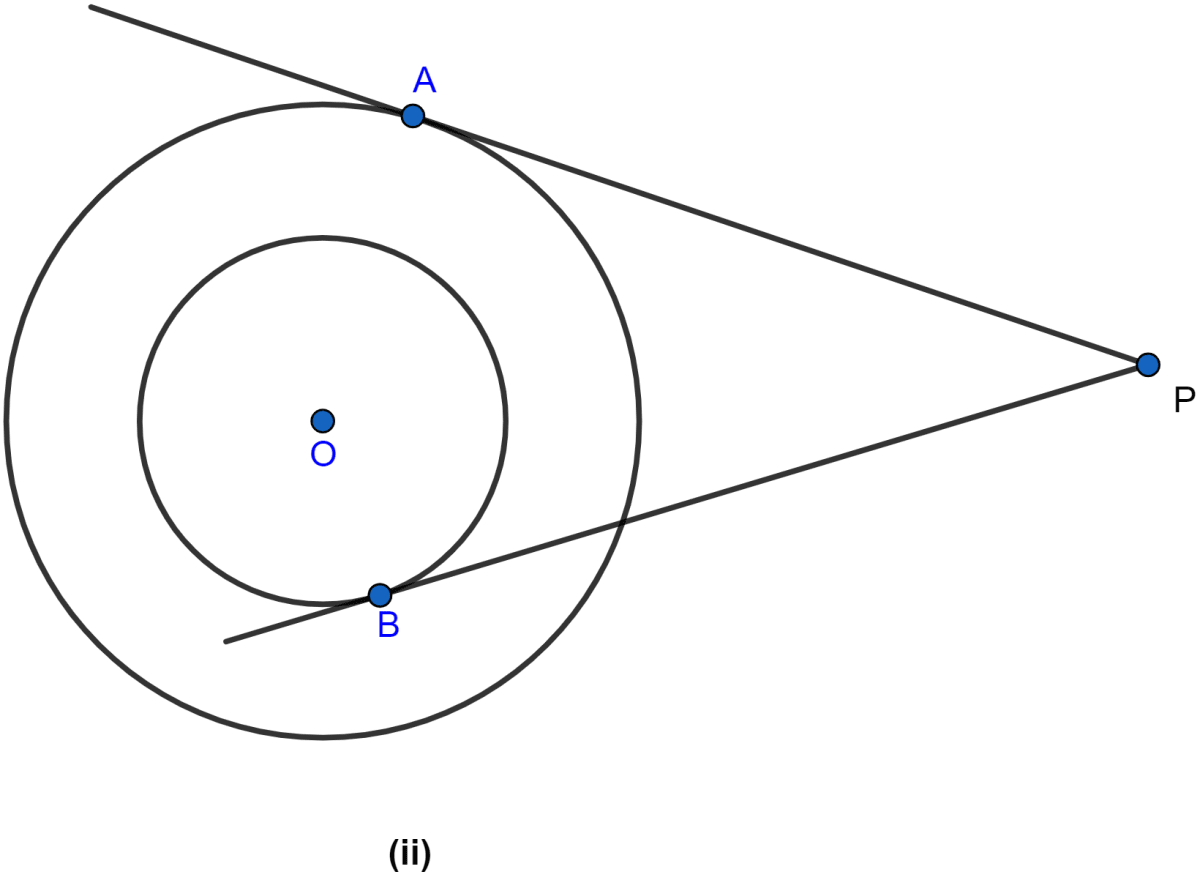
In the figure (ii) given below, two concentric circles with centre O are of radii 5 cm and 3 cm. From an external point P, tangents PA and PB are drawn to these circles. If AP = 12 cm, find BP.
Circles
18 Likes
Answer
Join OA and OB.
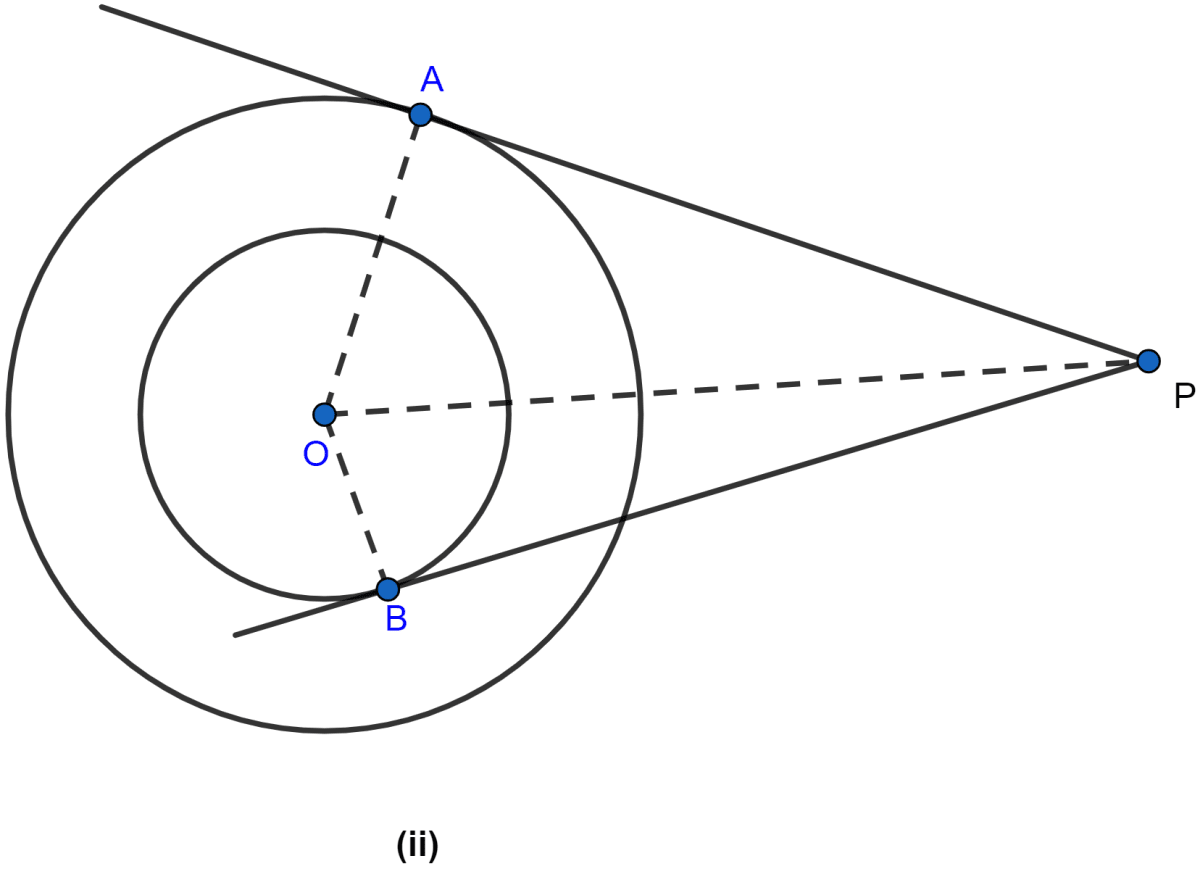
OA ⊥ AP. (∵ tangent at a point and radius through the point are perpendicular to each other.)
So, in right angled triangle OAP,
OB ⊥ BP. (∵ tangent at a point and radius through the point are perpendicular to each other.)
So, in right angled triangle OBP,
Hence, the length of BP = cm.
Answered By
12 Likes
Related Questions
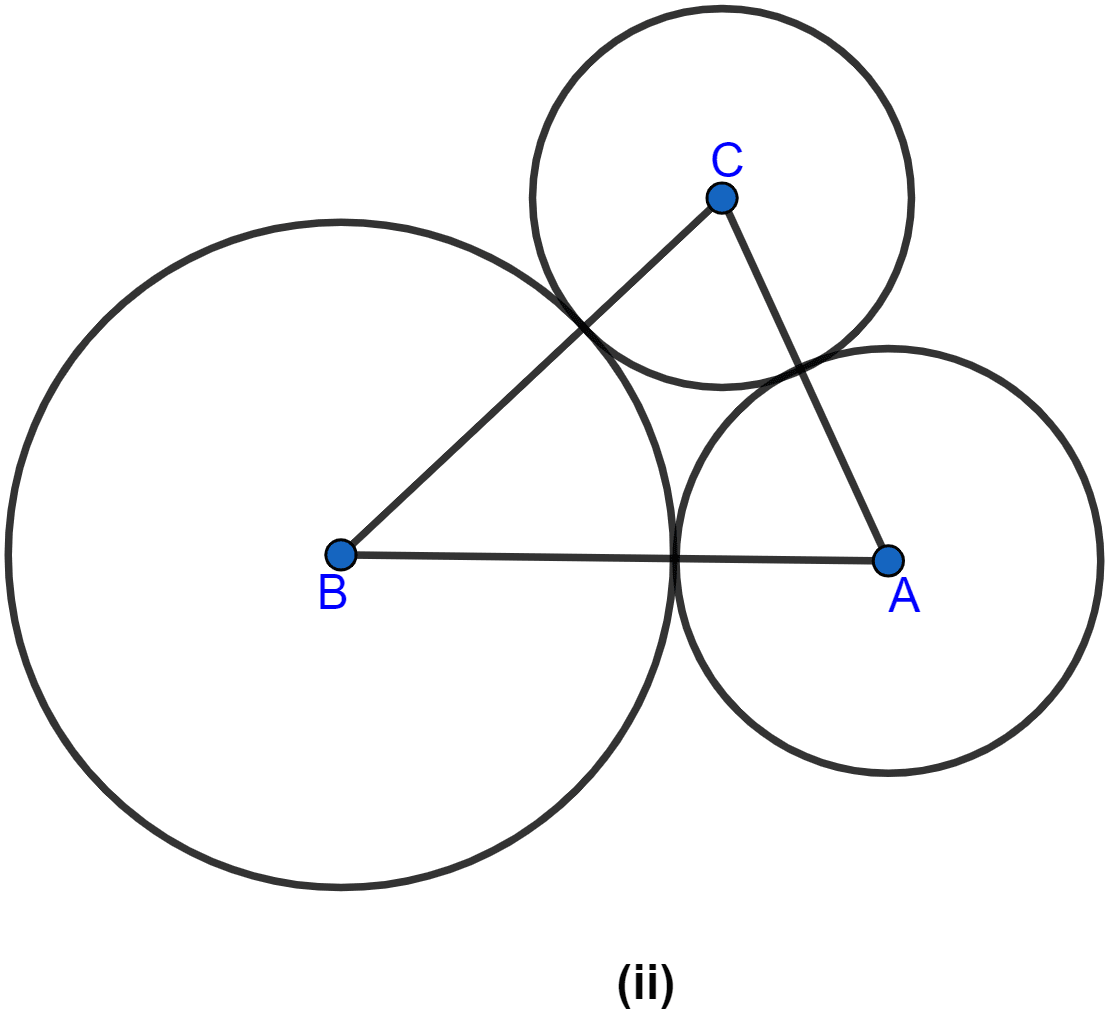
In the figure (ii) given below, ABC is a triangle with AB = 10 cm, BC = 8 cm and AC = 6 cm (not drawn to scale). Three circles are drawn touching each other with vertices A, B and C as their centres. Find the radii of the three circles.
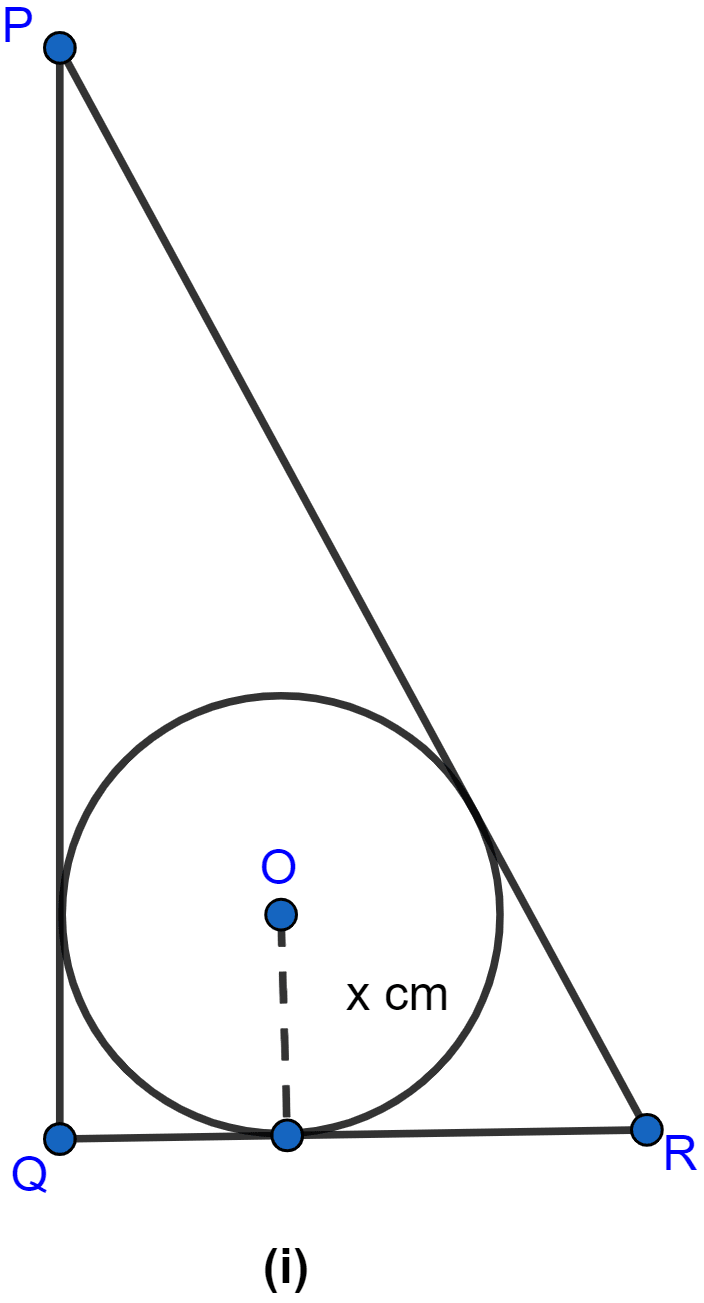
In the figure (i) given below, PQ = 24 cm, QR = 7 cm and ∠PQR = 90°. Find the radius of the inscribed circle of △PQR.
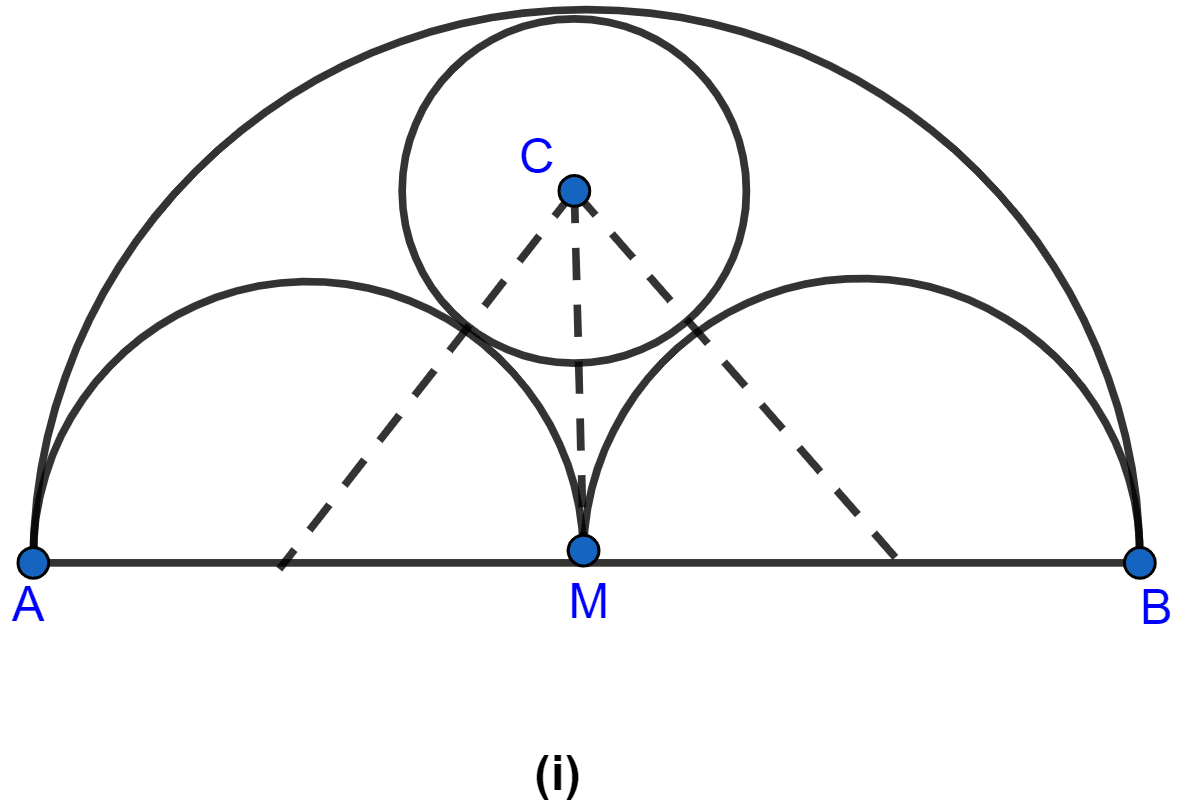
In the figure (i) given below, AB = 8 cm and M is mid-point of AB. Semicircles are drawn on AB, AM and MB as diameters. A circle with centre C touches all three semicircles as shown, find its radius.
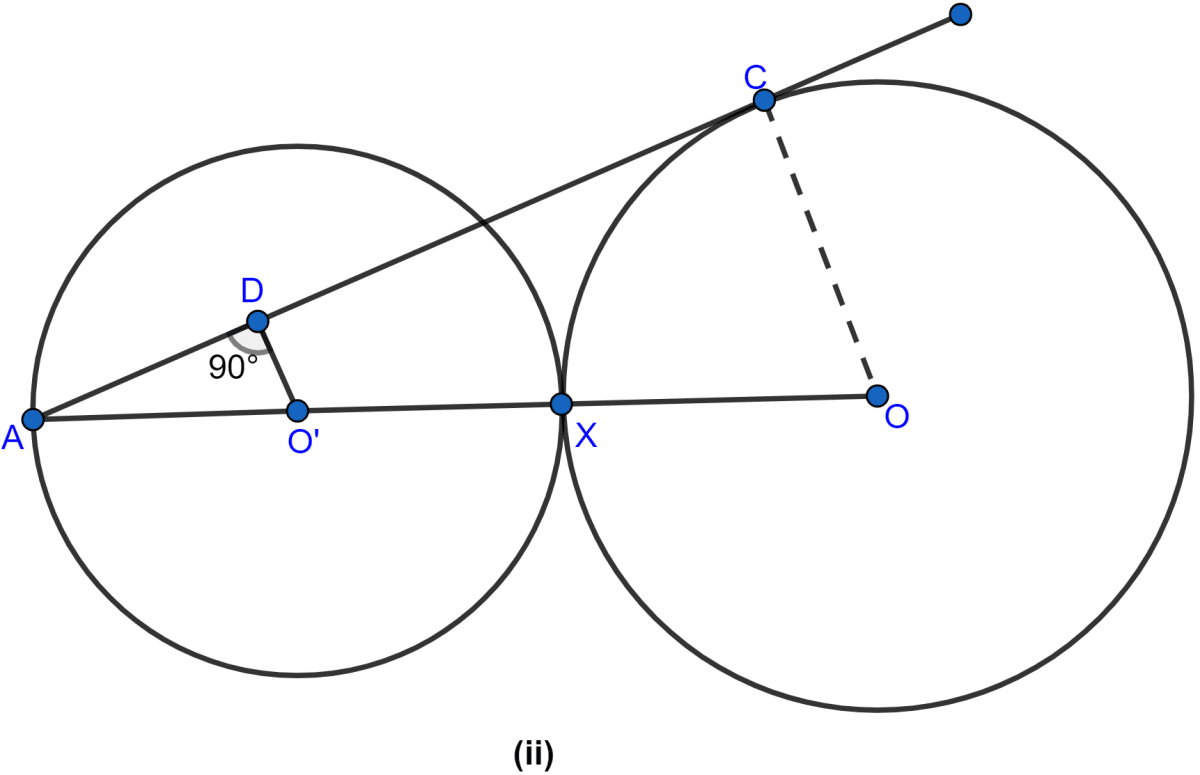
In the figure (ii) given below, equal circles with centres O and O' touch each other at X. OO' is produced to meet a circle O' at A. AC is tangent to the circle whose centre is O. O'D is perpendicular to AC. Find the value of
(i)
(ii)