Mathematics
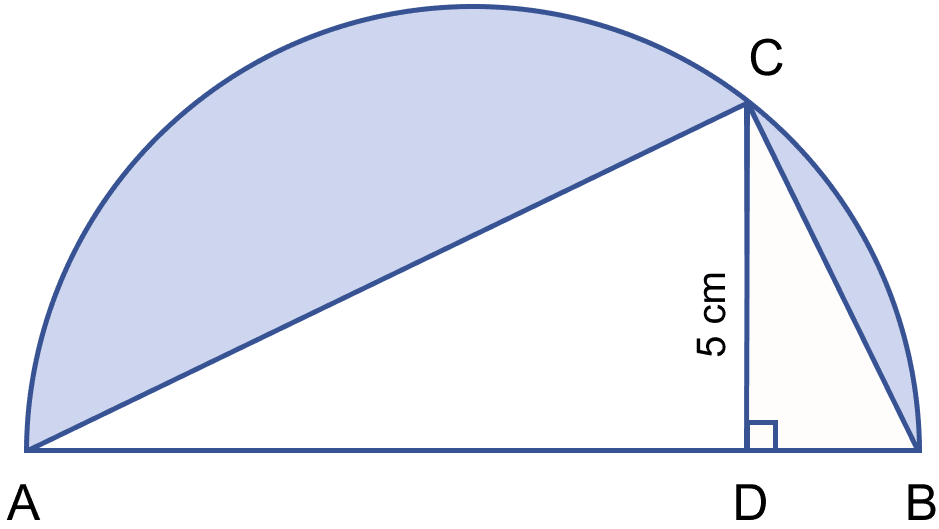
In the figure (ii) given below, area of △ABC = 35 cm2. Find the area of the shaded region.
Mensuration
53 Likes
Answer
From figure,
Area of △ABC = base × height
Substituting values we get,
⇒ AB × CD = 35
⇒ AB × 5 = 35
⇒ AB = = 14 cm.
From figure,
AB is the diameter of semicircle.
Radius = = 7 cm.
Area of semi-circle =
= 11 × 7
= 77 cm2.
Area of shaded region = Area of semi-circle - Area of △ABC
= 77 - 35
= 42 cm2.
Hence, area of shaded region = 42 cm2.
Answered By
32 Likes
Related Questions
In the figure (iii) given below, the diameter of the semicircle is equal to 14 cm. Calculate the area of the shaded region. Take π = .
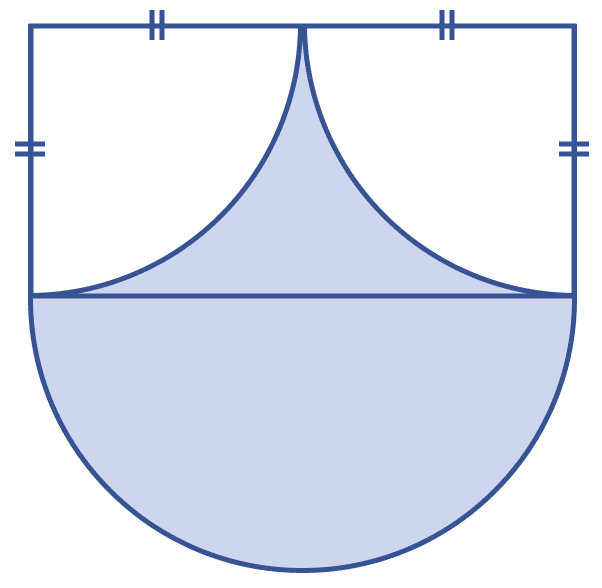
Find the area and the perimeter of the shaded region in figure (i) given below. The dimensions are in centimeters.

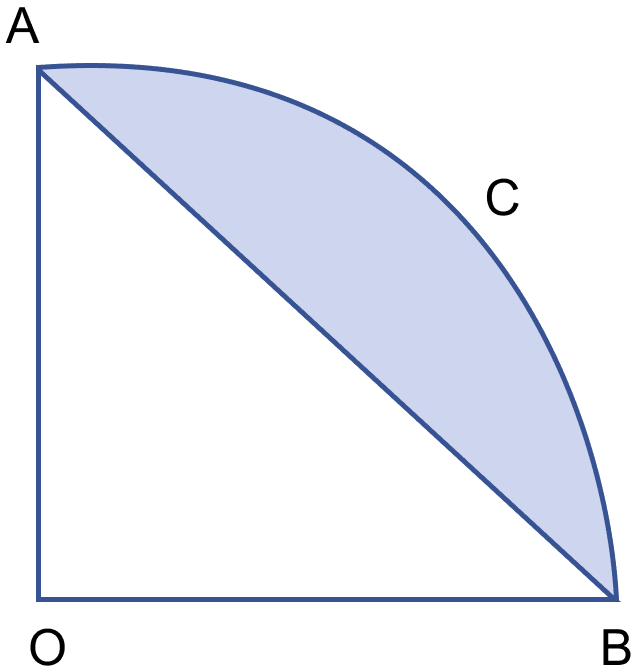
In the figure (i) given below, AOBC is a quadrant of a circle of radius 10 m. Calculate the area of the shaded portion. Take π = 3.14 and give your answer correct to two significant figures.
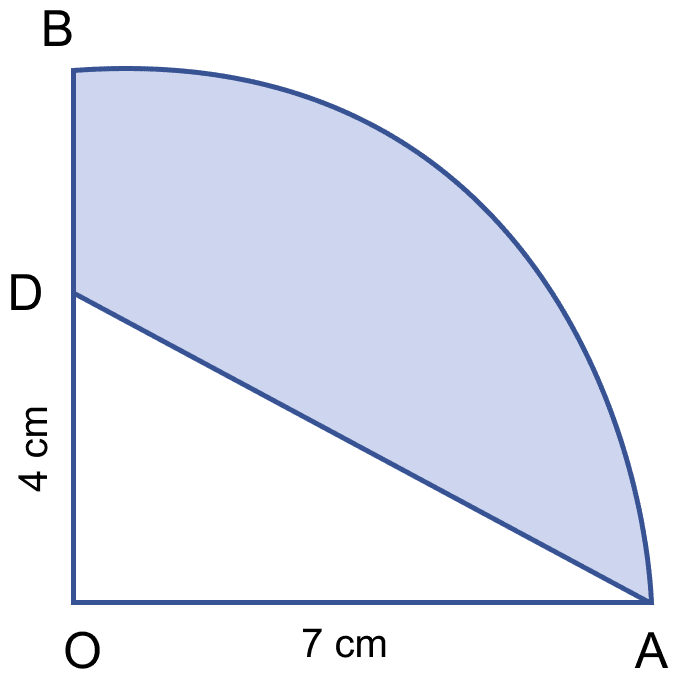
In the figure (ii) given below, OAB is a quadrant of a circle. The radius OA = 7 cm and OD = 4 cm. Calculate the area of the shaded portion.