Mathematics
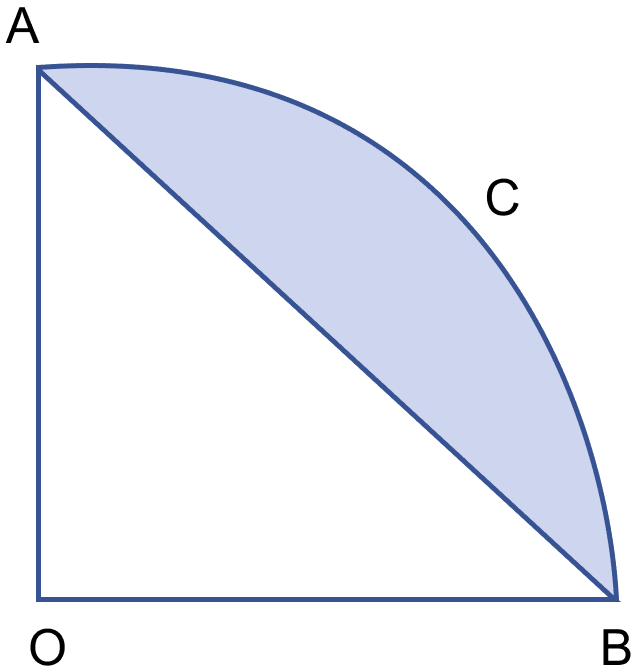
In the figure (i) given below, AOBC is a quadrant of a circle of radius 10 m. Calculate the area of the shaded portion. Take π = 3.14 and give your answer correct to two significant figures.
Mensuration
27 Likes
Answer
Area of quadrant =
Area of triangle AOB =
Area of shaded region = Area of quadrant - Area of triangle
= 78.5 - 50
= 28.5 ≈ 29 m2.
Hence, area of shaded region = 29 m2.
Answered By
18 Likes
Related Questions
Find the area and the perimeter of the shaded region in figure (i) given below. The dimensions are in centimeters.

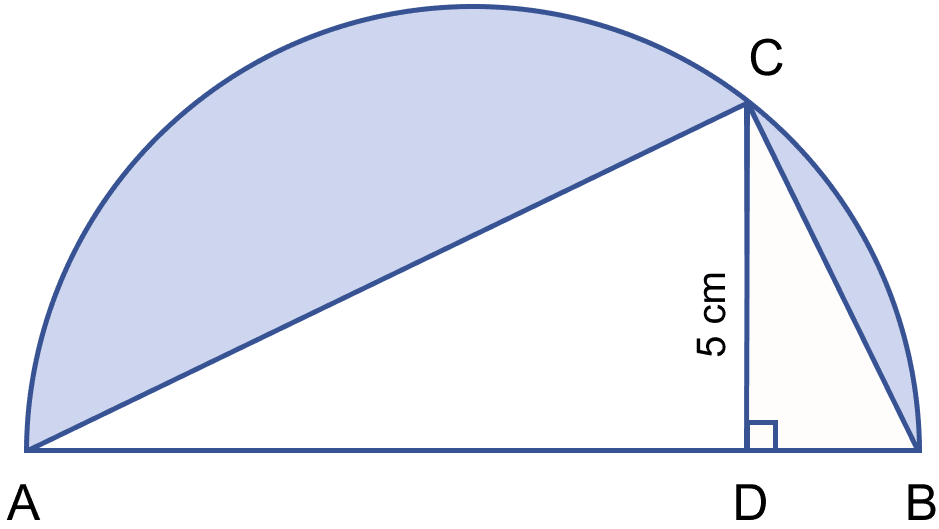
In the figure (ii) given below, area of △ABC = 35 cm2. Find the area of the shaded region.
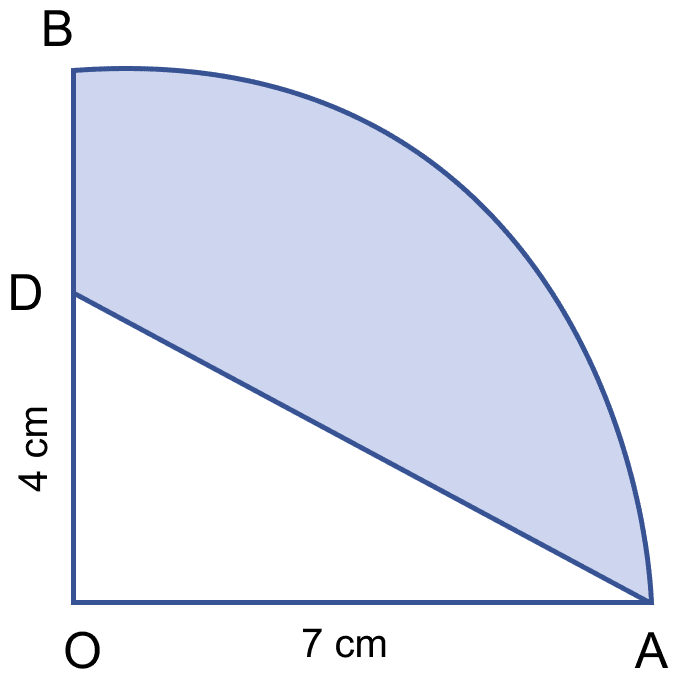
In the figure (ii) given below, OAB is a quadrant of a circle. The radius OA = 7 cm and OD = 4 cm. Calculate the area of the shaded portion.
A student takes a rectangular piece of paper 30 cm long and 21 cm wide. Find the area of the biggest circle that can be cut out from the paper. Also find the area of the paper left after cutting out the circle.