Mathematics
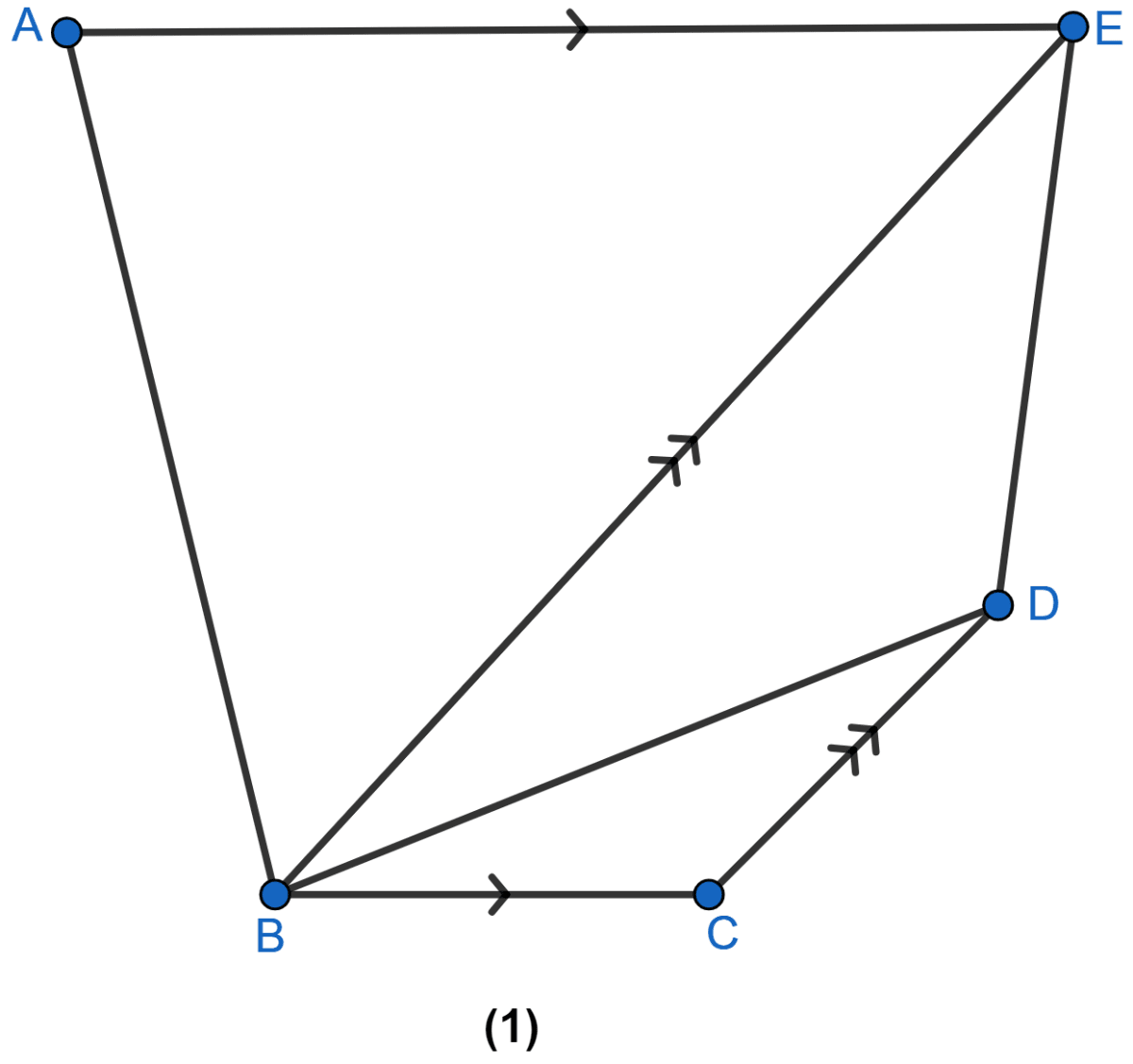
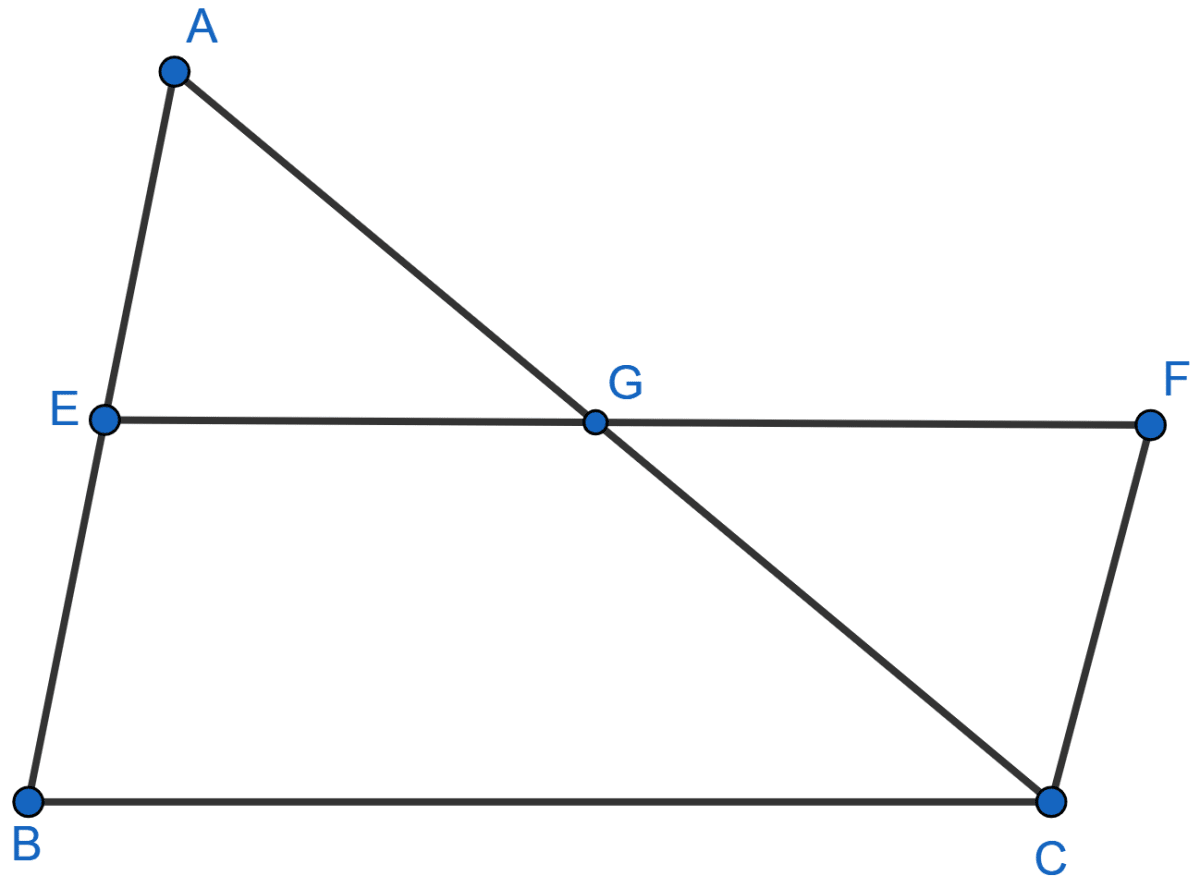
In the figure (1) given below, BC || AE and CD || BE. Prove that
area of ∆ABC= area of ∆EBD.
Theorems on Area
22 Likes
Answer
Join CE and AC.
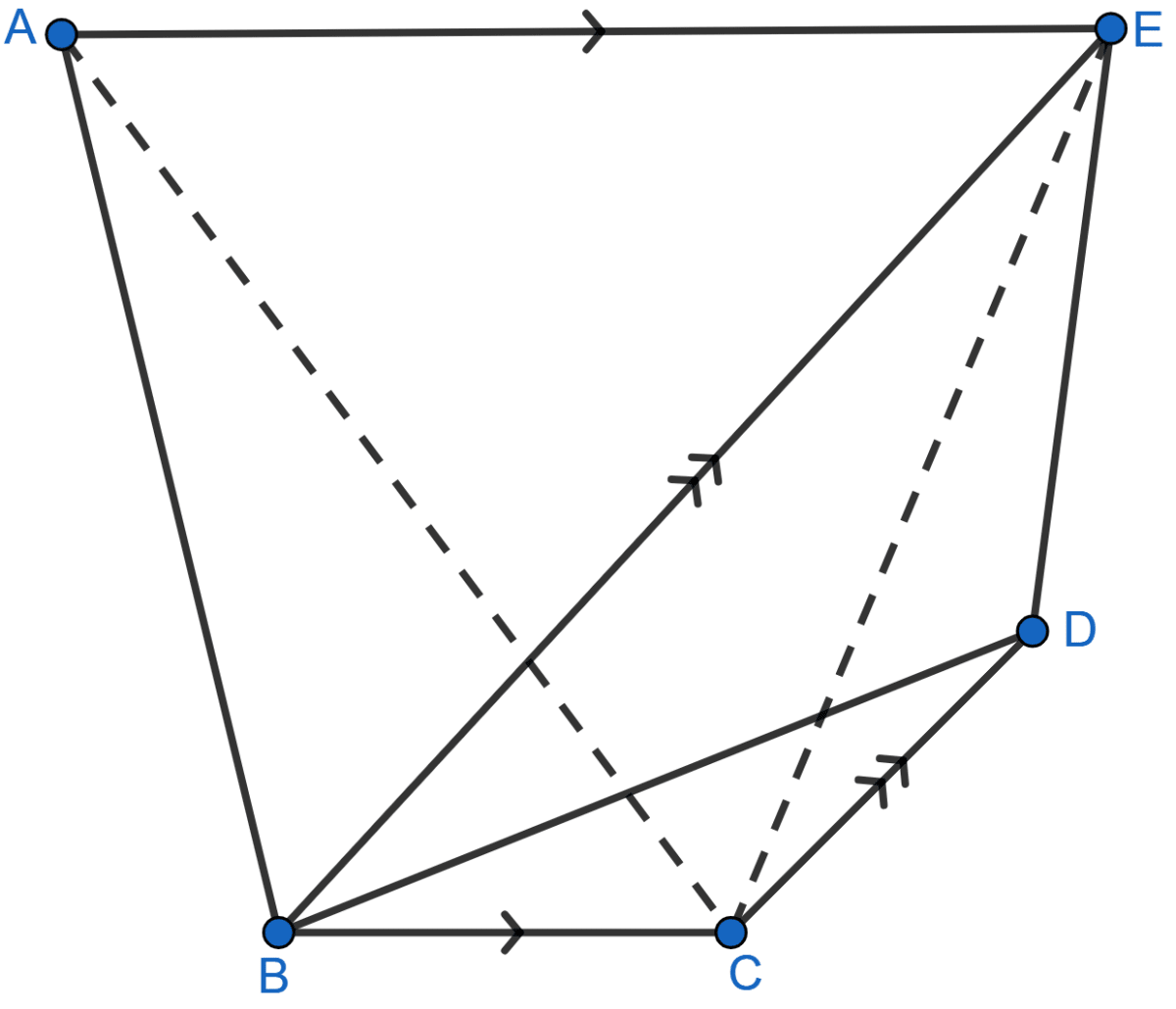
From figure,
∆ABC and ∆EBC lie on same base BC and between same parallel lines BC and AE.
∴ area of ∆ABC = area of ∆EBC ……. (i)
From figure,
∆EBC and ∆EBD lie on same base BE and between same parallel lines CD and BE.
∴ area of ∆EBC = area of ∆EBD ……. (ii)
From (i) and (ii), we get
area of ∆ABC = area of ∆EBD.
Hence, proved that area of ∆ABC = area of ∆EBD.
Answered By
15 Likes
Related Questions
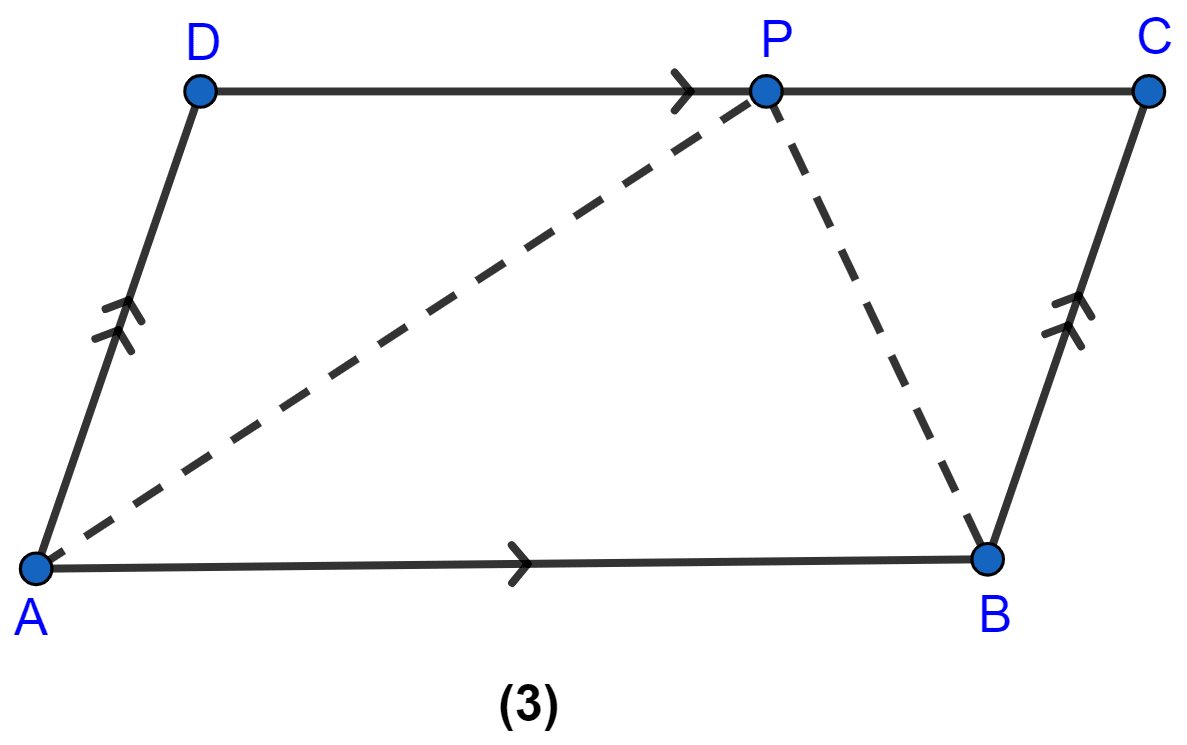
In the figure(3) given below, ABCD is a parallelogram. P is a point on DC such that area of ∆DAP = 25 cm2 and the area of ∆BCP = 15 cm2. Find
(i) area of || gm ABCD
(ii) DP : PC.
In the adjoining figure, E is the midpoint of the side AB of a triangle ABC and EBCF is a parallelogram. If the area of ∆ ABC is 25 sq. units, find the area of || gm EBCF.
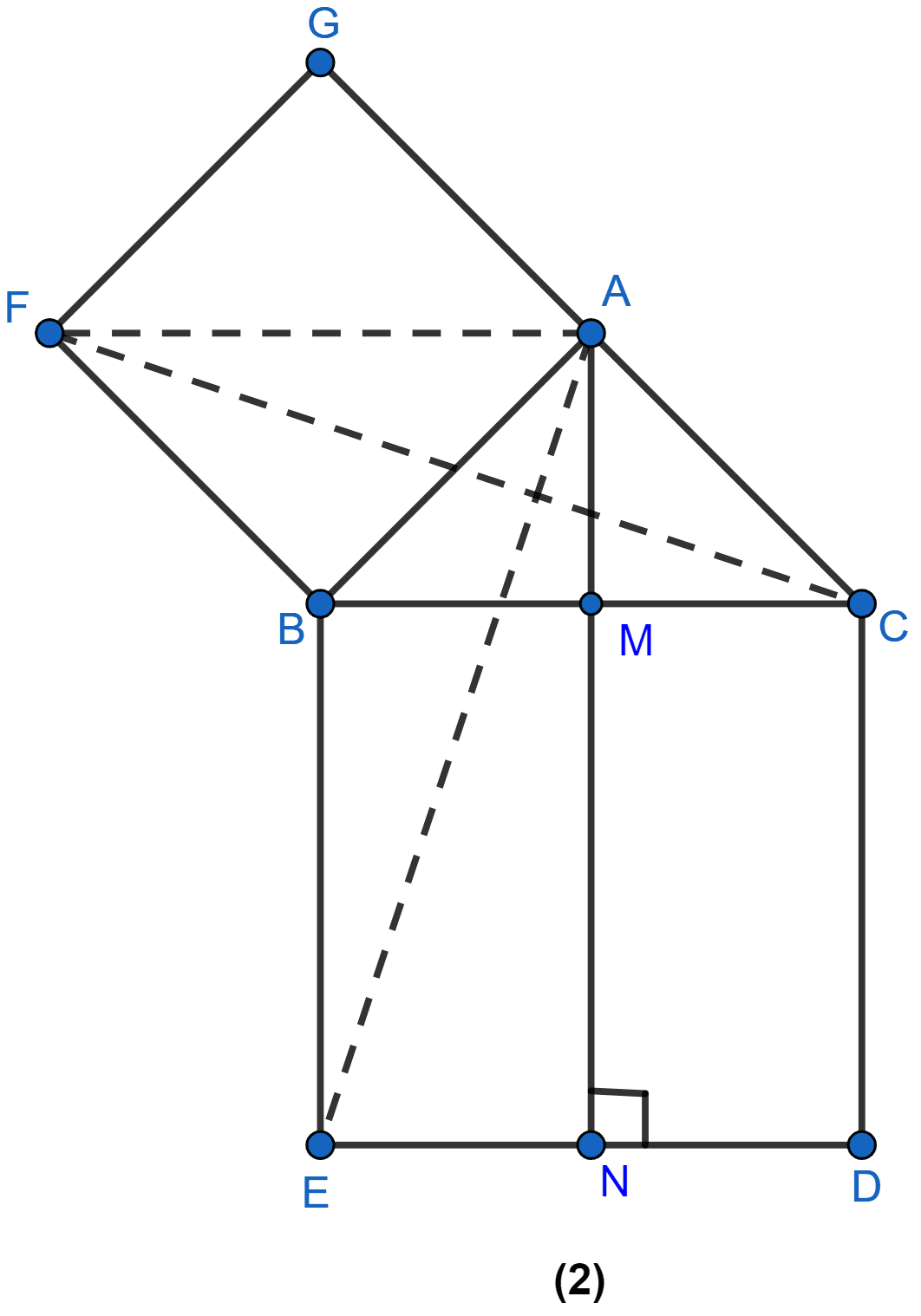
In figure (2) given below, ABC is a right-angled triangle at A. AGFB is a square on the side AB and BCDE is a square on the hypotenuse BC. If AN ⊥ ED, prove that
(i) ∆BCF ≅ ∆ABE.
(ii) area of square ABFG = area of rectangle BENM.
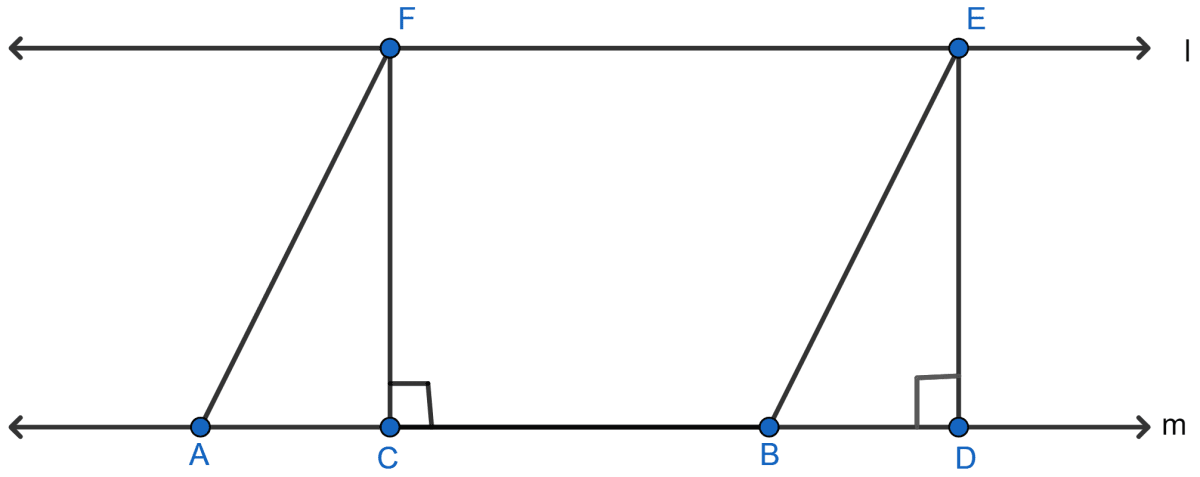
In the adjoining figure, if l || m, AF || BE, FC ⊥ m and ED ⊥ m, then the correct statement is
area of || ABEF = area of rect. CDEF
area of || ABEF = area of quad. CBEF
area of || ABEF = 2 area of △ACF
area of || ABEF = 2 area of △EBD