Mathematics
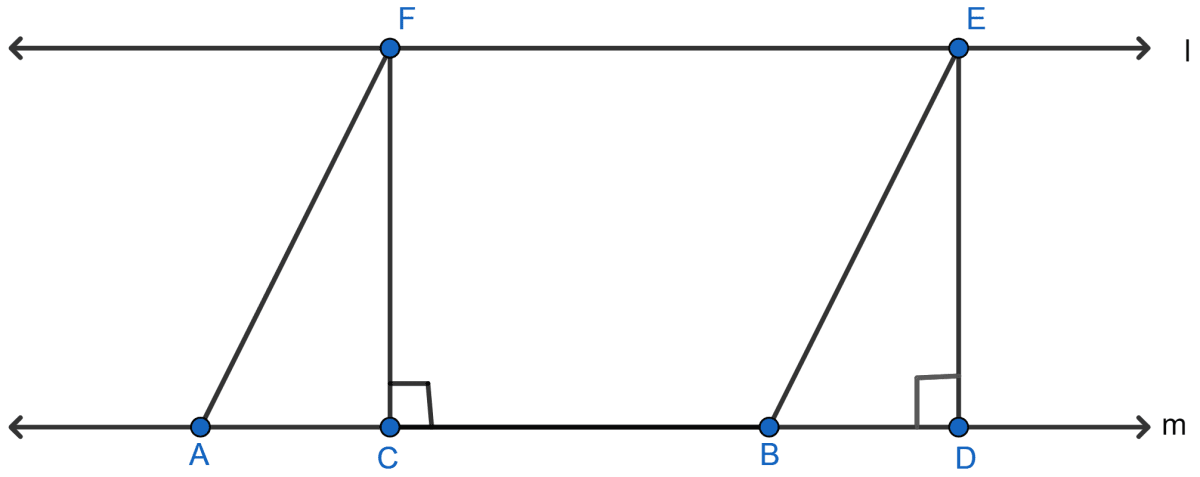
In the adjoining figure, if l || m, AF || BE, FC ⊥ m and ED ⊥ m, then the correct statement is
area of || ABEF = area of rect. CDEF
area of || ABEF = area of quad. CBEF
area of || ABEF = 2 area of △ACF
area of || ABEF = 2 area of △EBD
Theorems on Area
1 Like
Answer
We know that,
A parallelogram and a rectangle on the same base and between the same parallel lines are equal in area.
Since, || ABEF and rectangle CDEF are on same base EF and between same parallel lines l and m.
∴ area of || ABEF = area of rect. CDEF.
Hence, Option 1 is the correct option.
Answered By
3 Likes
Related Questions
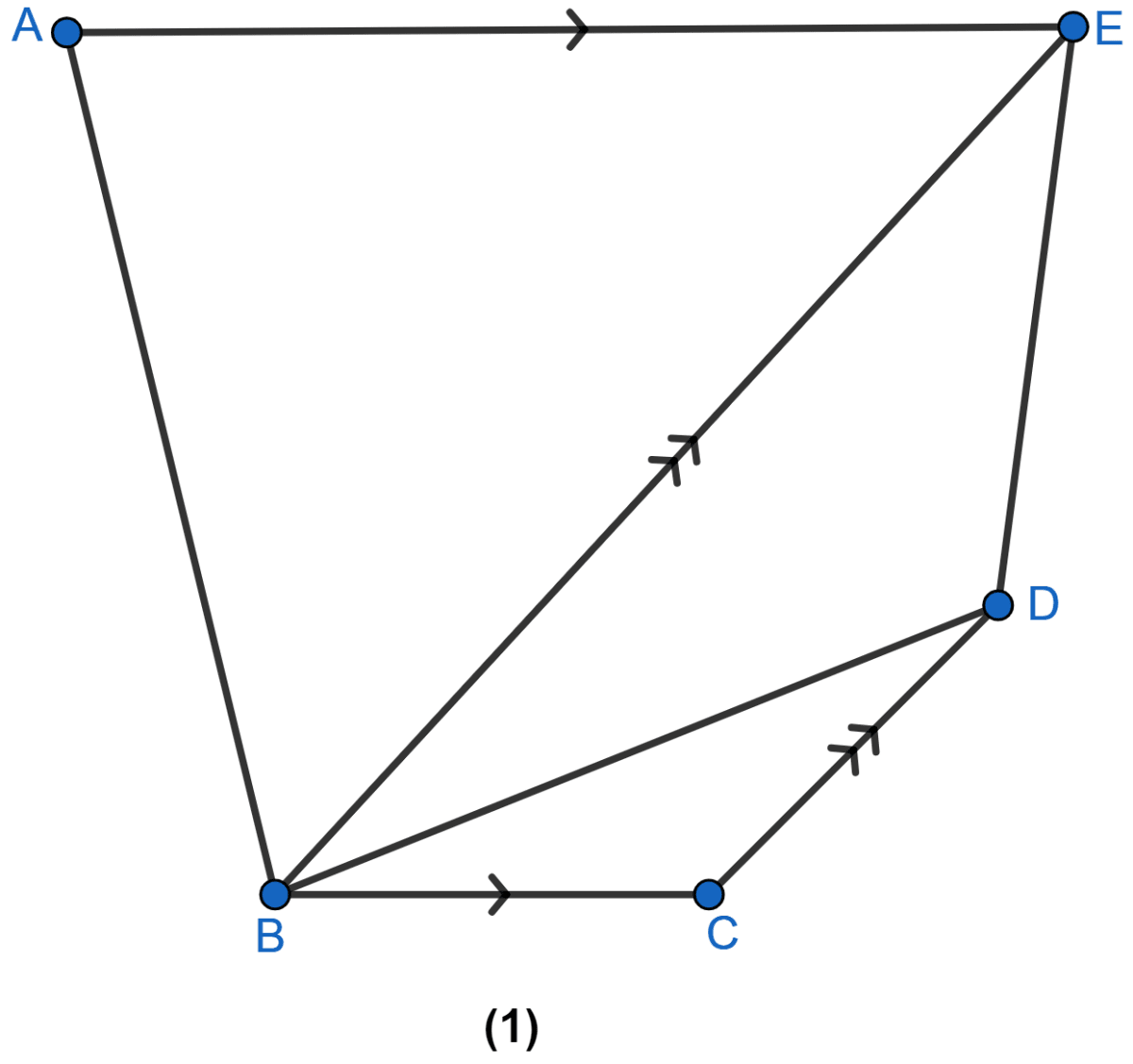
In the figure (1) given below, BC || AE and CD || BE. Prove that
area of ∆ABC= area of ∆EBD.
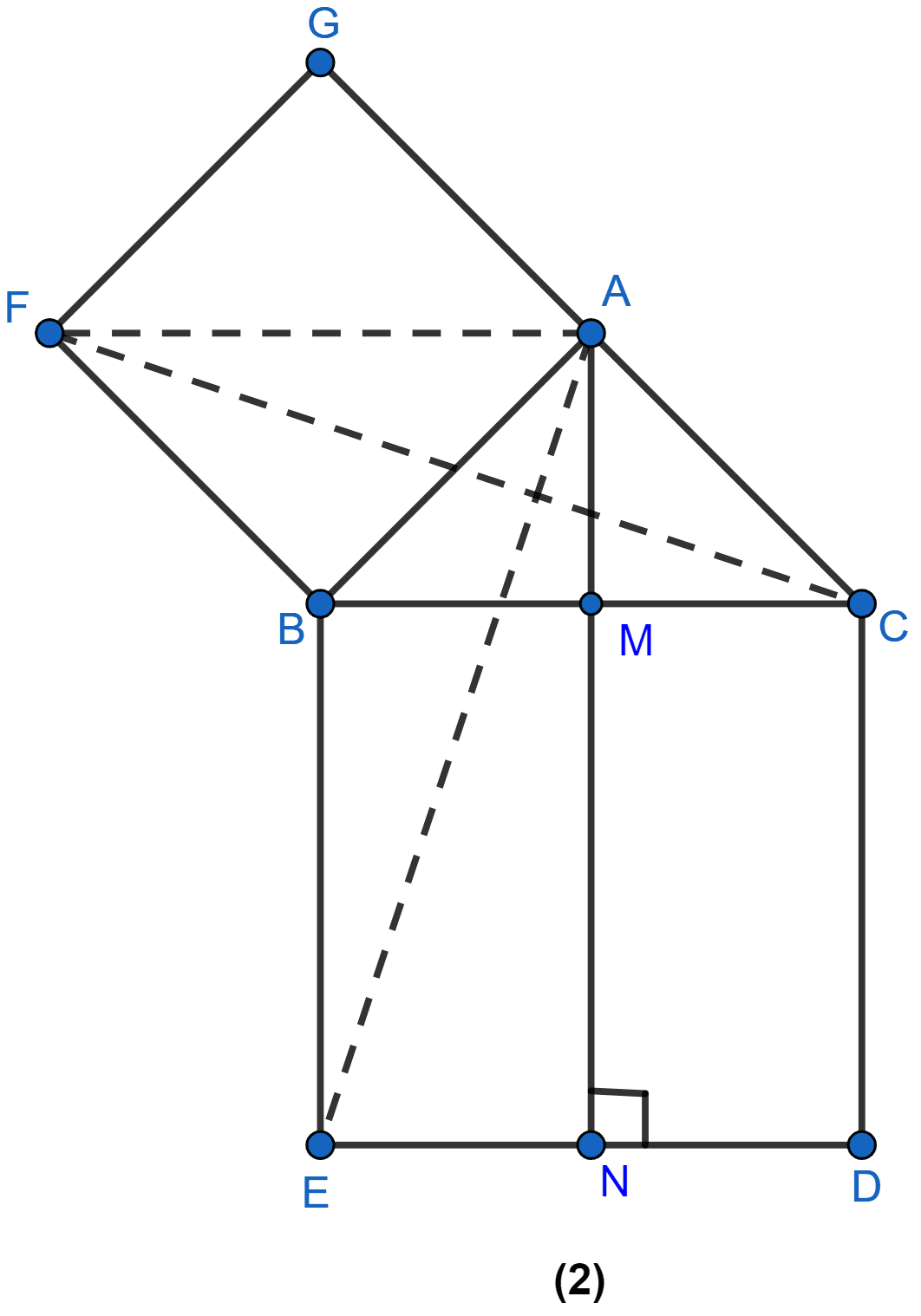
In figure (2) given below, ABC is a right-angled triangle at A. AGFB is a square on the side AB and BCDE is a square on the hypotenuse BC. If AN ⊥ ED, prove that
(i) ∆BCF ≅ ∆ABE.
(ii) area of square ABFG = area of rectangle BENM.
Two parallelograms are on equal bases and between the same parallels. The ratio of their areas is
1 : 2
1 : 1
2 : 1
3 : 1
If a triangle and a parallelogram are on the same base and between same parallels, then the ratio of area of the triangle to the area of parallelogram is
1 : 3
1 : 2
3 : 1
1 : 4