Mathematics
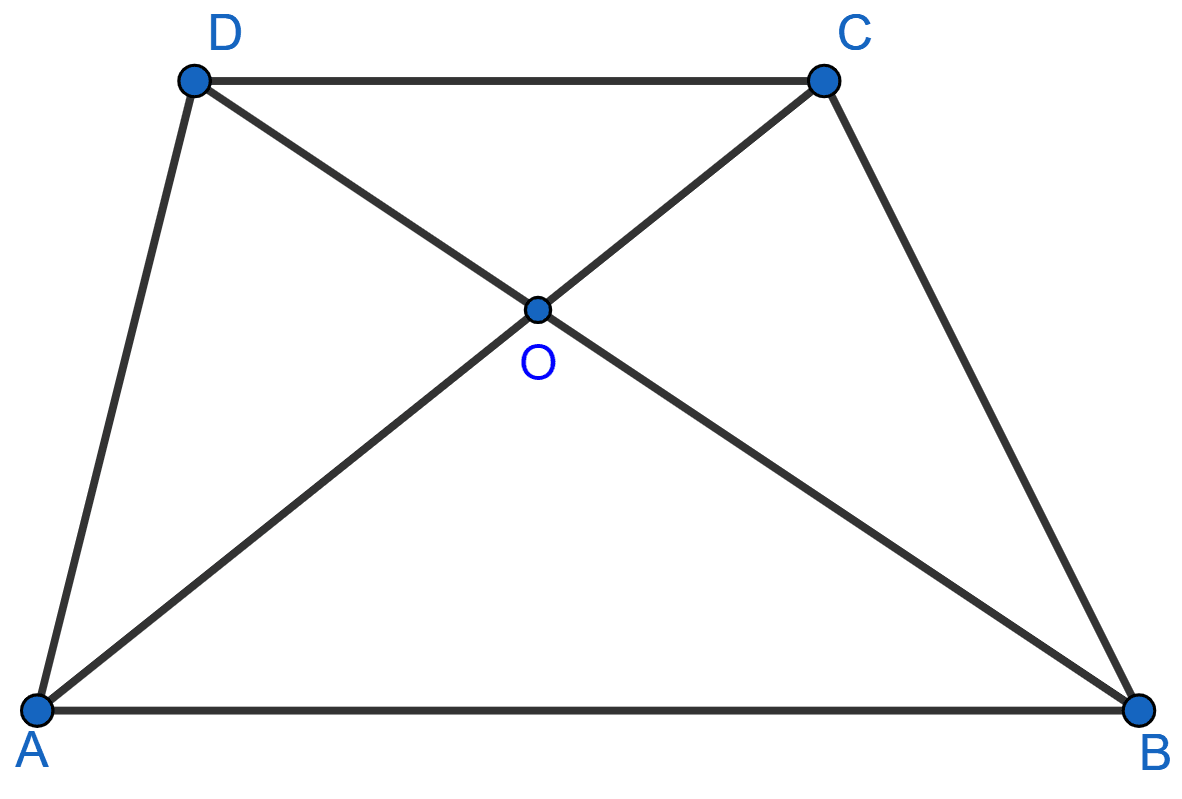
In the adjoining figure, AB || DC and AB ≠ DC. If the diagonals AC and BD of the trapezium ABCD intersect at O, then which of the following statements is not true ?
area of △ABC = area of △ABD
area of △ACD = area of △BCD
area of △OAB = area of △OCD
area of △OAD = area of △OBC
Theorems on Area
2 Likes
Answer
We know that,
Triangles on the same base and between same parallel lines are equal in area.
Hence,
⇒ area of △ABC = area of △ABD …….. (1)
⇒ area of △ACD = area of △BCD
From figure and eqn. (1),
area of (△AOB + △OAD) = area of (△AOB + △OBC)
⇒ area of △OAD = area of △OBC.
Hence, Option 3 is the correct option.
Answered By
1 Like
Related Questions
The mid-points of the sides of a triangle along with any of the vertices as the fourth point make a parallelogram of area equal to
area of △ABC
area of △ABC
area of △ABC
area of △ABC
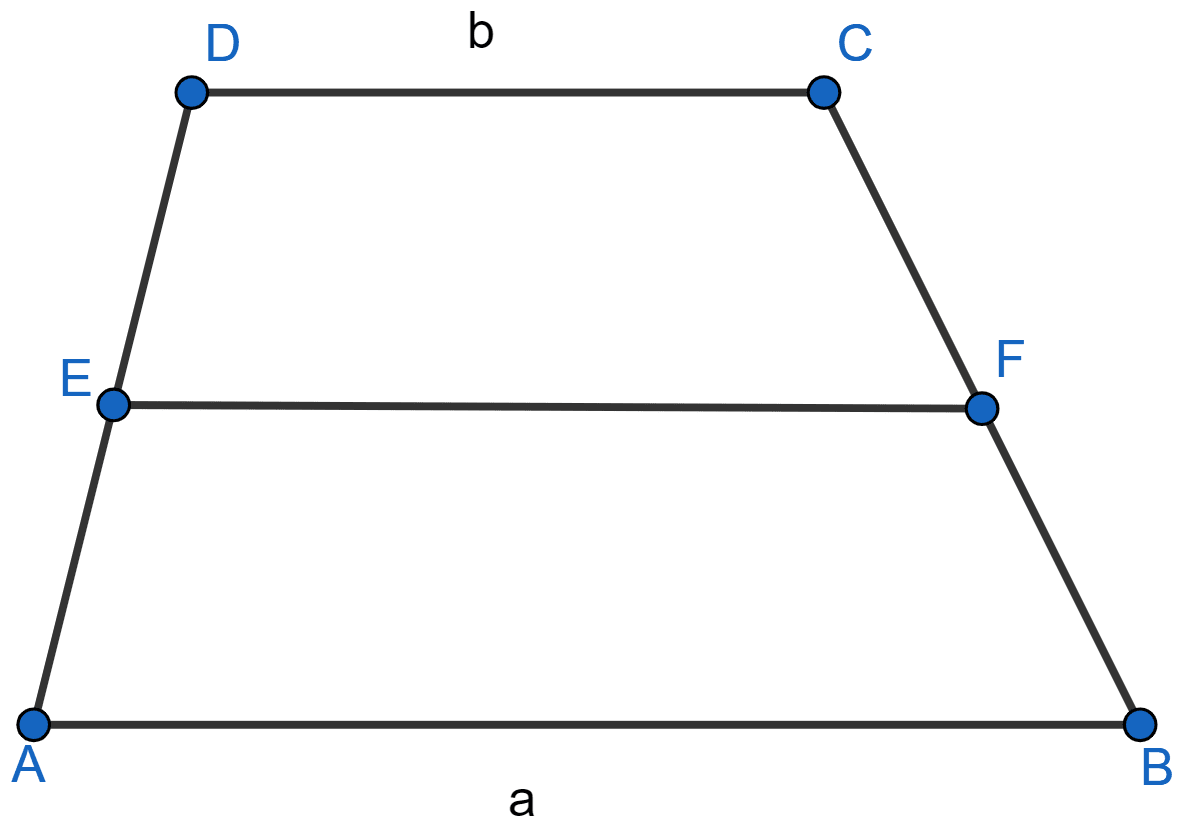
In the adjoining figure, ABCD is a trapezium with parallel sides AB = a cm and DC = b cm. E and F are mid-points of the non-parallel sides. The ratio of area of ABFE and area of EFCD is
a : b
(3a + b) : (a + 3b)
(a + 3b) : (3a + b)
(2a + b) : (3a + b)
In the figure (1) given below, ABCD is a rectangle (not drawn to scale) with side AB = 4 cm and AD = 6 cm. Find
(i) the area of parallelogram DEFC
(ii) area of △EFG.

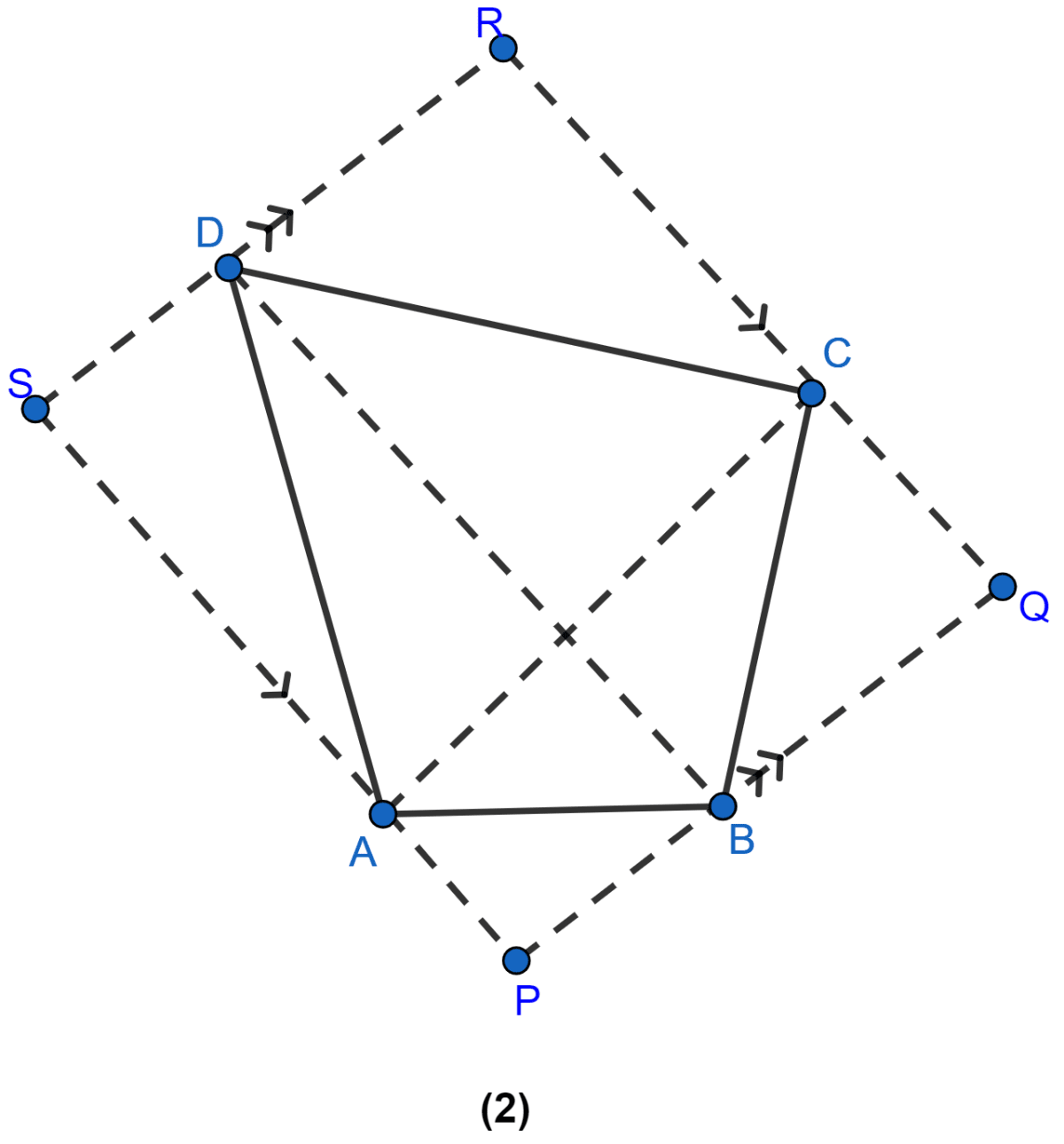
In the figure (2) given below, PQRS is a parallelogram formed by drawing lines parallel to the diagonals of a quadrilateral ABCD through its corners. Prove that area of || gm PQRS = 2 × area of quad. ABCD.