Triangles

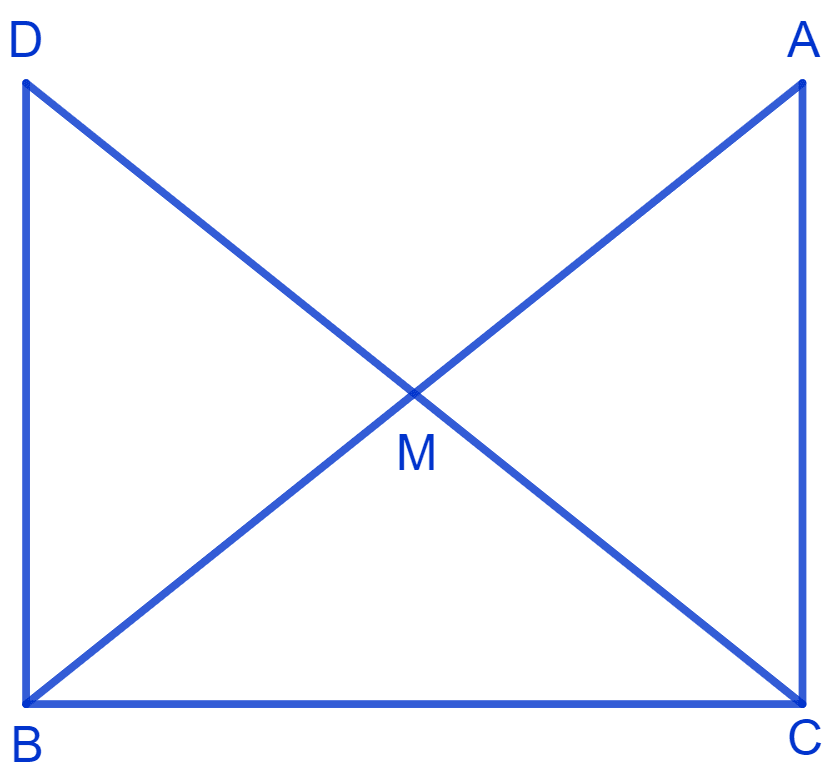
In quadrilateral ACBD, AC = AD and AB bisects ∠A. Show that Δ ABC ≅ Δ ABD. What can you say about BC and BD?

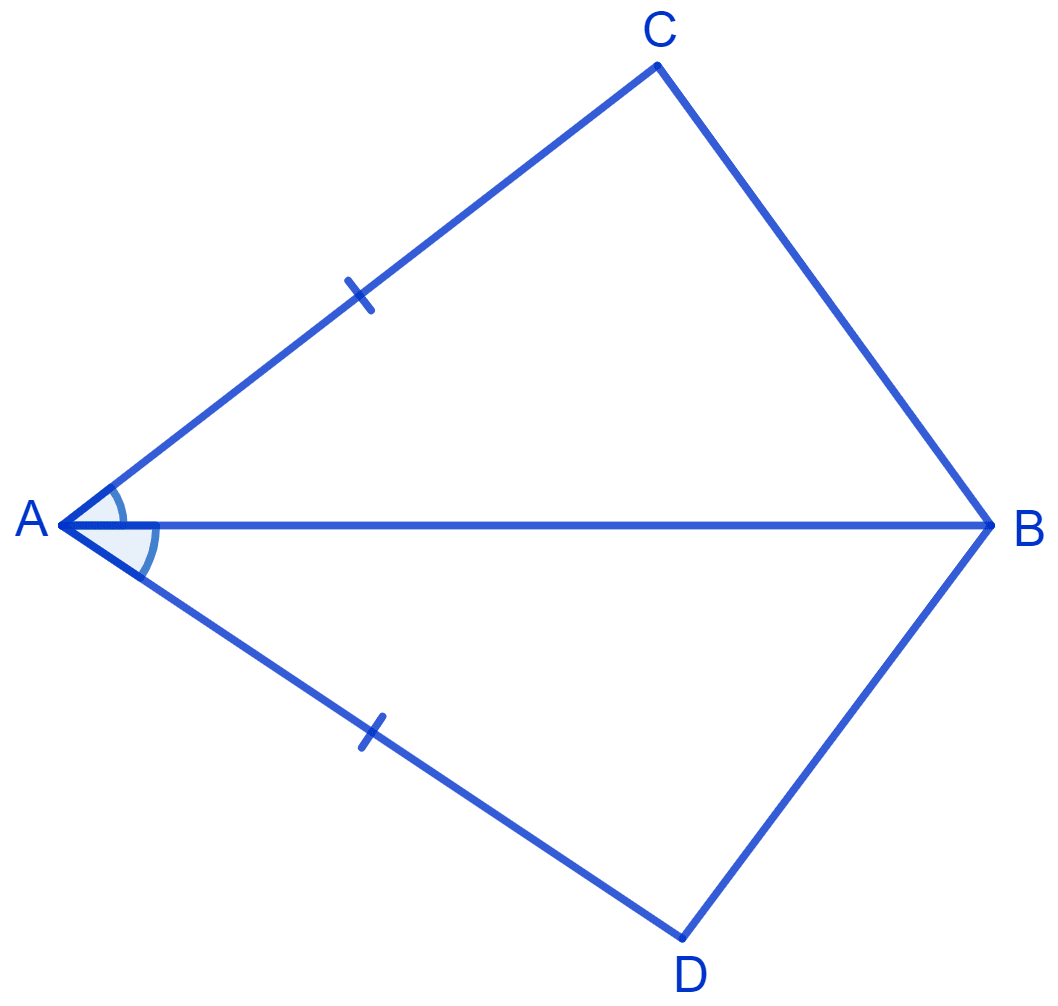
Answer

Given :

AC = AD

AB bisects ∠A i.e, ∠CAB = ∠DAB

In Δ ABC and Δ ABD

⇒ AB = AB (Common side)

⇒ ∠CAB = ∠DAB (Proved above)

⇒ AC = AD (Given)

∴ Δ ABC ≅ Δ ABD (By S.A.S. Congruence rule)

⇒ BC = BD (By C.P.C.T.)

Hence, BC and BD are of equal length.

ABCD is a quadrilateral in which AD = BC and ∠DAB = ∠CBA. Prove that

(i) Δ ABD ≅ Δ BAC

(ii) BD = AC

(iii) ∠ABD = ∠BAC

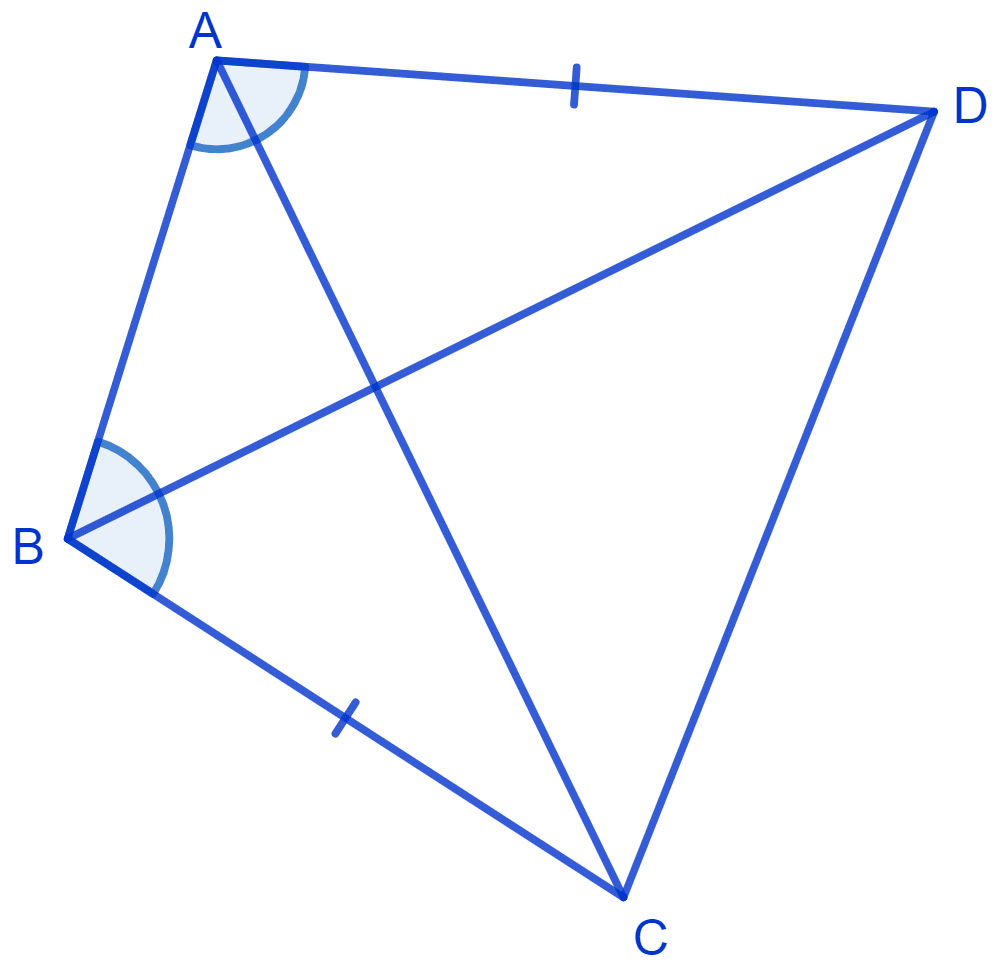
Answer

Given :

AD = BC and ∠DAB = ∠CBA

(i) In △ ABD and △ BAC,

⇒ AD = BC (Given)

⇒ ∠DAB = ∠CBA (Given)

⇒ AB = BA (Common side)

∴ △ ABD ≅ △ BAC (By S.A.S. congruence rule)

Hence, proved that △ ABD ≅ △ BAC.

(ii) As,

△ ABD ≅ △ BAC,

We know that,

Corresponding parts of congruent triangles are equal.

∴ BD = AC (By C.P.C.T.)

Hence, proved that BD = AC.

(iii) As,

△ ABD ≅ △ BAC,

We know that,

Corresponding parts of congruent triangles are equal.

⇒ ∠ABD = ∠BAC (By C.P.C.T.)

Hence, proved that ∠ABD = ∠BAC.

AD and BC are equal, perpendiculars to a line segment AB. Show that CD bisects AB.

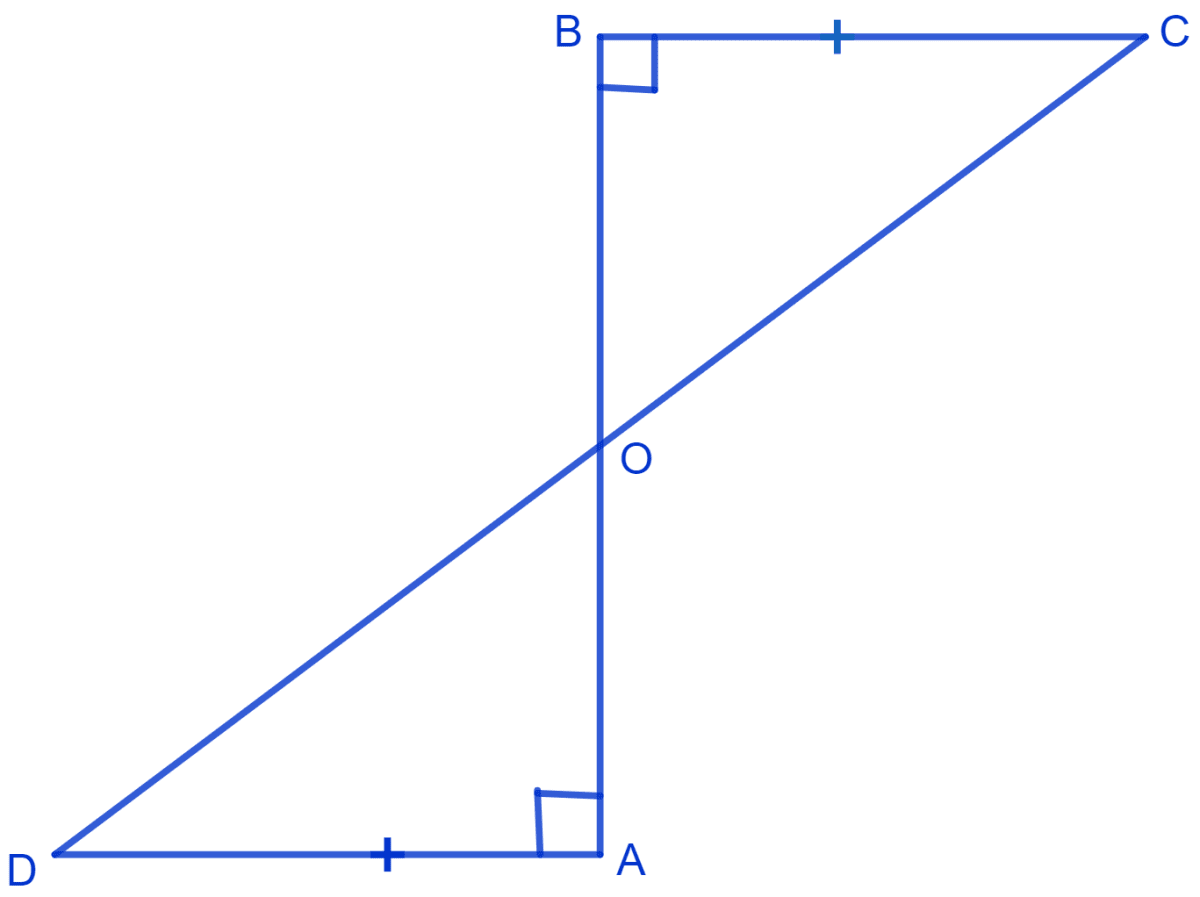
Answer

Given :

⇒ AD = BC

From figure,

⇒ AD ⊥ AB, ∠OAD = 90°

⇒ BC ⊥ AB, ∠OBC = 90°

In △ BOC and △ AOD,

⇒ ∠BOC = ∠AOD (Vertically opposite angles are equal)

⇒ ∠OBC = ∠OAD (Each equal to 90°)

⇒ BC = AD (Given)

∴ △ BOC ≅ △ AOD (By A.A.S. congruence rule)

We know that,

Corresponding parts of congruent triangles are equal.

∴ BO = AO (By C.P.C.T.)

Hence, proved that CD bisects AB and O is the mid-point of AB.

l and m are two parallel lines intersected by another pair of parallel lines p and q. Show that Δ ABC ≅ Δ CDA.

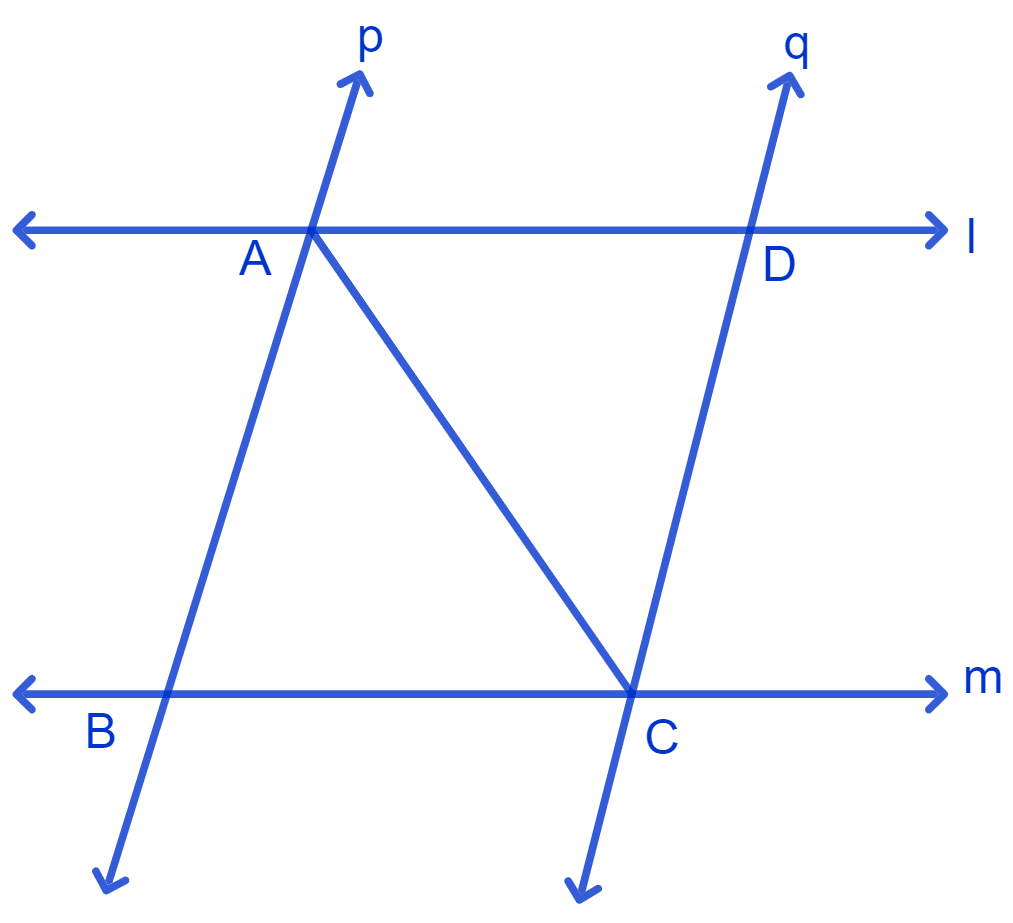
Answer

Given :

l || m and p || q

In Δ ABC and Δ CDA,

⇒ ∠BAC = ∠DCA (Alternate interior angles are equal)

⇒ AC = CA (Common side)

⇒ ∠BCA = ∠DAC (Alternate interior angles are equal)

∴ Δ ABC ≅ Δ CDA (By A.S.A. congruence rule)

Hence, proved that Δ ABC ≅ Δ CDA.

Line l is the bisector of an angle ∠A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠A. Show that:

(i) Δ APB ≅ Δ AQB

(ii) BP = BQ or B is equidistant from the arms of ∠A.

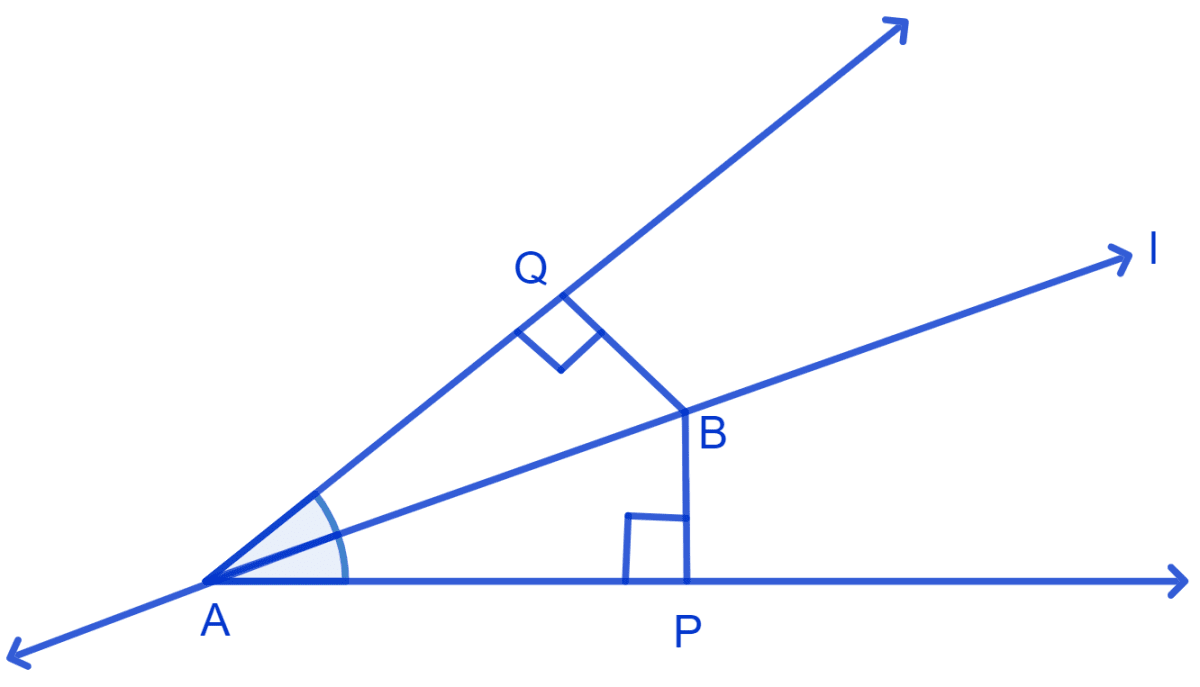
Answer

Given :

l is the bisector of an angle ∠A and BP ⊥ AP and BQ ⊥ AQ

(i) In Δ APB and Δ AQB,

⇒ ∠BAP = ∠BAQ (l is the angle bisector of ∠A)

⇒ ∠APB = ∠AQB (Each equal to 90°)

⇒ AB = AB (Common side)

∴ Δ APB ≅ Δ AQB (By A.A.S. congruence rule)

Hence, proved that Δ APB ≅ Δ AQB.

(ii) As,

Δ APB ≅ Δ AQB

We know that,

Corresponding parts of congruent triangles are equal.

∴ BP = BQ (By C.P.C.T.)

Hence, proved that BP = BQ or point B is equidistant from the arms of ∠A.

In figure, AC = AE, AB = AD and ∠BAD = ∠EAC. Show that BC = DE.

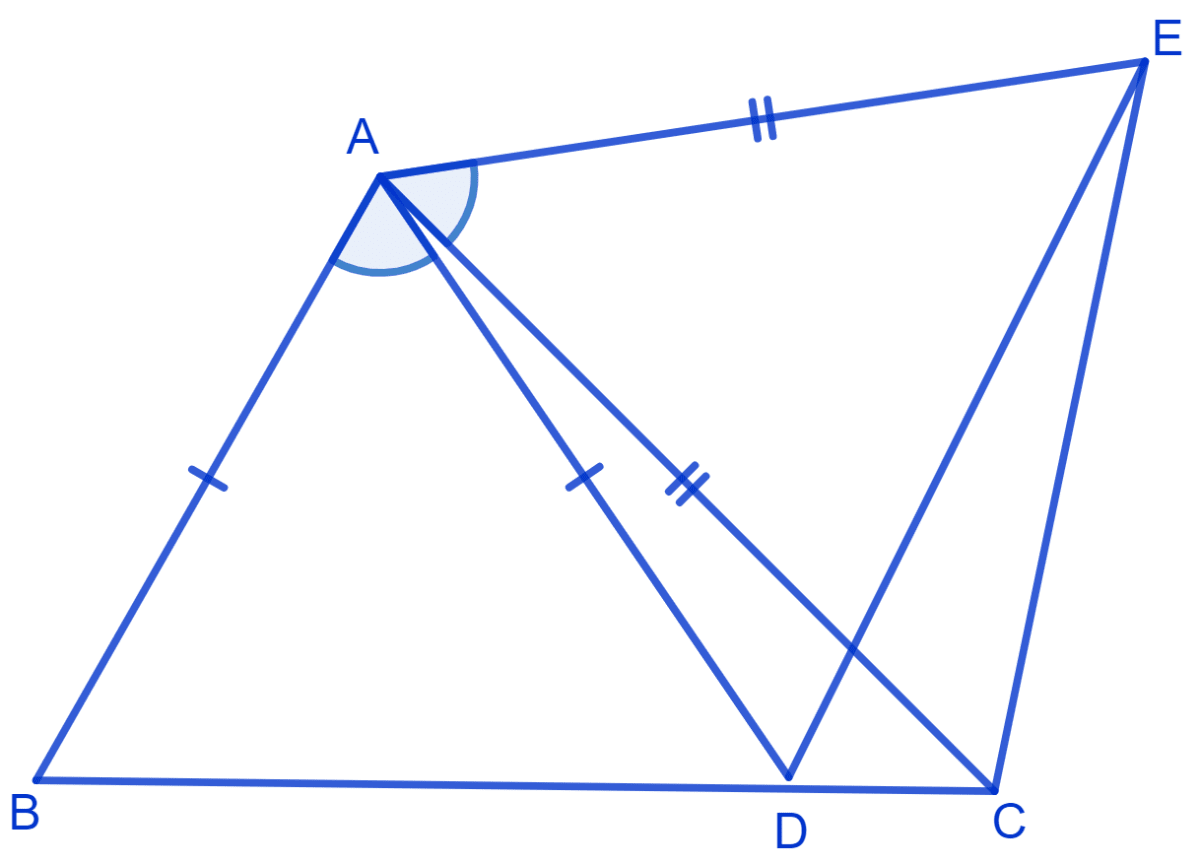
Answer

Given :

AC = AE, AB = AD,

⇒ ∠BAD = ∠EAC.

Adding ∠DAC to both sides of this equation, we get :

⇒ ∠BAD + ∠DAC = ∠EAC + ∠DAC

⇒ ∠BAC = ∠DAE.

In Δ BAC and Δ DAE,

⇒ AB = AD (Given)

⇒ ∠BAC = ∠DAE (Proved above)

⇒ AC = AE (Given)

∴ Δ BAC ≅ Δ DAE (By S.A.S. congruence rule)

We know that,

Corresponding parts of congruent triangles are equal.

∴ BC = DE (By C.P.C.T.)

Hence, proved that BC = DE.

AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB. Show that

(i) Δ DAP ≅ Δ EBP

(ii) AD = BE

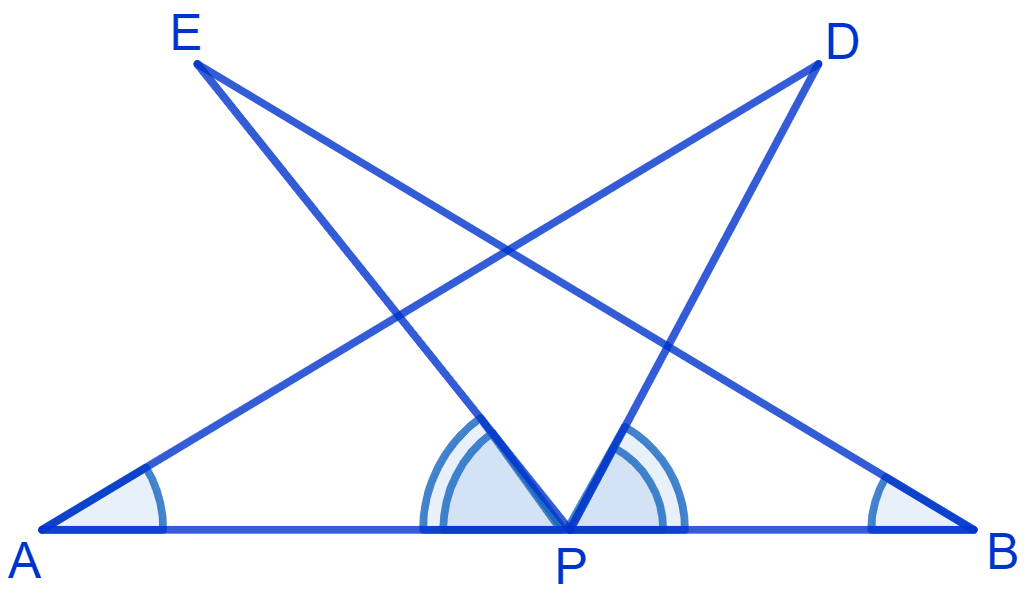
Answer

Given :

P is the mid-point of AB.

∴ AP = BP ......(1)

Given,

⇒ ∠BAD = ∠ABE .....(2)

From figure,

⇒ ∠BAD = ∠PAD and ∠ABE = ∠PBE

Substituting values in equation (2), we get :

⇒ ∠PAD = ∠PBE ........(3)

(i) Given,

⇒ ∠EPA = ∠DPB .........(4)

Adding ∠DPE to both sides of the above equation,

⇒ ∠EPA + ∠DPE = ∠DPB + ∠DPE

∴ ∠DPA = ∠EPB ......(5)

In Δ DAP and Δ EBP,

⇒ ∠PAD = ∠PBE [From (3)]

⇒ AP = BP [From (1)]

⇒ ∠DPA = ∠EPB [From (5)]

∴ Δ DAP ≅ Δ EBP (By A.S.A. congruence rule)

Hence, proved that Δ DAP ≅ Δ EBP.

(ii) As,

Δ DAP ≅ Δ EBP

We know that,

Corresponding parts of congruent triangles are equal.

∴ AD = BE (By C.P.C.T.)

Hence, proved that AD = BE.

In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B. Show that :

(i) Δ AMC ≅ Δ BMD

(ii) ∠DBC is a right angle

(iii) Δ DBC ≅ Δ ACB

(iv) CM = $\dfrac{1}{2}AB$

Answer

(i) In Δ AMC and Δ BMD,

⇒ AM = BM (M is the mid - point of AB)

⇒ ∠AMC = ∠BMD (Vertically opposite angles are equal)

⇒ CM = DM (Given)

∴ Δ AMC ≅ Δ BMD (By S.A.S. congruence rule)

Hence, proved that Δ AMC ≅ Δ BMD.

(ii) Since,

Δ AMC ≅ Δ BMD

∴ ∠ACM = ∠BDM (By C.P.C.T.)

From figure,

∠ACM and ∠BDM are alternate interior angles. Since alternate angles are equal, it can be said that DB || AC.

We know that,

Sum of co-interior angles = 180°.

⇒ ∠DBC + ∠ACB = 180°

⇒ ∠DBC + 90° = 180° [Since, ΔACB is right angled triangle at point C]

⇒ ∠DBC = 180° - 90°

∴ ∠DBC = 90°.

Hence, proved that ∠DBC is a right angle.

(iii) Since,

Δ AMC ≅ Δ BMD

∴ DB = AC (By C.P.C.T.)

In Δ DBC and Δ ACB,

⇒ DB = AC (Proved above)

⇒ ∠DBC = ∠ACB (Both equal to 90°)

⇒ BC = CB (Common)

∴ Δ DBC ≅ Δ ACB (By S.A.S. congruence rule)

Hence, proved that Δ DBC ≅ Δ ACB.

(iv) Since,

Δ DBC ≅ Δ ACB

⇒ AB = DC (By C.P.C.T.)

⇒ $\dfrac{1}{2}AB$ = $\dfrac{1}{2}DC$

It is given that M is the midpoint of DC

⇒ CM = $\dfrac{1}{2}DC$ = $\dfrac{1}{2}AB$

∴ CM = $\dfrac{1}{2}AB$

Hence, proved that CM = $\dfrac{1}{2}AB$.

In an isosceles triangle ABC, with AB = AC, the bisectors of ∠B and ∠C intersect each other at O. Join A to O. Show that :

(i) OB = OC

(ii) AO bisects ∠A

Answer

Given :

AB = AC

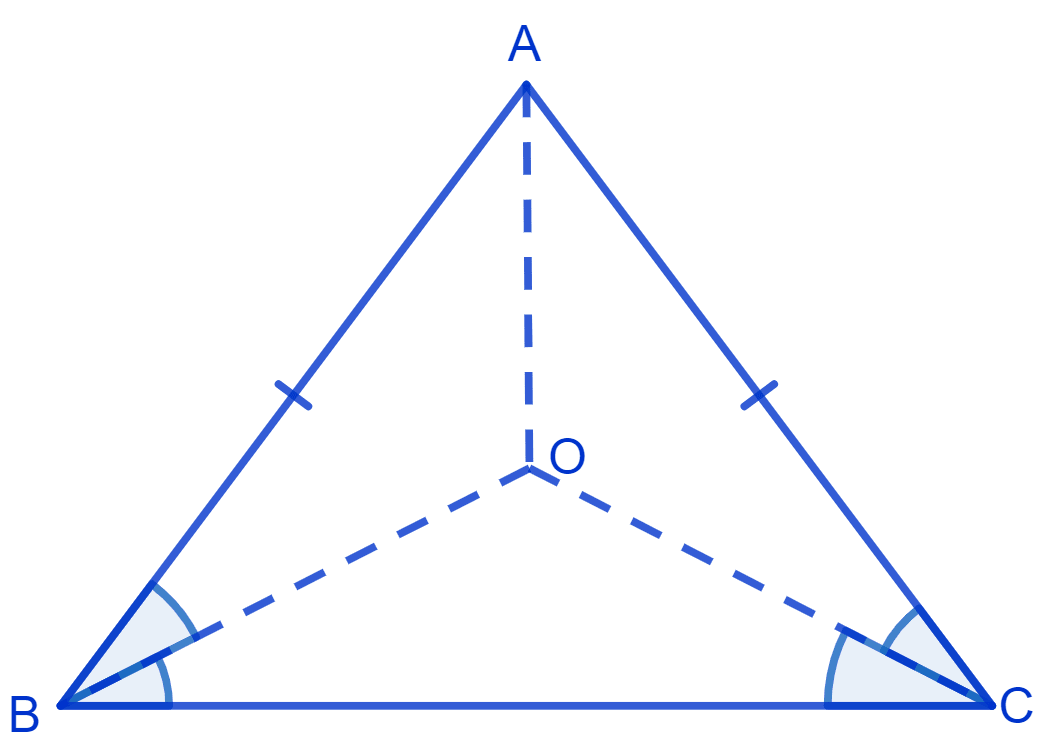
OB is the bisectors of ∠B

⇒ ∠ABO = ∠OBC = $\dfrac{1}{2}∠B$.

OC is the bisectors of ∠C

⇒ ∠ACO = ∠OCB = $\dfrac{1}{2}∠C$.

(i) It is given that in triangle ABC, AB = AC

⇒ ∠ACB = ∠ABC

Dividing both sides of equation by 2, we get :

$\Rightarrow \dfrac{∠ACB}{2} = \dfrac{∠ABC}{2}$

⇒ ∠OCB = ∠OBC

We know that,

Sides opposite to equal angles of a triangle are also equal.

⇒ OB = OC.

Hence, proved that OB = OC.

(ii) In Δ OAB and Δ OAC,

⇒ AO = AO (Common)

⇒ OB = OC (Proved above)

⇒ AB = AC (Proved above)

∴ Δ OAB ≅ Δ OAC (By S.S.S. congruence rule)

We know that,

Corresponding parts of congruent triangles are equal.

⇒ ∠BAO = ∠CAO (By C.P.C.T.)

∴ AO bisects ∠A

Hence, proved that AO bisects ∠A.

In Δ ABC, AD is the perpendicular bisector of BC. Show that Δ ABC is an isosceles triangle in which AB = AC.

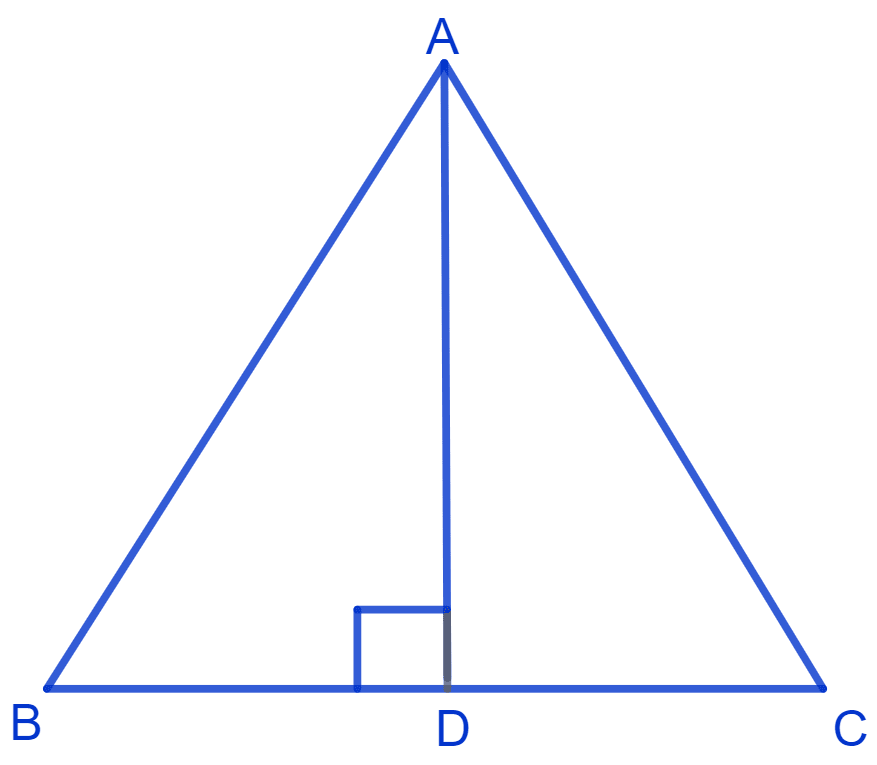
Answer

Given :

AD is the perpendicular bisector of BC.

∴ ∠ADB = ∠ADC = 90° and BD = DC.

In Δ ADC and Δ ADB,

⇒ AD = AD (Common side)

⇒ ∠ADC = ∠ADB (Each equal to 90°)

⇒ CD = BD (AD is the perpendicular bisector of BC)

∴ Δ ADC ≅ Δ ADB (By S.A.S. congruence rule)

We know that,

Corresponding parts of congruent triangles are equal.

∴ AB = AC (By C.P.C.T.)

Hence, proved that ABC is an isosceles triangle in which AB = AC.

ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively see Fig. Show that these altitudes are equal.

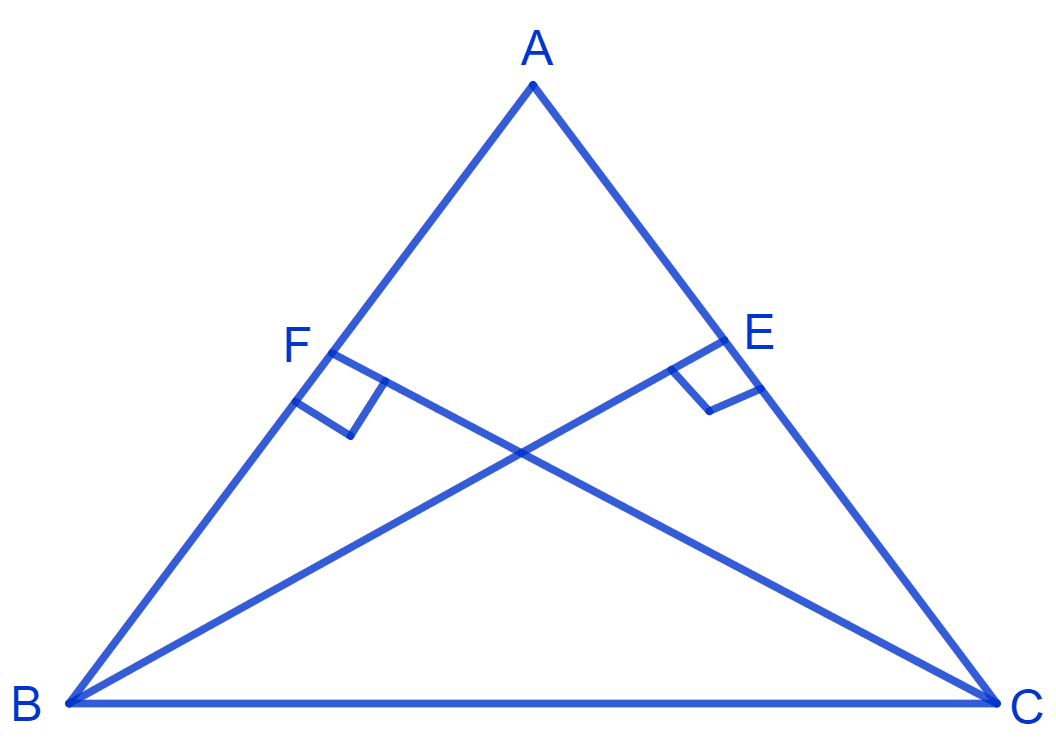
Answer

Given :

Δ ABC is an isosceles triangle with AB and AC as equal sides.

In Δ AEB and Δ AFC,

⇒ ∠AEB = ∠AFC (Each equal to 90° as BE and CF are altitudes)

⇒ ∠A = ∠A (Common angle)

⇒ AB = AC

∴ Δ AEB ≅ Δ AFC (By A.A.S. congruence rule)

We know that,

Corresponding parts of congruent triangles are equal.

∴ BE = CF (By C.P.C.T.)

Hence, proved that BE = CF.

ABC is a triangle in which altitudes BE and CF to sides AC and AB are equal. Show that

(i) Δ ABE ≅ Δ ACF

(ii) AB = AC, i.e., ABC is an isosceles triangle

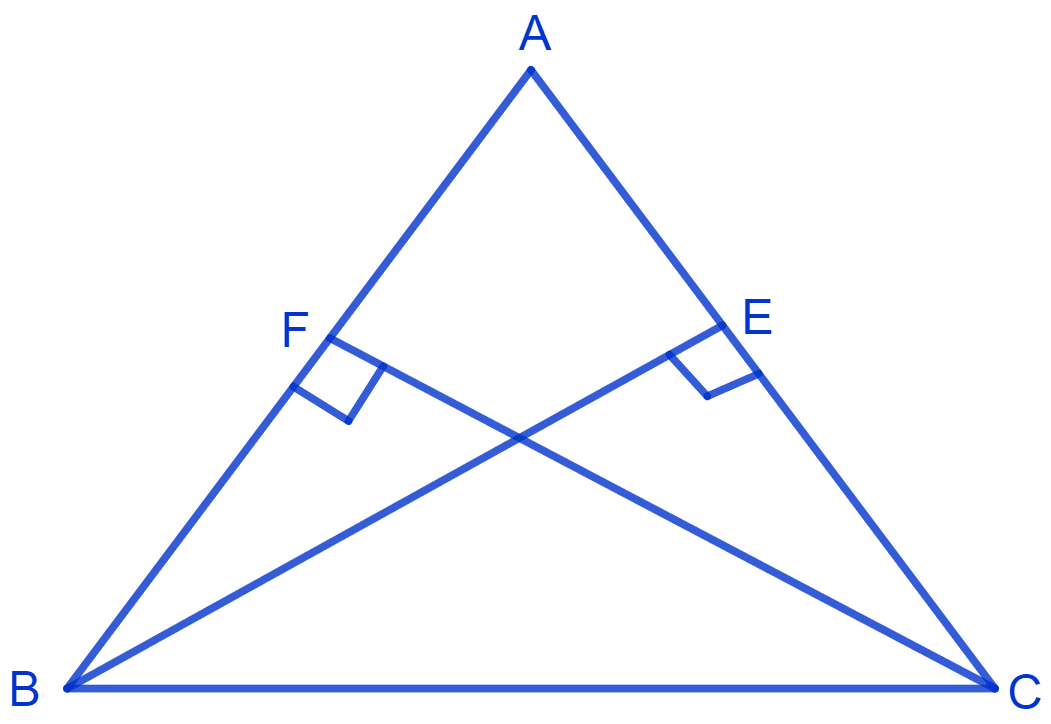
Answer

Given :

BE = CF, where BE and CF are altitudes

So, ∠AEB = 90° and ∠AFC = 90°

(i) In Δ ABE and Δ ACF,

⇒ ∠AEB = ∠AFC (Each 90°)

⇒ ∠A = ∠A (Common angle)

⇒ BE = CF (Given)

∴ Δ ABE ≅ Δ ACF (By A.A.S. congruence rule)

Hence, proved that Δ ABE ≅ Δ ACF.

(ii) As,

Δ ABE ≅ Δ ACF

We know that,

Corresponding parts of congruent triangles are equal.

∴ AB = AC (By C.P.C.T.)

Hence, proved that ABC is an isosceles triangle with AB = AC.

ABC and DBC are two isosceles triangles on the same base BC. Show that ∠ABD = ∠ACD.

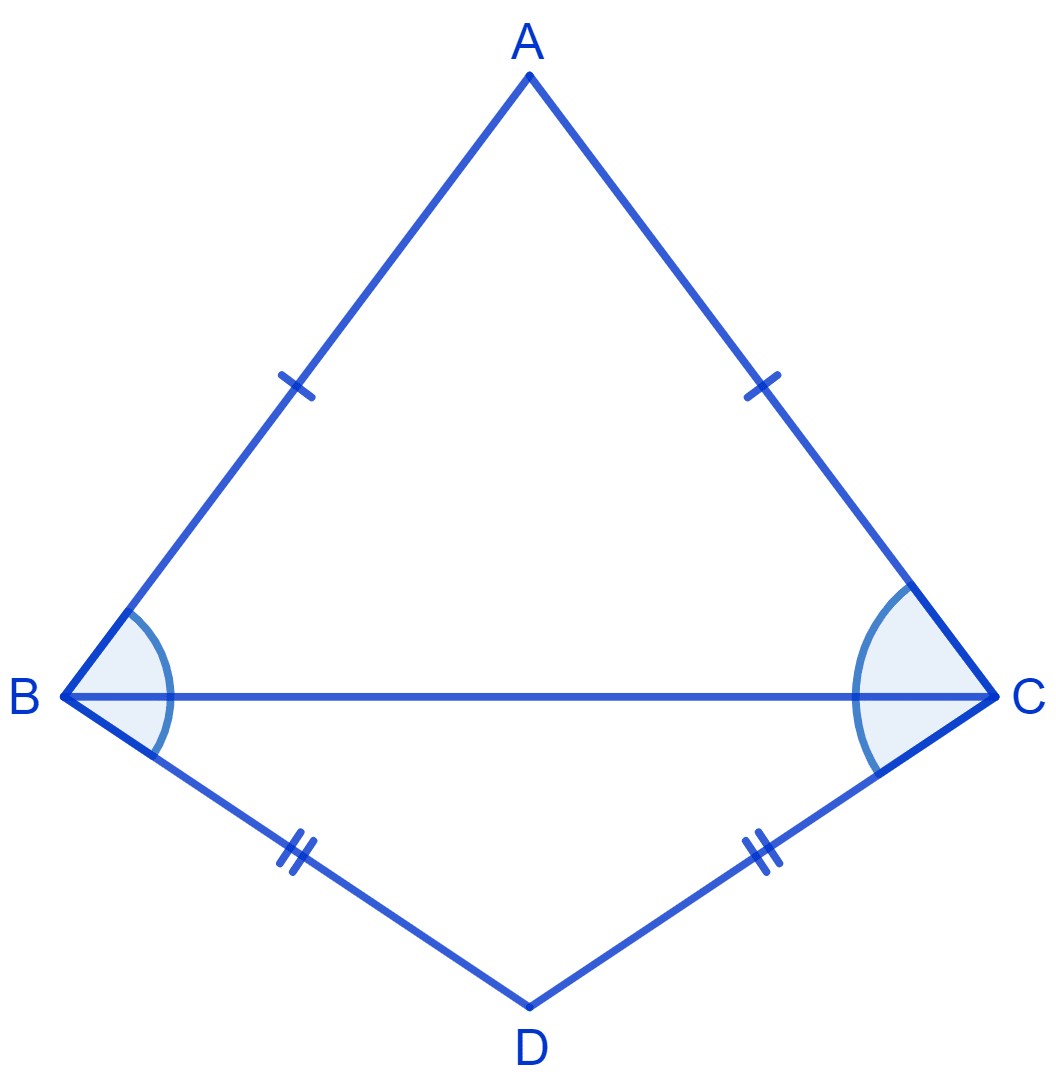
Answer

Given :

ABC and DBC are isosceles triangles.

Join AD.

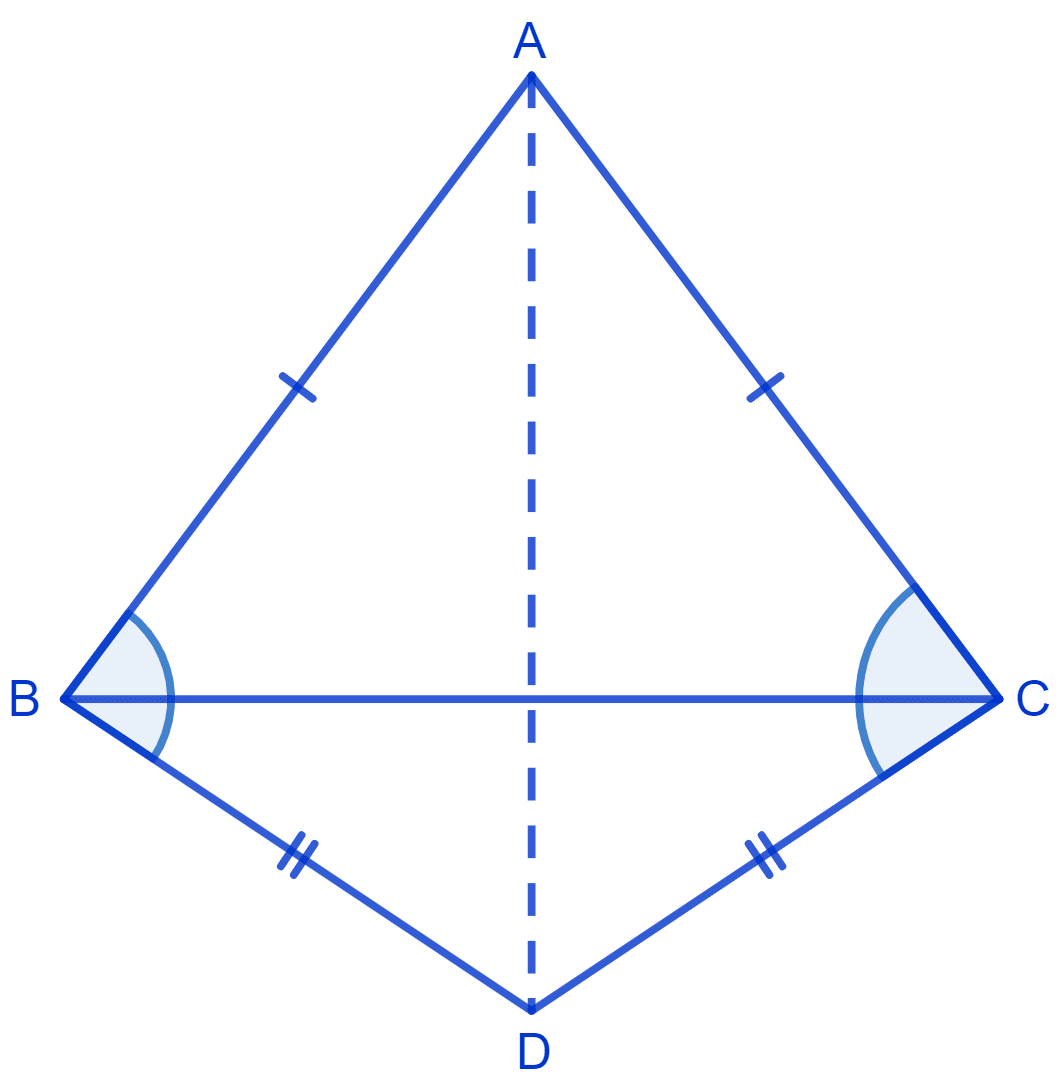
In △ DAB and △ DAC,

⇒ AB = AC (Given)

⇒ BD = CD (Given)

⇒ AD = AD (Common side)

∴ △ ABD ≅ △ ACD (By S.S.S. congruence rule)

We know that,

Corresponding sides of congruent triangles are equal.

∴ ∠ABD = ∠ACD (By C.P.C.T.)

Hence, proved that ∠ABD = ∠ACD.

Δ ABC is an isosceles triangle in which AB = AC. Side BA is produced to D such that AD = AB. Show that ∠BCD is a right angle.

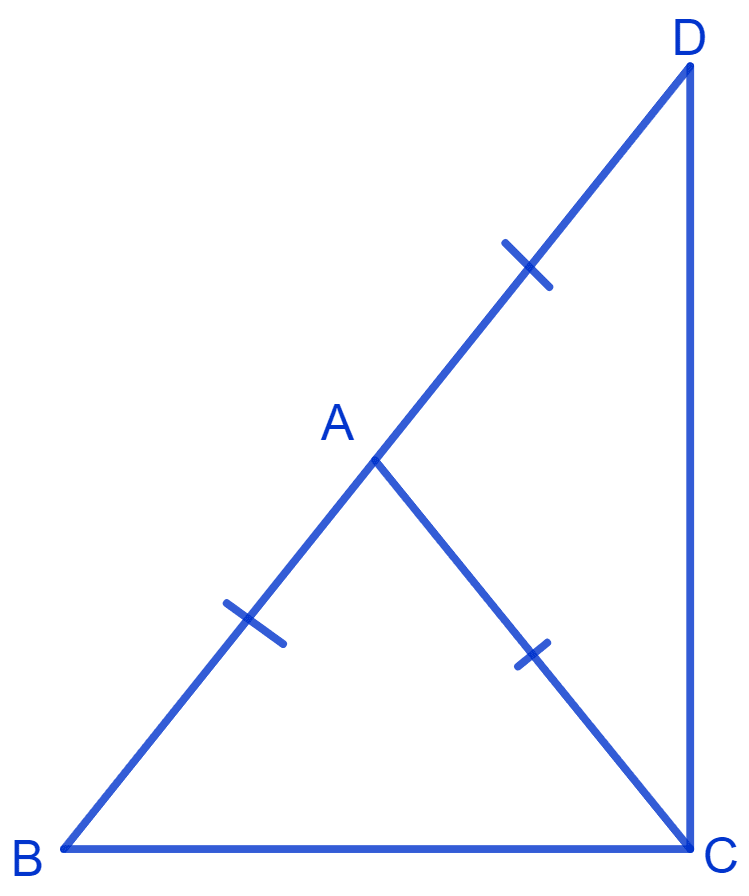
Answer

Given :

⇒ AB = AC .......(1)

⇒ AD = AB ......(2)

From equation (1) and (2), we get :

⇒ AB = AC = AD

In an isosceles triangle ABC,

⇒ AB = AC

We know that,

Angles opposite to equal sides of a triangle are equal.

∴ ∠ACB = ∠ABC = x (let)

In Δ ACD,

⇒ AC = AD

We know that,

Angles opposite to equal sides of a triangle are equal.

∴ ∠ADC = ∠ACD = y (let)

From figure,

⇒ ∠BCD = ∠ACB + ∠ACD

⇒ ∠BCD = x + y .....(3)

In Δ BCD,

⇒ ∠ABC + ∠BCD + ∠ADC = 180° (Angle sum property of a triangle)

⇒ x + (x + y) + y = 180° [From equation (1), (2) and (3)]

⇒ 2(x + y) = 180°

Substituting value of (x + y) from equation (3) in above equation :

⇒ 2(∠BCD) = 180°

⇒ ∠BCD = $\dfrac{180°}{2}$

⇒ ∠BCD = 90°

Hence, proved that ∠BCD is a right angle.

ABC is a right angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.

Answer

Given :

AB = AC and ∠A = 90°

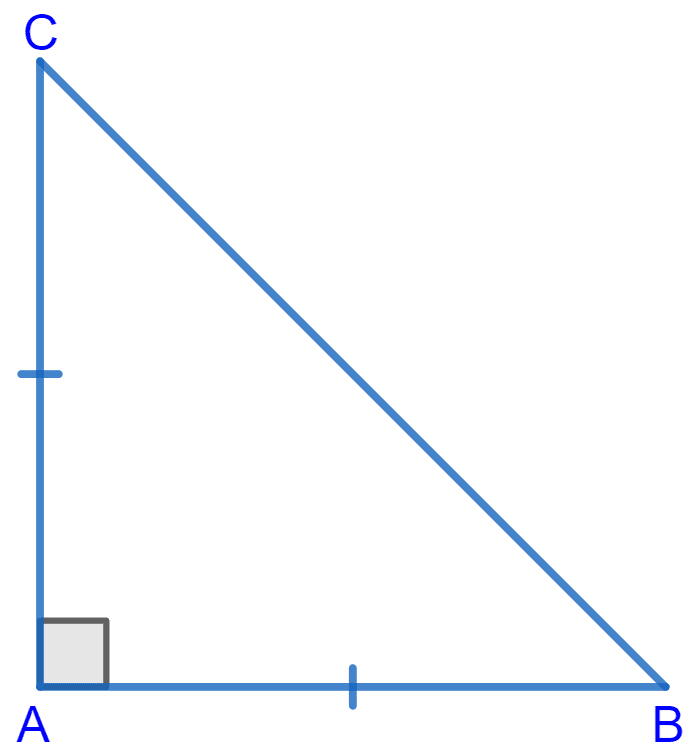
We know that,

Angles opposite to equal sides are also equal.

∠C = ∠B = x (let)

In Δ ABC,

⇒ ∠A + ∠B + ∠C = 180° (Angle sum property of a triangle)

⇒ 90° + ∠B + ∠C = 180°

⇒ 90° + x + x = 180° (From(1))

⇒ 2x = 180° - 90°

⇒ 2x = 90°

⇒ x = $\dfrac{90°}{2}$

⇒ x = 45°.

∴ ∠B = ∠C = 45°

Hence, ∠B = 45° and ∠C = 45°.

Show that the angles of an equilateral triangle are 60° each.

Answer

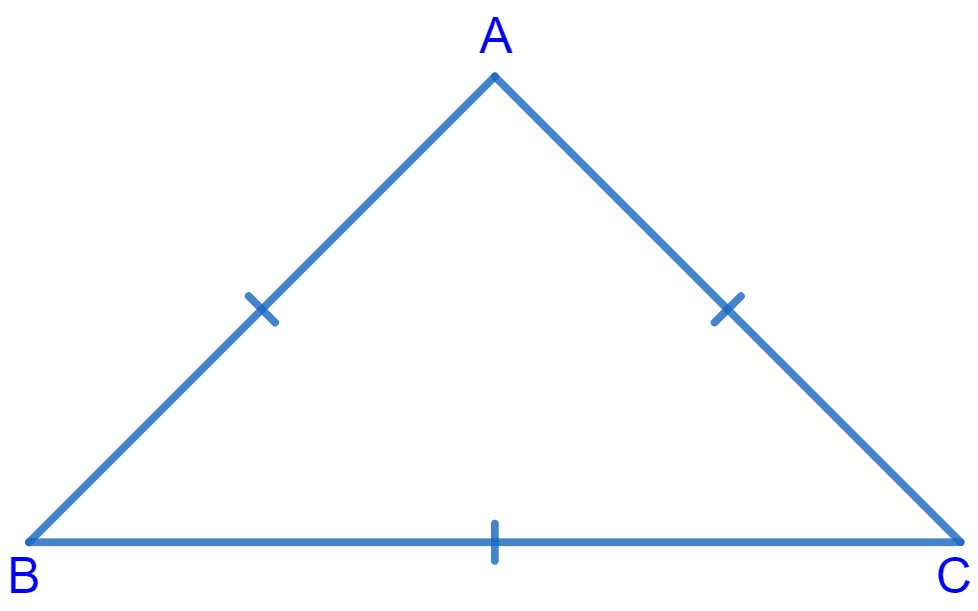
Construct a triangle ABC with AB = BC = AC.

In △ ABC,

AB = BC = AC

We know that,

Angles opposite to equal sides of a triangle are equal.

∴ ∠C = ∠A = ∠B = x (let)

In △ ABC,

⇒ ∠A + ∠B + ∠C = 180° (Angle sum property of a triangle)

⇒ x + x + x = 180°

⇒ 3x = 180°

⇒ x = $\dfrac{180°}{3}$

⇒ x = 60°.

∴ ∠A = ∠B = ∠C = 60°.

Hence, proved that the angles of an equilateral triangle are 60° each.

Δ ABC and Δ DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC. If AD is extended to intersect BC at P, show that

(i) Δ ABD ≅ Δ ACD

(ii) Δ ABP ≅ Δ ACP

(iii) AP bisects ∠A as well as ∠D

(iv) AP is the perpendicular bisector of BC

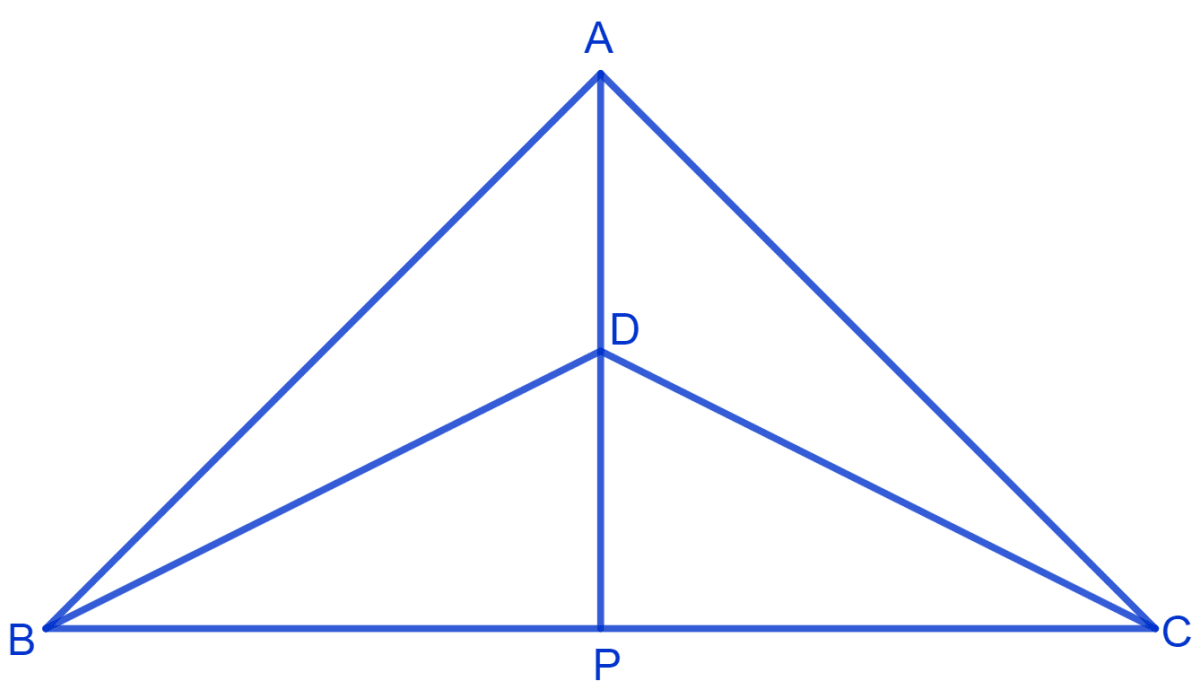
Answer

Given :

Δ ABC and Δ DBC are isosceles triangles on the same base BC.

∴ AB = AC and DB = DC

(i) In Δ ABD and Δ ACD,

⇒ AB = AC (Equal sides of isosceles Δ ABC)

⇒ BD = CD (Equal sides of isosceles Δ DBC)

⇒ AD = AD (Common)

∴ Δ ABD ≅ Δ ACD (By S.S.S. congruence rule)

Hence, proved that Δ ABD ≅ Δ ACD.

(ii) Since,

Δ ABD ≅ Δ ACD.

We know that,

Corresponding parts of congruent triangles are equal.

⇒ ∠BAD = ∠CAD ........(1)

From figure,

⇒ ∠BAD = ∠BAP and ∠CAD = ∠CAP

Substituting above value in equation (1), we get :

⇒ ∠BAP = ∠CAP ..........(2)

In Δ ABP and Δ ACP,

⇒ AB = AC (Equal sides of isosceles Δ ABC)

⇒ ∠BAP = ∠CAP [From equation (2)]

⇒ AP = AP (Common)

∴ Δ ABP ≅ Δ ACP (By S.A.S. congruence rule)

Hence, proved that Δ ABP ≅ Δ ACP.

(iii) Since,

Δ ABD ≅ Δ ACD

∴ ∠ADB = ∠ADC (By C.P.C.T.) ..........(3)

∴ ∠BAP = ∠CAP [From equation (2)]

∴ AP is the angle bisector of ∠A.

From equation (3),

⇒ ∠ADB = ∠ADC

⇒ 180° - ∠ADB = 180° - ∠ADC

⇒ ∠BDP = ∠CDP ......(4)

∴ AP is the bisector of ∠D

Hence, proved that AP bisects ∠A as well as ∠D.

(iv) In Δ BDP and Δ CDP,

⇒ DP = DP (Common side)

⇒ ∠BDP = ∠CDP [From equation (4)]

⇒ DB = DC (Equal sides of isosceles Δ DBC)

∴ Δ BDP ≅ Δ CDP (By S.A.S. congruence rule)

∴ ∠BPD = ∠CPD (By C.P.C.T.) .......(5)

From figure,

⇒ ∠BPD + ∠CPD = 180° (Linear pair)

⇒ ∠BPD + ∠BPD = 180° [From Equation (5)]

⇒ 2∠BPD = 180°

⇒ ∠BPD = 90° .......(6)

We know that,

⇒ BP = CP [Proved above]

Hence, proved that AP is the perpendicular bisector of BC.

AD is an altitude of an isosceles triangle ABC in which AB = AC. Show that

(i) AD bisects BC

(ii) AD bisects ∠A

Answer

Given :

Δ ABC is an isosceles triangle and AB = AC.

AD is altitude

∴ ∠ADB = ∠ADC = 90°.

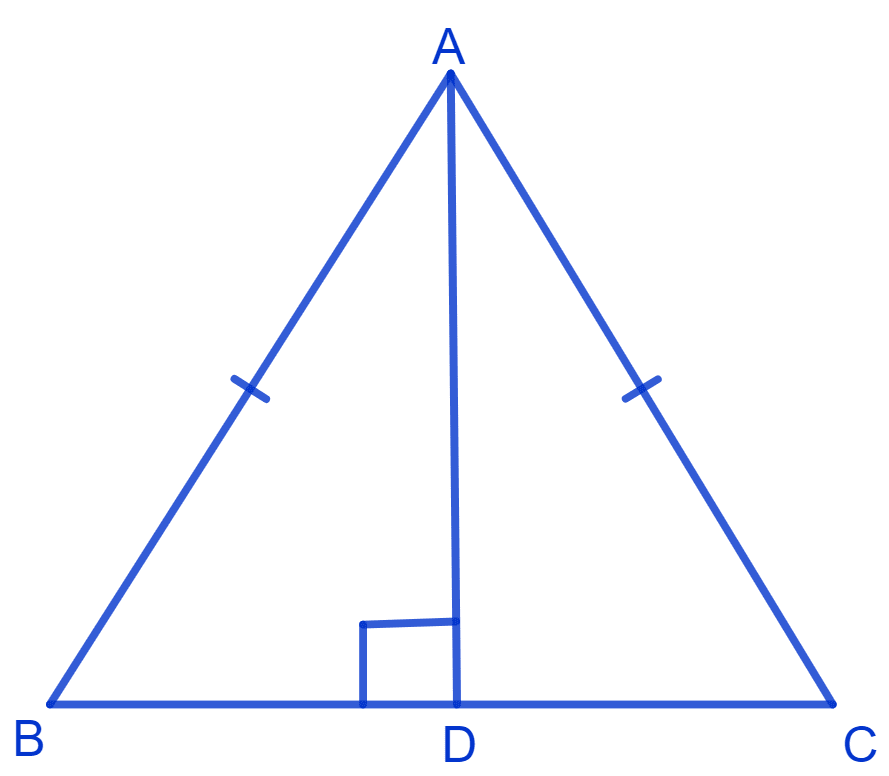
(i) In Δ BAD and Δ CAD,

⇒ ∠ADB = ∠ADC (Each equal to 90° as AD is altitude)

⇒ AB = AC (Given)

⇒ AD = AD (Common)

∴ Δ BAD ≅ Δ CAD (By R.H.S. Congruence rule)

We know that,

Corresponding parts of congruent triangles are equal.

∴ BD = CD (By C.P.C.T.)

Hence, proved that AD bisects BC.

(ii) Since, Δ BAD ≅ Δ CAD

∴ ∠BAD = ∠CAD (By C.P.C.T.)

Hence, proved that AD bisects ∠A.

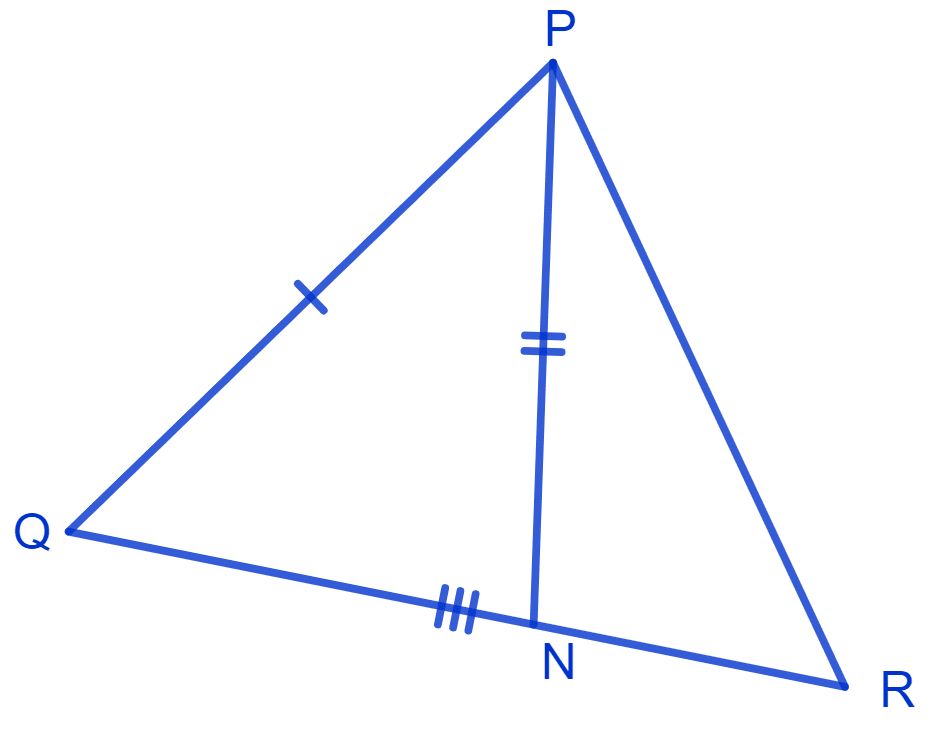
Two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of Δ PQR. Show that :

(i) Δ ABM ≅ Δ PQN

(ii) Δ ABC ≅ Δ PQR

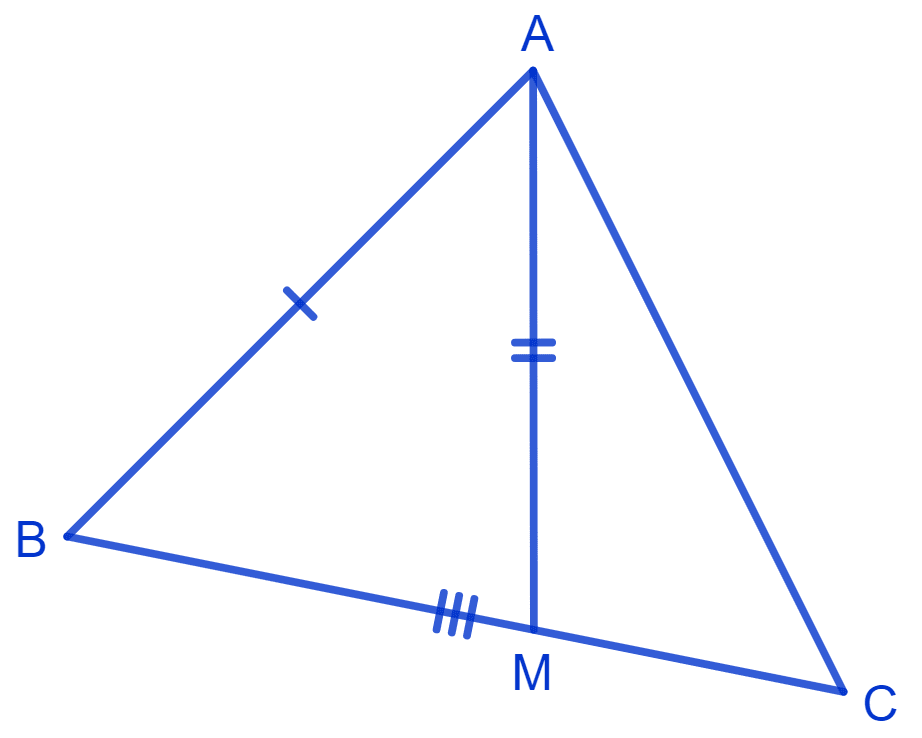
Answer

Given :

AB = PQ, BC = QR = x (let) and AM = PN.

(i) Given,

AM is the median of △ ABC

∴ BM = CM = $\dfrac{1}{2}BC = \dfrac{x}{2}$ .....(1)

Also, PN is the median of △ PQR

∴ QN = RN = $\dfrac{1}{2}QR = \dfrac{x}{2}$ ......(2)

From equation (1) and (2), we get :

BM = QN ..........(3)

Now, in △ ABM and △ PQN we have,

⇒ AB = PQ (Given)

⇒ BM = QN [From equation (3)]

⇒ AM = PN (Given)

∴ △ ABM ≅ △ PQN (By S.S.S. congruence rule)

Hence, proved that △ ABM ≅ △ PQN.

(ii) Since,

△ ABM ≅ △ PQN

We know that,

Corresponding parts of the congruent triangle are equal.

∠B = ∠Q (By C.P.C.T.) ...........(4)

Now, In △ ABC and △ PQR we have

⇒ AB = PQ (Given)

⇒ ∠B = ∠Q [From equation (4)]

⇒ BC = QR (Given)

∴ △ ABC ≅ △ PQR (By S.A.S. congruence rule)

Hence, proved that △ ABC ≅ △ PQR.

BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.

Answer

Triangle ABC with BE and CF as equal altitudes is shown in the figure below:

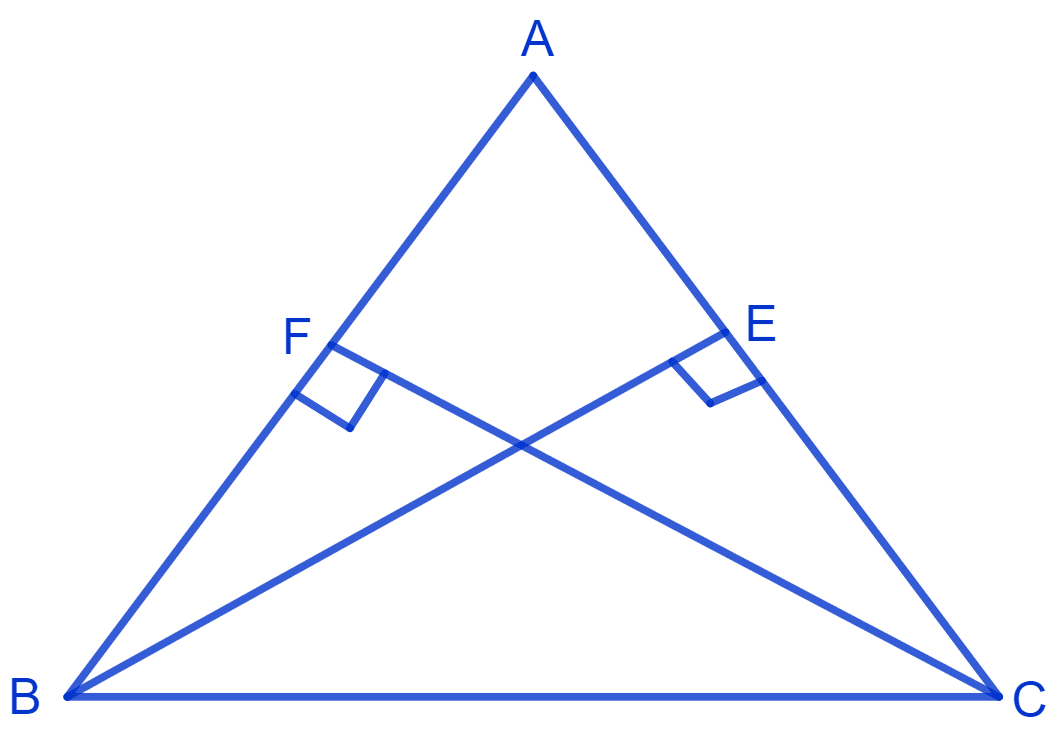
Given :

BE is a altitude.

∴ ∠AEB = ∠CEB = 90°

CF is a altitude.

∴ ∠AFC = ∠BFC = 90°

Also, BE = CF.

In Δ BEC and Δ CFB,

⇒ ∠BEC = ∠CFB (Each equal to 90°)

⇒ BC = CB (Common)

⇒ BE = CF (Given)

⇒ Δ BEC ≅ Δ CFB (By R.H.S. congruence rule)

We know that,

Corresponding parts of congruent triangle are equal.

⇒ ∠BCE = ∠CBF (By C.P.C.T.)

As,

Sides opposite to equal angles of a triangle are equal.

∴ AB = AC.

Hence, proved that Δ ABC is an isosceles triangle.

ABC is an isosceles triangle with AB = AC. Draw AP ⊥ BC to show that ∠B = ∠C.

Answer

Given :

Δ ABC is an isosceles with AB = AC.

Draw AP ⊥ BC,

∴ ∠APB = ∠APC = 90°

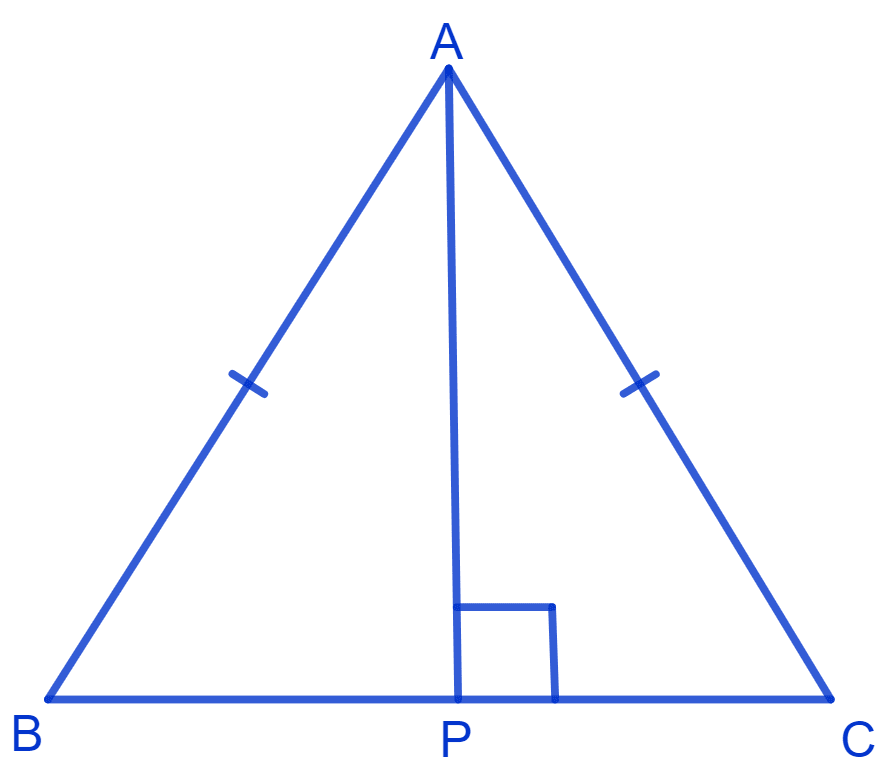
In Δ APB and Δ APC,

⇒ ∠APB = ∠APC (Each equal to 90°)

⇒ AB = AC (Since ABC is an isosceles triangle)

⇒ AP = AP (Common)

∴ Δ APB ≅ Δ APC (By R.H.S. congruence rule)

We know that,

Corresponding parts of congruent triangle are equal.

∴ ∠B = ∠C (By C.P.C.T.)

Hence, proved that ∠B = ∠C.