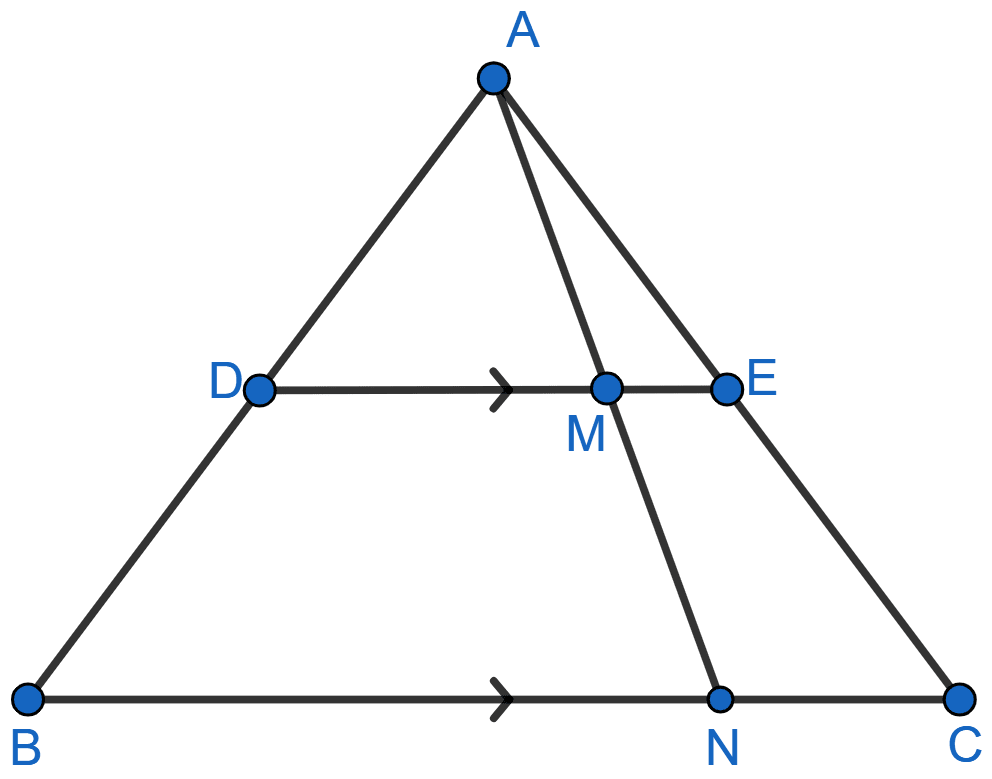
In the given figure, DE || BC, AE = 15 cm, EC = 9 cm, NC = 6 cm and BN = 24 cm.

(i) Write all possible pairs of similar triangles.

(ii) Find the lengths of ME and DM.

(i) In ΔAME and ΔANC,

⇒ ∠AME = ∠ANC [Since DE || BC so, ME || NC and AN is transversal]

⇒ ∠MAE = ∠NAC [Common angle]

∴ ∆AME ~ ∆ANC [By AA]

In ΔADM and ΔABN,

⇒ ∠ADM = ∠ABN [Since DE || BC so, DM || BN and AB is transversal]

⇒ ∠DAM = ∠BAN [Common angle]

∴ ∆ADM ~ ∆ABN [By AA]

In ΔADE and ΔABC,

⇒ ∠ADE = ∠ABC [Since DE || BC and AB is transversal]

⇒ ∠AED = ∠ACB [Since DE || BC and AC is transversal]

∴ ∆ADE ~ ∆ABC [By AA]

Hence, ∆ADM ~ ∆ABN, ∆AME ~ ∆ANC and ∆ADE ~ ∆ABC.

(ii) Since, ∆AME ~ ∆ANC

We know that,

Corresponding sides of similar triangles are proportional.

$\Rightarrow \dfrac{ME}{NC} = \dfrac{AE}{AC} \\[1em] \Rightarrow \dfrac{ME}{6} = \dfrac{15}{15 + 9} \\[1em] \Rightarrow ME = \dfrac{15}{24} \times 6 \\[1em] \Rightarrow ME = 3.75 \text{ cm}.$

Since, ∆ADE ~ ∆ABC [Proved above]

We know that,

Corresponding sides of similar triangles are proportional.

$\dfrac{AD}{AB} = \dfrac{AE}{AC} = \dfrac{15}{24}$ ……… (1)

Also, ∆ADM ~ ∆ABN [Proved above]

$\therefore \dfrac{DM}{BN} = \dfrac{AD}{AB} = \dfrac{15}{24} ……..[From (1)] \\[1em] \therefore \dfrac{DM}{BN} = \dfrac{15}{24} \\[1em] DM = \dfrac{15}{24} \times BN = \dfrac{15}{24} \times 24 = 15 \text{ cm}.$

Hence, ME = 3.75 cm and DM = 15 cm.