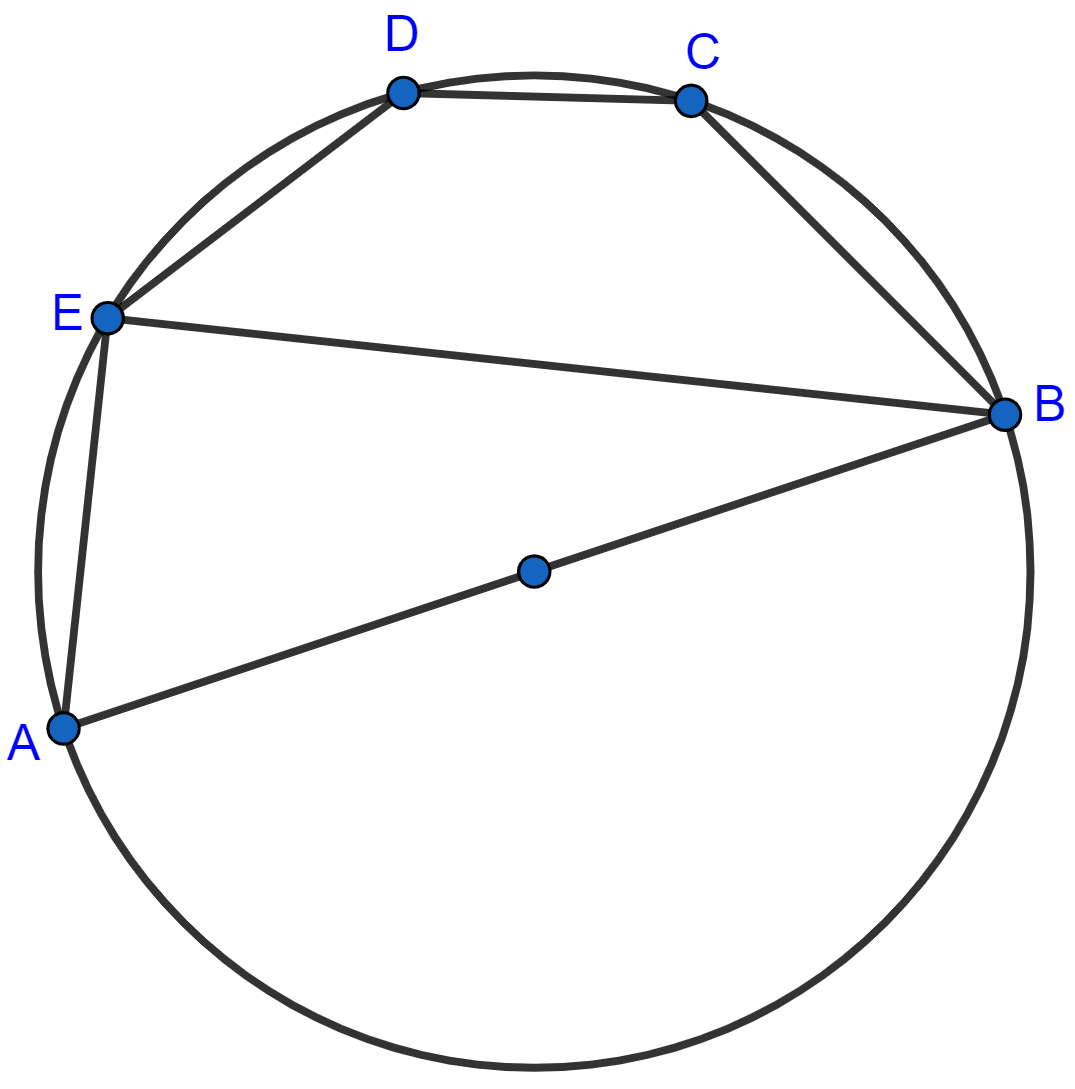
In the given figure, AB is a diameter of the circle. Chord ED is parallel to AB and ∠EAB = 63°. Calculate :

(i) ∠EBA,

(ii) ∠BCD.

(i) We know that,

Angle in semi-circle is a right angle.

∴ ∠AEB = 90°.

In △AEB,

⇒ ∠AEB + ∠EBA + ∠EAB = 180°

⇒ 90° + ∠EBA + 63° = 180°

⇒ 153° + ∠EBA = 180°

⇒ ∠EBA = 180° - 153° = 27°.

Hence, ∠EBA = 27°.

(ii) As, AB || ED

∴ ∠DEB = ∠EBA = 27° [Alternate angles]

BCDE is a cyclic quadrilateral.

∴ ∠DEB + ∠BCD = 180° [Sum of opposite angles in a cyclic quadrilateral = 180°.]

⇒ 27° + ∠BCD = 180°

⇒ ∠BCD = 180° - 27° = 153°.

Hence, ∠BCD = 153°.