Mathematics
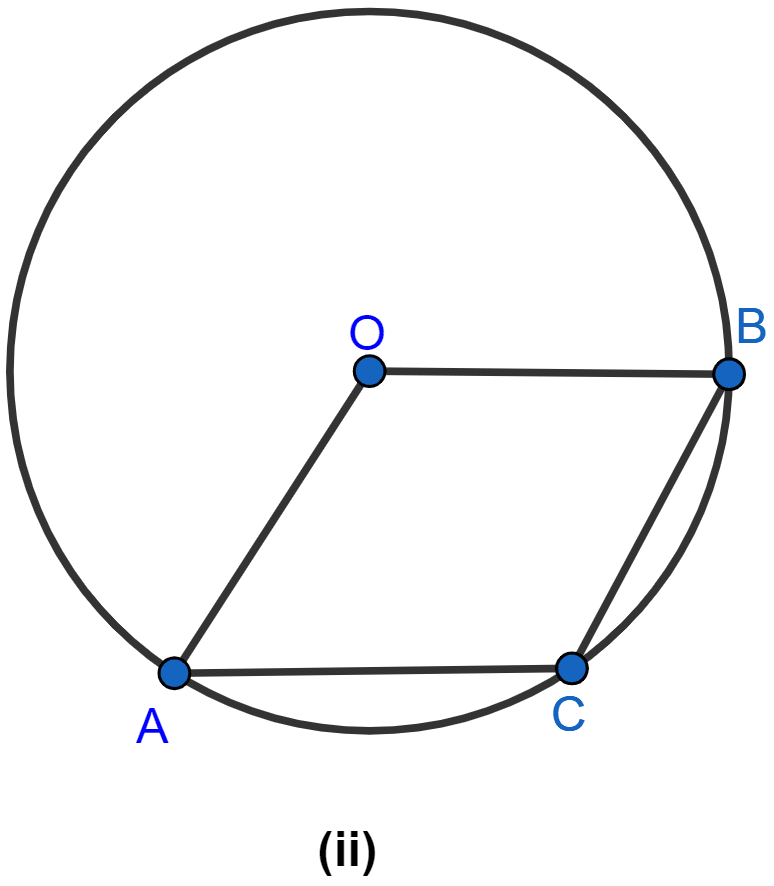
In the figure (ii) given below, C is a point on the minor arc AB of the circle with centre O. Given ∠ACB = p°, ∠AOB = q°, express q in terms of p. Calculate p if OACB is a parallelogram.
Circles
56 Likes
Answer
From figure,
⇒ ∠AOB + Reflex ∠AOB = 360°
⇒ q° + Reflex ∠AOB = 360°
⇒ Reflex ∠AOB = 360° - q°.
Arc AB subtends reflex ∠AOB at center and ∠ACB at the point C on the circle.
∴ Reflex ∠AOB = 2∠ACB
⇒ 360° - q° = 2 × p°
⇒ 360° - q° = 2p°
⇒ 2p° + q° = 360°
⇒ q° = 360° - 2p°
⇒ q° = 2(180° - p°)
⇒ q = 2(180 - p).
Given, OABC is a parallelogram, then
Opposite angles are equal.
∴ ∠AOB = ∠ACB
⇒ p° = q°
⇒ p° = 360° - 2p°
⇒ 3p° = 360°
⇒ p° = 120°.
Hence, q = 2(180 - p) and the value of p = 120.
Answered By
31 Likes
Related Questions
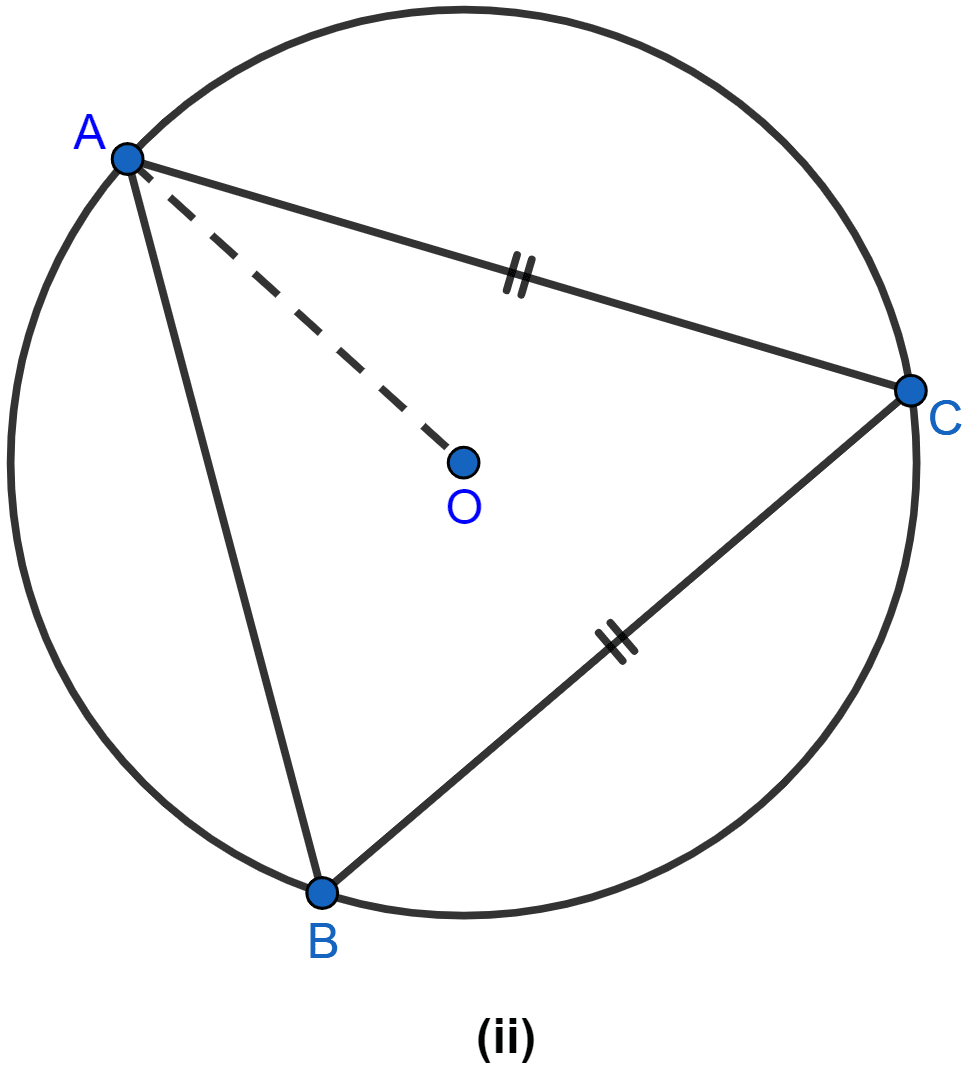
In the figure (ii) given below, O is the circumcenter of triangle ABC in which AC = BC. Given that ∠ACB = 56°, calculate
(i) ∠CAB
(ii) ∠OAC.
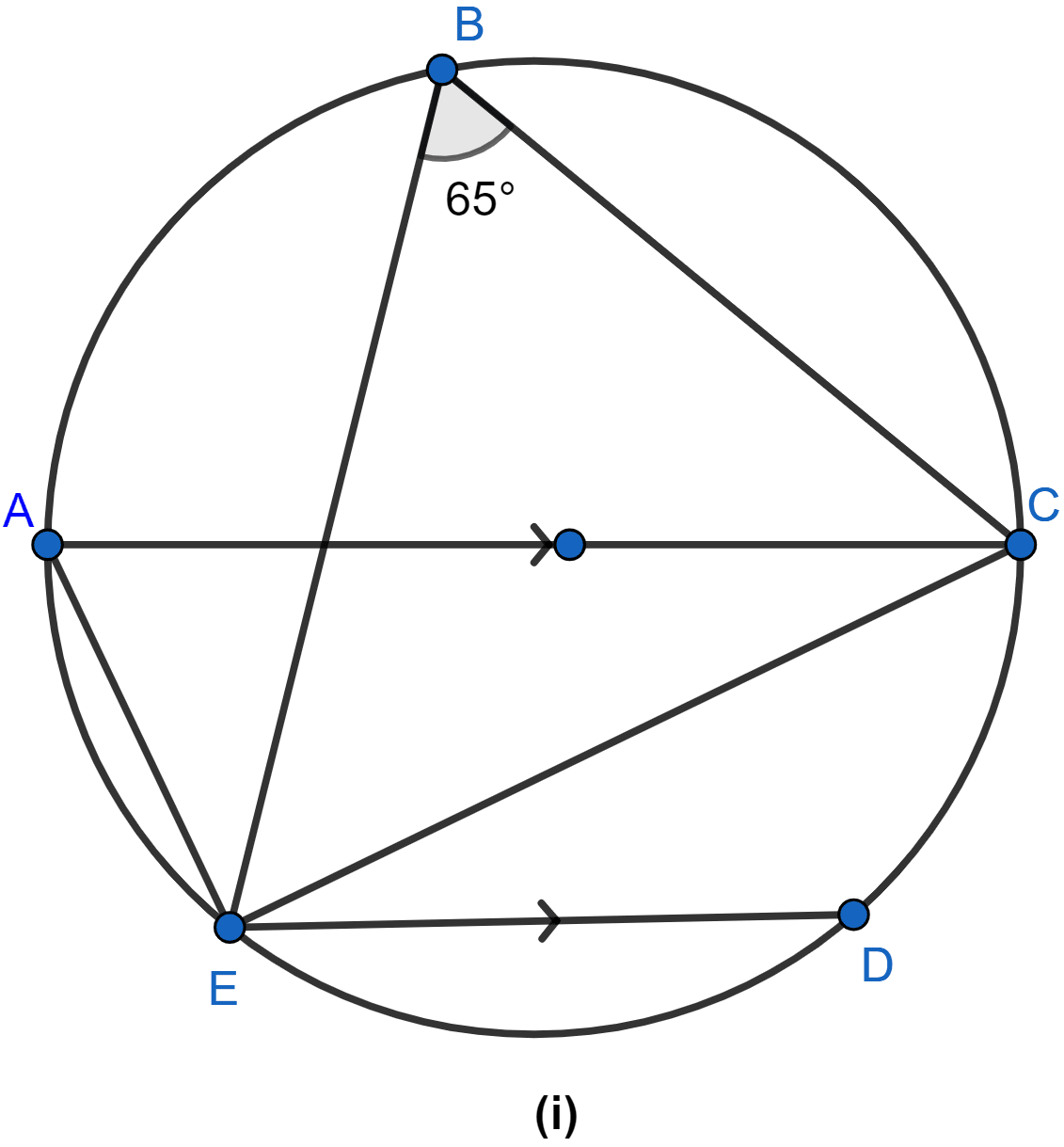
In the figure (i) given below, chord ED is parallel to the diameter AC of the circle. Given ∠CBE = 65°, calculate ∠DEC.
In the figure (i) given below, straight lines AB and CD pass through the center O of a circle. If ∠OCE = 40° and ∠AOD = 75°, find the number of degrees in
(i) ∠CDE
(ii) ∠OBE.

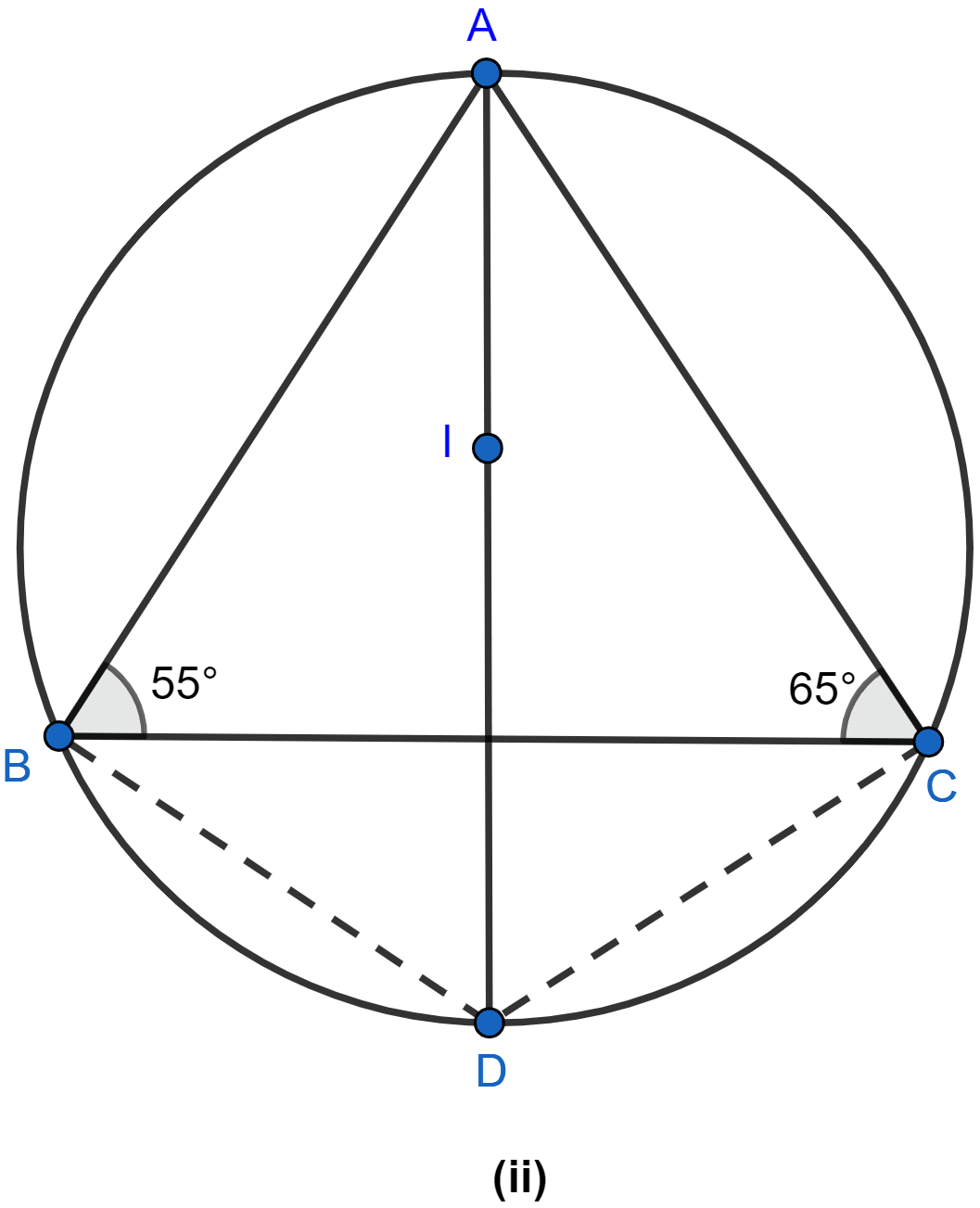
In the figure (ii) given below, I is the incentre of △ABC. AI produced meets the circumcircle of △ABC at D. Given that ∠ABC = 55° and ∠ACB = 65°, calculate
(i) ∠BCD
(ii) ∠CBD
(iii) ∠DCI
(iv) ∠BIC.