Mathematics
In the figure (i) given below, chord ED is parallel to the diameter AC of the circle. Given ∠CBE = 65°, calculate ∠DEC.
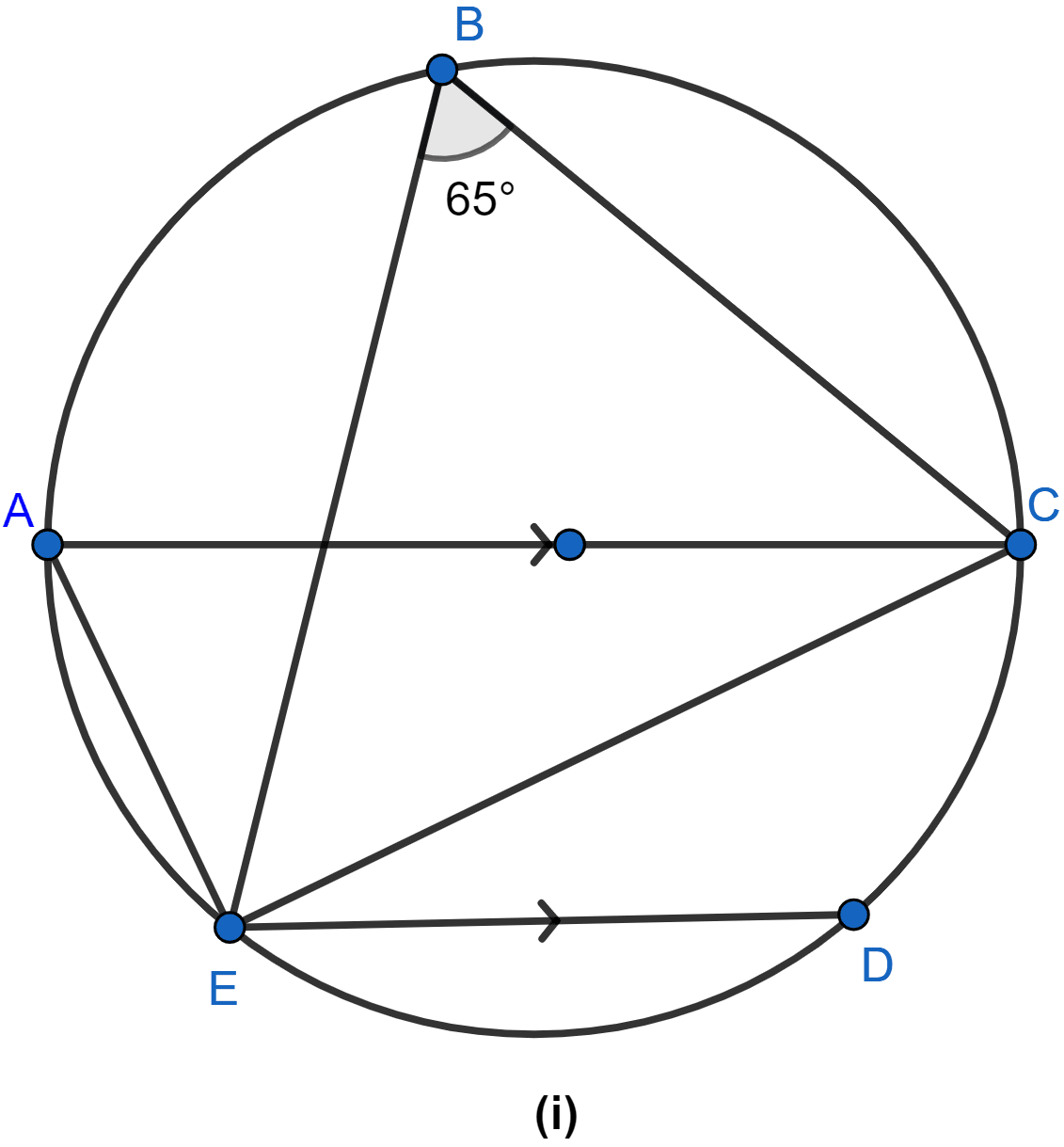
Answer
Consider △AEC and △EBC,
∠EAC = ∠EBC = 65° (∵ angles in same segment are equal.)
In △AEC,
∠AEC = 90° (∵ angle in semicircle is 90°.)
We know that sum of angles of a triangle is 180°.
⇒ ∠AEC + ∠EAC + ∠ACE = 180°.
⇒ 90° + 65° + ∠ACE = 180°
⇒ ∠ACE + 155° = 180°
⇒ ∠ACE = 180° - 155°
⇒ ∠ACE = 25°.
∠DEC = ∠ACE (∵ Alternate angles)
∴∠DEC = 25°
Hence, the value of ∠DEC = 25°.
Related Questions
In the figure (i) given below, P and Q are centers of two circles intersecting at B and C. ACD is a straight line. Calculate the value of x.

In the figure (ii) given below, C is a point on the minor arc AB of the circle with centre O. Given ∠ACB = p°, ∠AOB = q°, express q in terms of p. Calculate p if OACB is a parallelogram.
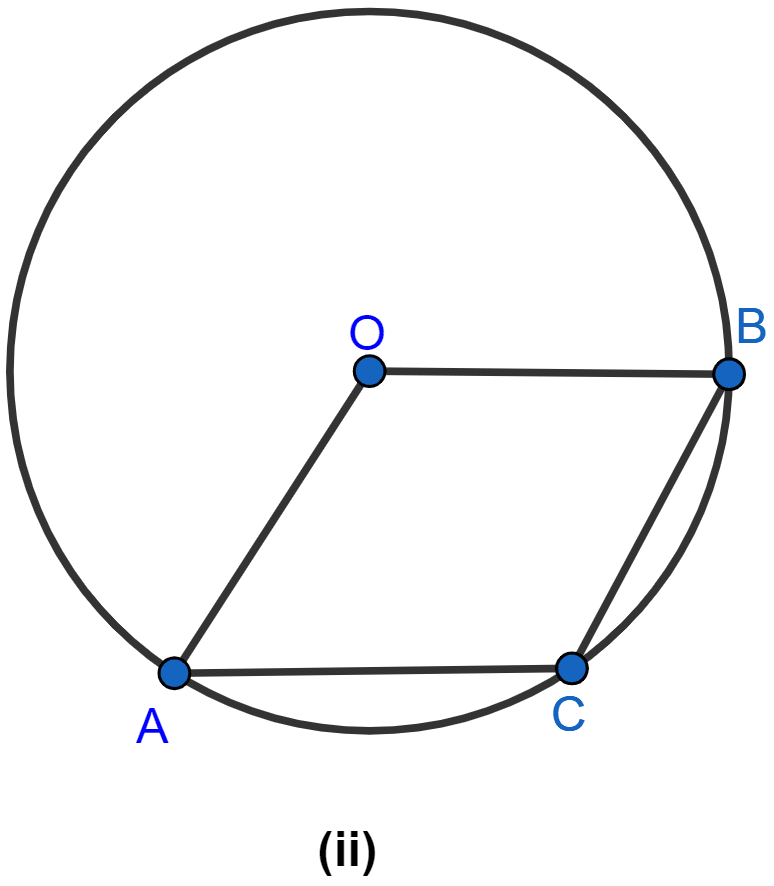
In the figure (ii) given below, O is the circumcenter of triangle ABC in which AC = BC. Given that ∠ACB = 56°, calculate
(i) ∠CAB
(ii) ∠OAC.
In the figure (i) given below, straight lines AB and CD pass through the center O of a circle. If ∠OCE = 40° and ∠AOD = 75°, find the number of degrees in
(i) ∠CDE
(ii) ∠OBE.
