KnowledgeBoat Logo
|

Mathematics

In the figure given below, ∠BAC = 90°, ADC = 90°, AD = 6 cm, CD = 8 cm and BC = 26 cm. Find

(i) AC

(ii) AB

(iii) area of the shaded region.

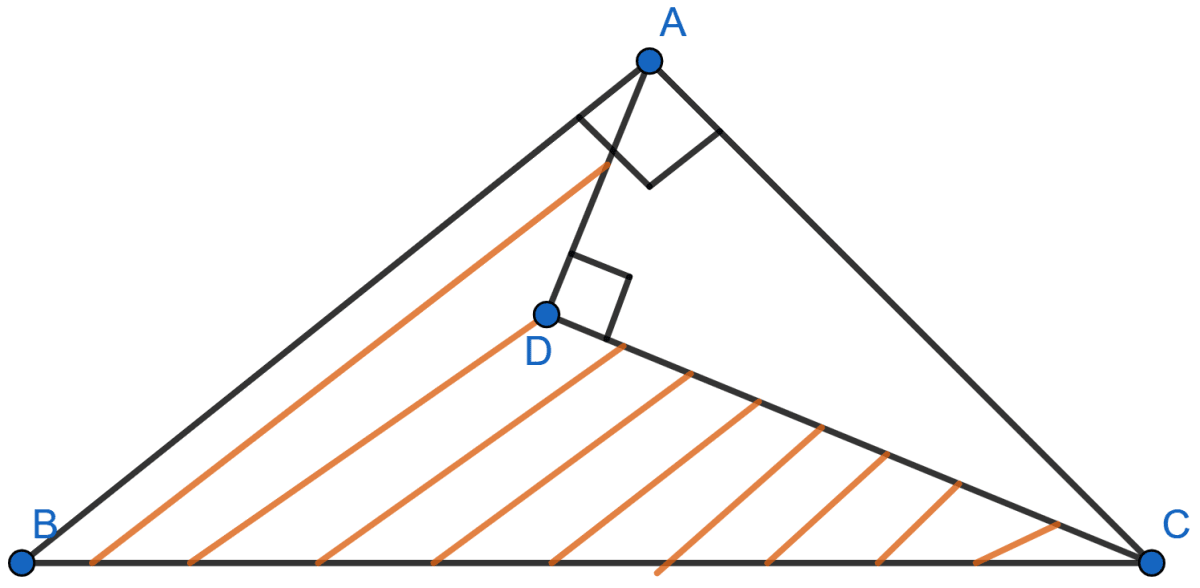
In the figure given below, ∠BAC = 90°, ADC = 90°, AD = 6 cm, CD = 8 cm and BC = 26 cm. Find AC AB area of the shaded region. Pythagoras Theorem, ML Aggarwal Understanding Mathematics Solutions ICSE Class 9.

Pythagoras Theorem

9 Likes

Answer

(i) By pythagoras theorem,

In right angle triangle ADC,

⇒ AC2 = AD2 + DC2

⇒ AC2 = 62 + 82

⇒ AC2 = 36 + 64

⇒ AC2 = 100

⇒ AC = 100\sqrt{100} = 10 cm.

Hence, AC = 10 cm.

(ii) By pythagoras theorem,

In right angle triangle ABC,

⇒ BC2 = AB2 + AC2

⇒ 262 = AB2 + 102

⇒ AB2 = 676 - 100

⇒ AB2 = 576

⇒ AB = 576\sqrt{576} = 24 cm.

Hence, AB = 24 cm.

(iii) Area of shaded region = Area of △ABC - Area of △ADC

=12×AB×AC−12×AD×DC=12×24×10−12×6×8=120−24=96 cm2.= \dfrac{1}{2} \times AB \times AC - \dfrac{1}{2} \times AD \times DC \\[1em] = \dfrac{1}{2} \times 24 \times 10 - \dfrac{1}{2} \times 6 \times 8 \\[1em] = 120 - 24 \\[1em] = 96 \text{ cm}^2.

Hence, area of shaded region = 96 cm2.

Answered By

5 Likes

Related Questions