Mathematics
If in △ABC, AB > AC and AD ⊥ BC, prove that AB2 - AC2 = BD2 - CD2.
Pythagoras Theorem
14 Likes
Answer
From figure,
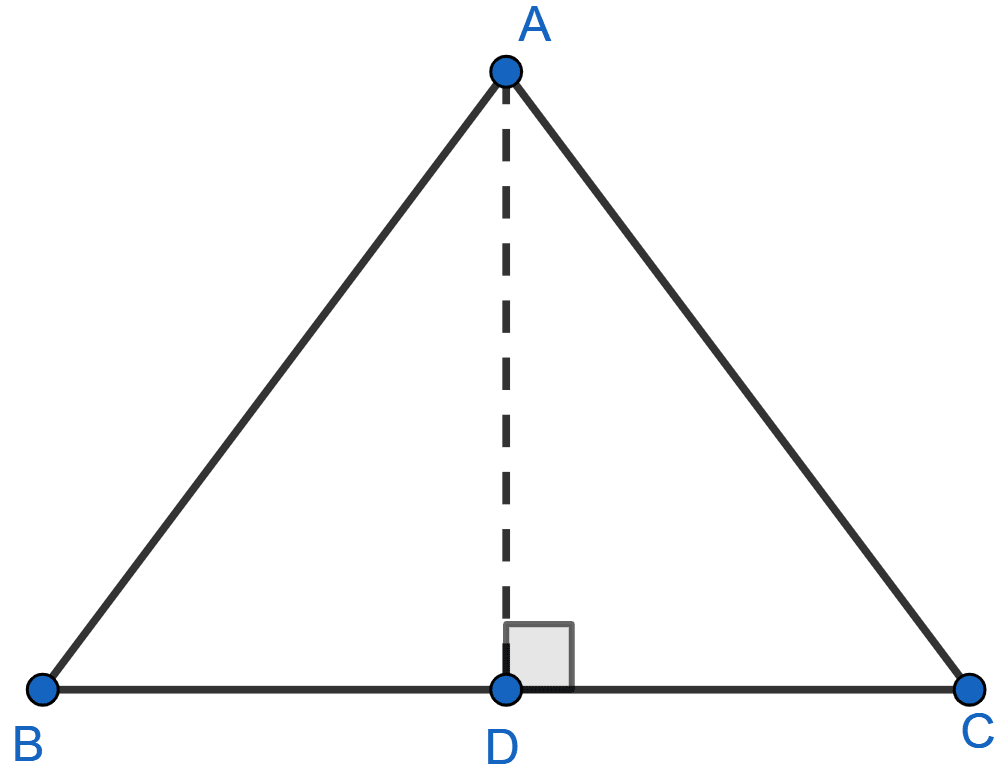
In right angle △ADB,
By pythagoras theorem we get,
AB2 = AD2 + BD2 ……..(i)
In right angle △ADC,
By pythagoras theorem we get,
AC2 = AD2 + CD2 ……..(ii)
Subtracting (ii) from (i),
AB2 - AC2 = AD2 + BD2 - (AD2 + CD2)
AB2 - AC2 = BD2 - CD2.
Hence, proved that AB2 - AC2 = BD2 - CD2.
Answered By
10 Likes
Related Questions
In the figure given below, ∠BAC = 90°, ADC = 90°, AD = 6 cm, CD = 8 cm and BC = 26 cm. Find
(i) AC
(ii) AB
(iii) area of the shaded region.
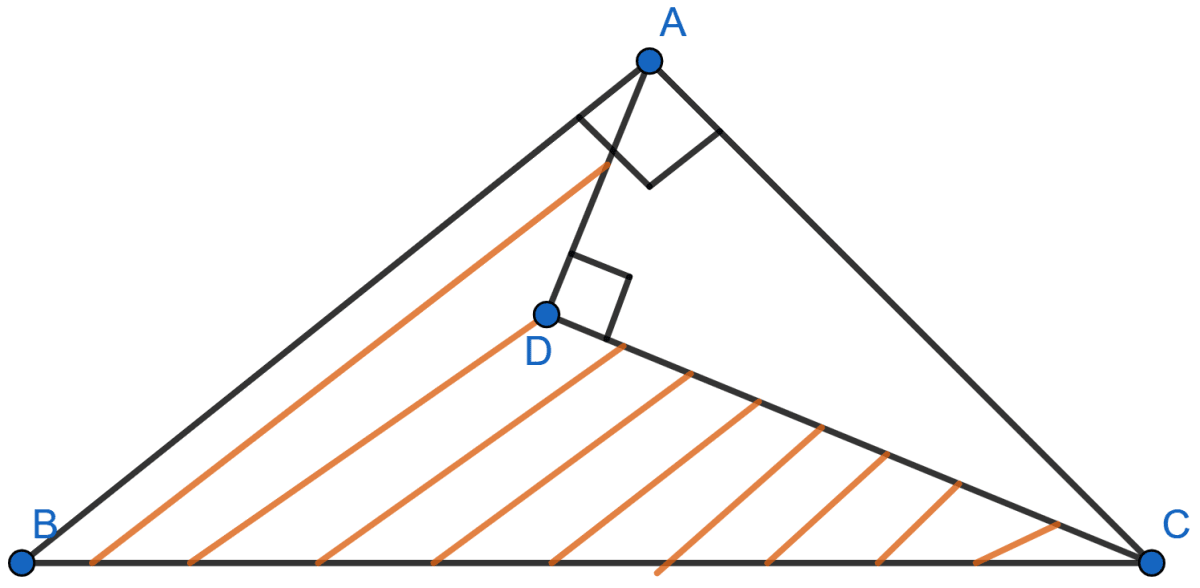
In figure given below, triangle ABC is right angled at B. Given that AB = 9 cm, AC = 15 cm and D, E are mid-points of the sides AB and AC respectively, calculate (i) the length of BC (ii) the area of △ADE.

In a right angled triangle ABC, right angled at C, P and Q are the points on the sides CA and CB respectively which divide these sides in the ratio 2 : 1. Prove that
(i) 9AQ2 = 9AC2 + 4BC2
(ii) 9BP2 = 9BC2 + 4AC2
(iii) 9(AQ2 + BP2) = 13AB2
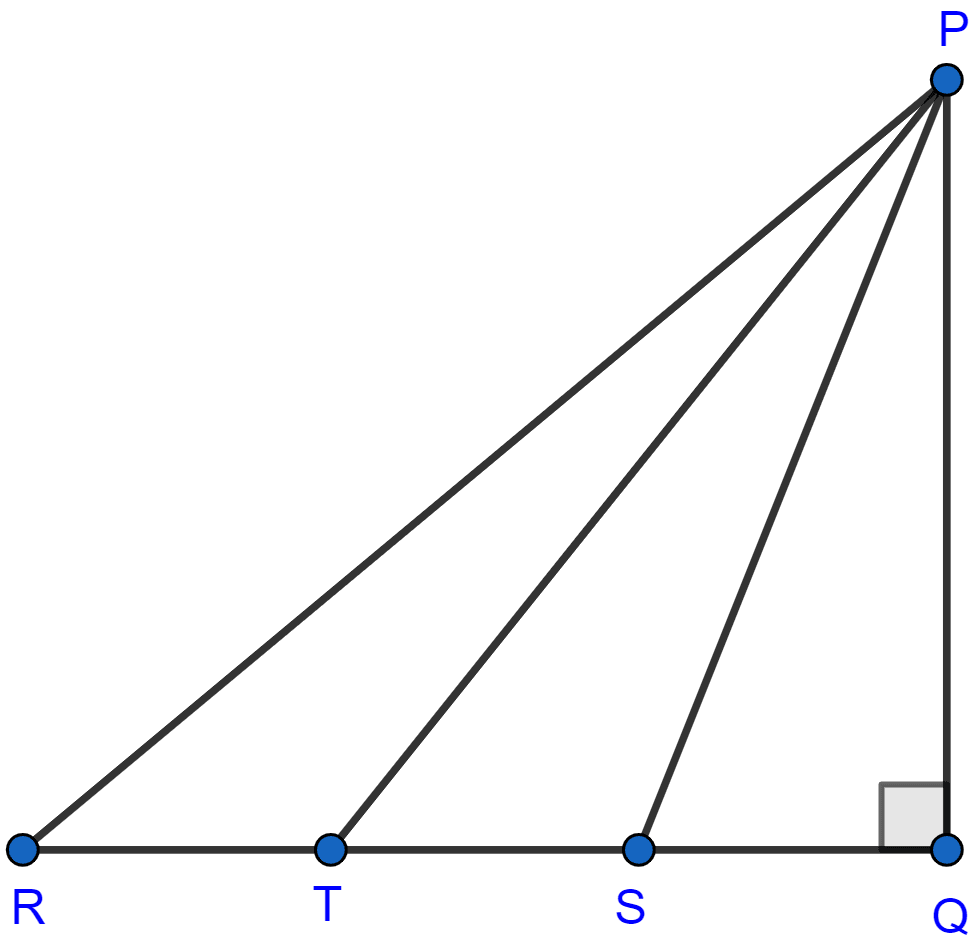
In the adjoining figure, △PQR is right angled at Q and points S and T trisect side QR. Prove that
8PT2 = 3PR2 + 5PS2.