Mathematics
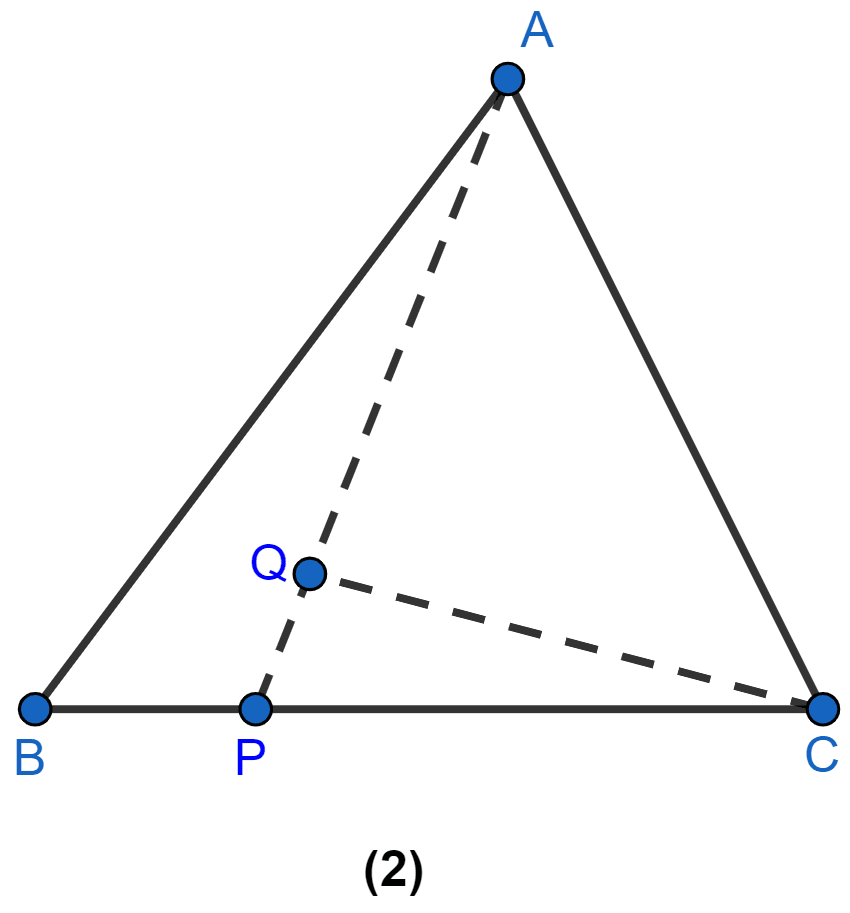
In the figure (2) given below, P is a point on the side BC of ∆ABC such that PC = 2BP, and Q is a point on AP such that QA = 5PQ, find area of ∆AQC : area of ∆ABC.
Theorems on Area
15 Likes
Answer
Given, PC = 2BP
From figure,
BC = BP + PC = BP + 2BP = 3BP.
PC = BC
Let AD be the altitude.
Area of △ABC = × BC × AD ……(i)
Area of △APC = × PC × AD
= ……..(ii)
Dividing (ii) by (i) we get,
Given,
QA = 5PQ
From figure,
AP = AQ + QP = 5PQ + PQ = 6PQ.
.
AQ =
Let CE be the altitude.
Area of △AQC = × AQ × CE ……(iv)
Area of △APC = × AP × CE …….(v)
Dividing (iv) by (v) we get,
∴ area of △AQC : area of △ABC = 5 : 9.
Hence, area of △AQC : area of △ABC = 5 : 9.
Answered By
10 Likes
Related Questions
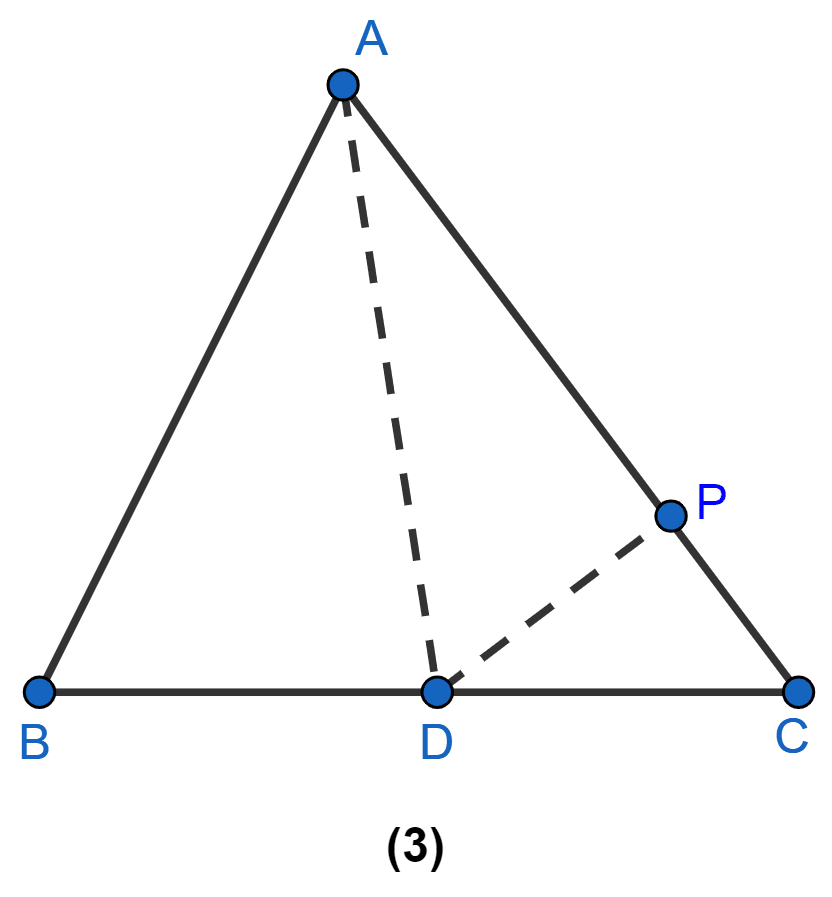
In the figure (3) given below, AD is a median of △ABC and P is a point in AC such that area of △ADP : area of △ABD = 2 : 3. Find
(i) AP : PC
(ii) area of △PDC : area of △ABC
In the adjoining figure, D, E and F are midpoints of the sides BC, CA and AB respectively of ∆ABC. Prove that BCEF is a trapezium and area of the trap. BCEF = area of ∆ABC.

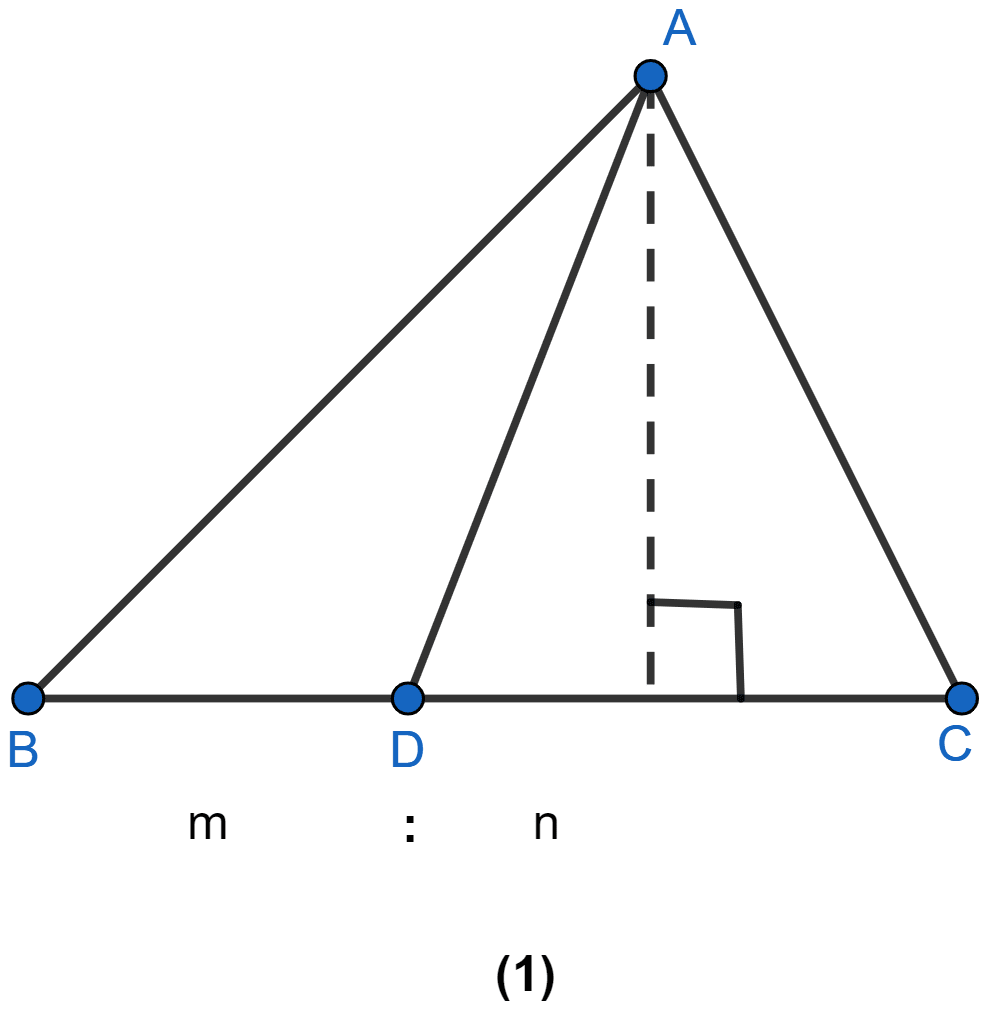
In figure (1) given below, point D divides the side BC of ∆ABC in the ratio m : n. Prove that area of ∆ABD : area of ∆ADC = m : n.
In figure (1) given below, area of parallelogram ABCD is 29 cm2. Calculate the height of parallelogram ABEF if AB = 5.8 cm.
