Mathematics
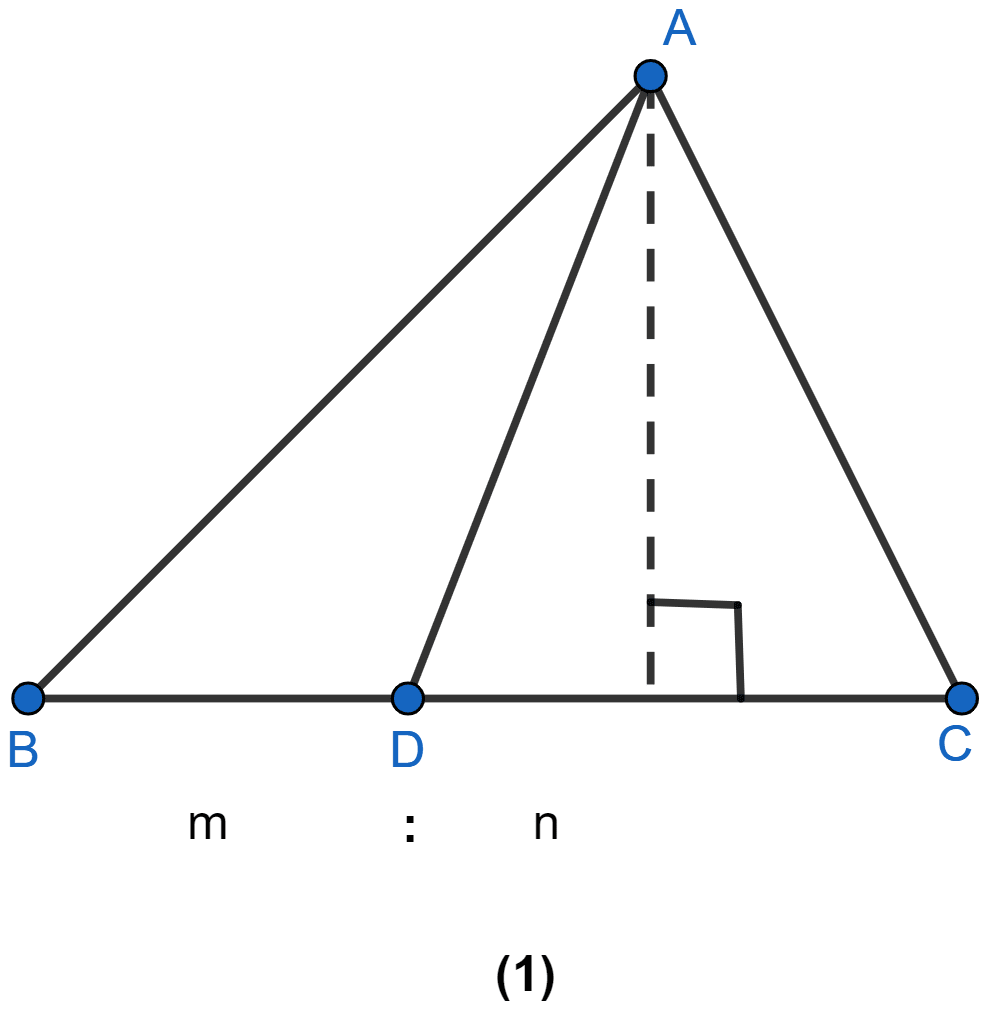
In figure (1) given below, point D divides the side BC of ∆ABC in the ratio m : n. Prove that area of ∆ABD : area of ∆ADC = m : n.
Theorems on Area
22 Likes
Answer
From fig (1)
In ∆ABC, point D divides the side BC in the ratio m : n.
BD : DC = m : n
area of ∆ABD = × base × height
= × BD × AE …….. (i)
area of ∆ADC = × DC × AE …… (ii)
Dividing (i) by (ii)
Hence, proved that area of ∆ABD : area of ∆ADC = m : n.
Answered By
13 Likes
Related Questions
D, E and F are mid-points of the sides BC, CA and AB respectively of a ∆ABC. Prove that
(i) FDCE is a parallelogram
(ii) area of ∆DEF = area of ∆ABC
(iii) area of || gm FDCE = area of ∆ABC
In the adjoining figure, D, E and F are midpoints of the sides BC, CA and AB respectively of ∆ABC. Prove that BCEF is a trapezium and area of the trap. BCEF = area of ∆ABC.

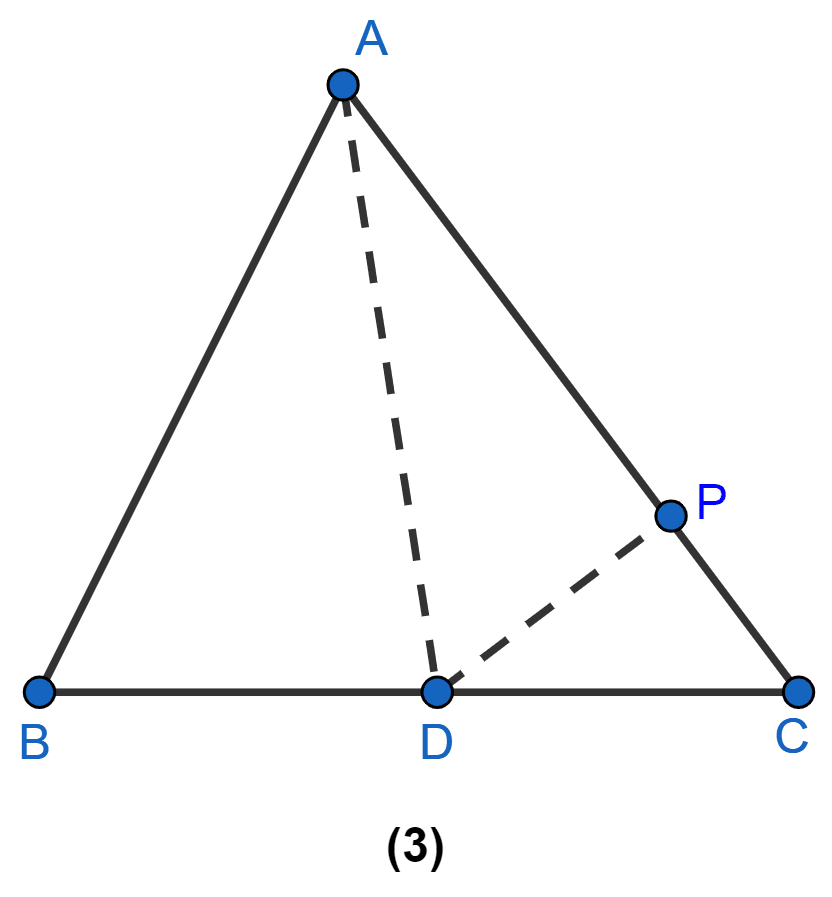
In the figure (2) given below, P is a point on the side BC of ∆ABC such that PC = 2BP, and Q is a point on AP such that QA = 5PQ, find area of ∆AQC : area of ∆ABC.
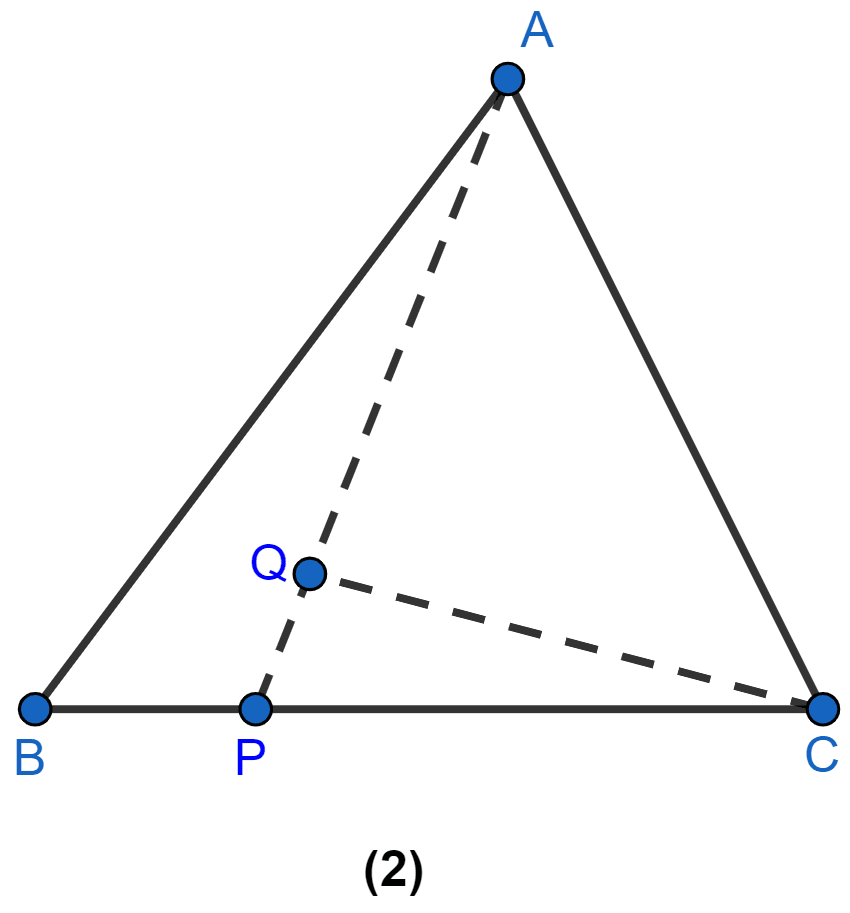
In the figure (3) given below, AD is a median of △ABC and P is a point in AC such that area of △ADP : area of △ABD = 2 : 3. Find
(i) AP : PC
(ii) area of △PDC : area of △ABC