Mathematics
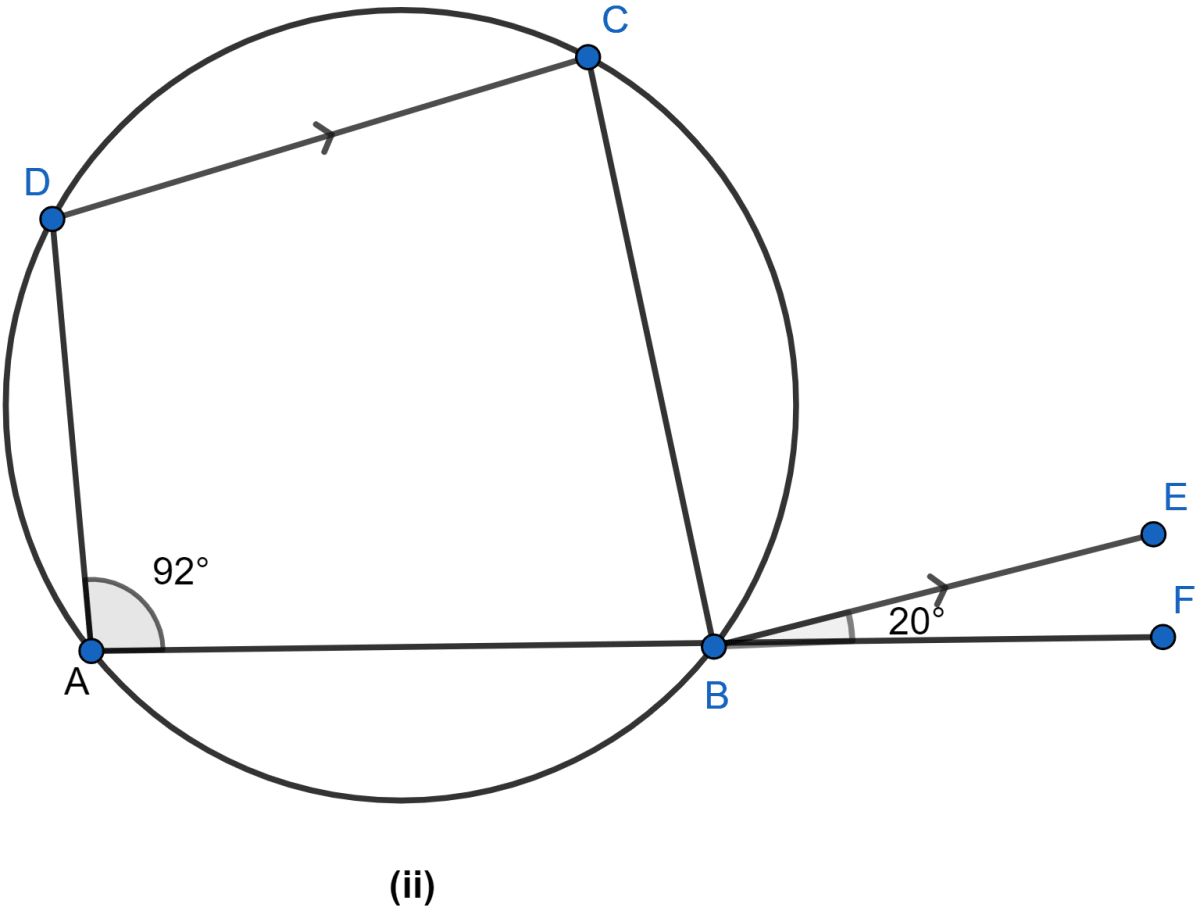
In figure (ii) given below, ABF is a straight line and BE || DC. If ∠DAB = 92° and ∠EBF = 20°, find
(i) ∠BCD
(ii) ∠ADC
Circles
53 Likes
Answer
(i) Sum of opposite angles of cyclic quadrilateral = 180°
⇒ ∠DAB + ∠BCD = 180°
⇒ 92° + ∠BCD = 180°
⇒ ∠BCD = 180° - 92°
⇒ ∠BCD = 88°.
Hence, the value of ∠BCD = 88°.
(ii) ∠CBE = ∠BCD = 88° (∵ ∠CBE and ∠BCD are alternate angles)
∵ exterior angle of a cyclic quadrilateral is equal to the opposite interior angle.
⇒ ∠ADC = ∠CBF
⇒ ∠ADC = ∠CBE + ∠EBF
⇒ ∠ADC = 88° + 20°
⇒ ∠ADC = 108°.
Hence, the value of ∠ADC = 108°.
Answered By
34 Likes
Related Questions
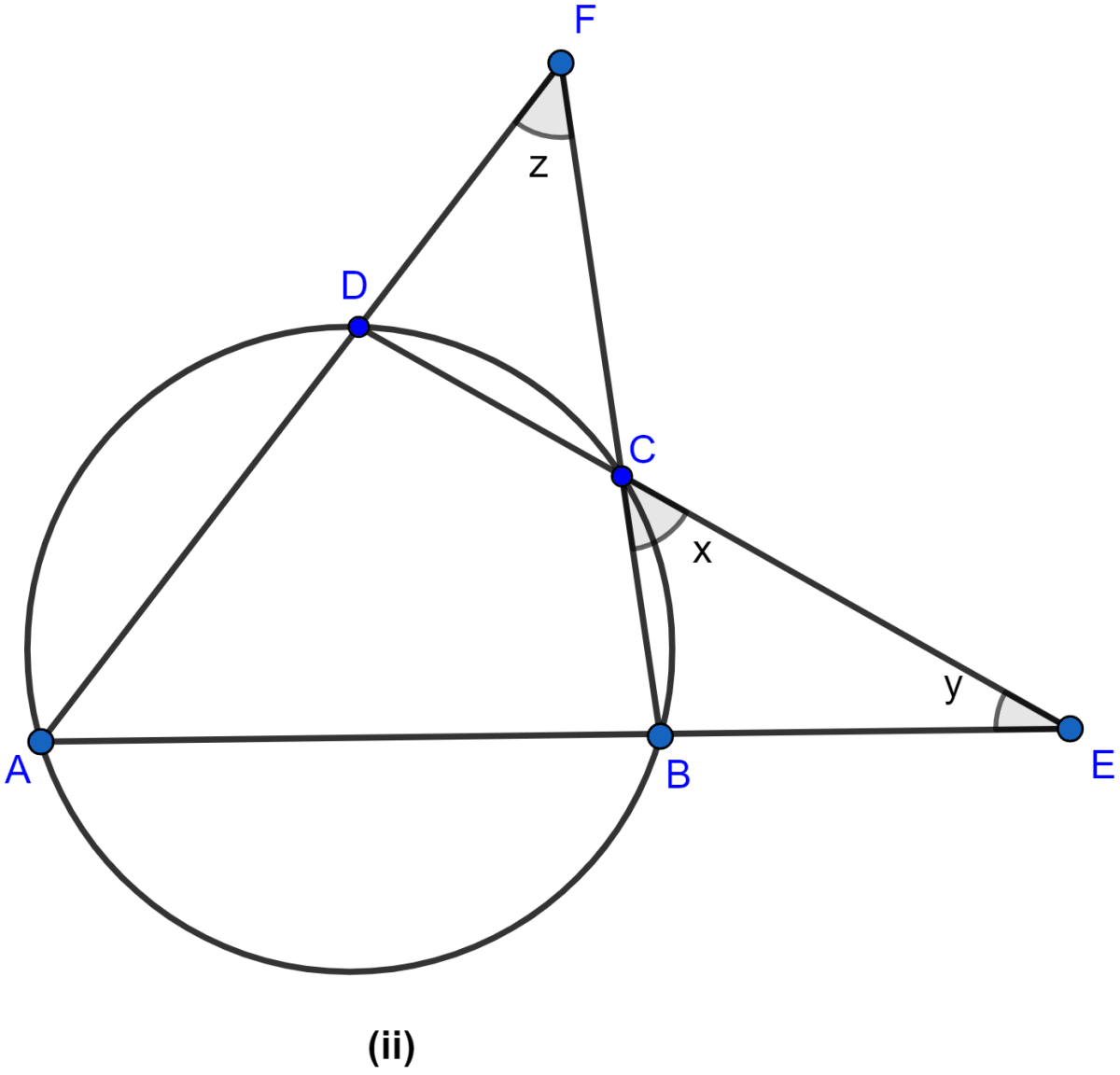
In the figure (ii) given below, sides AB and DC of a cyclic quadrilateral ABCD are produced to meet at E, the sides AD and BC are produced to meet at F. If x : y : z = 3 : 4 : 5, find the values of x, y and z.
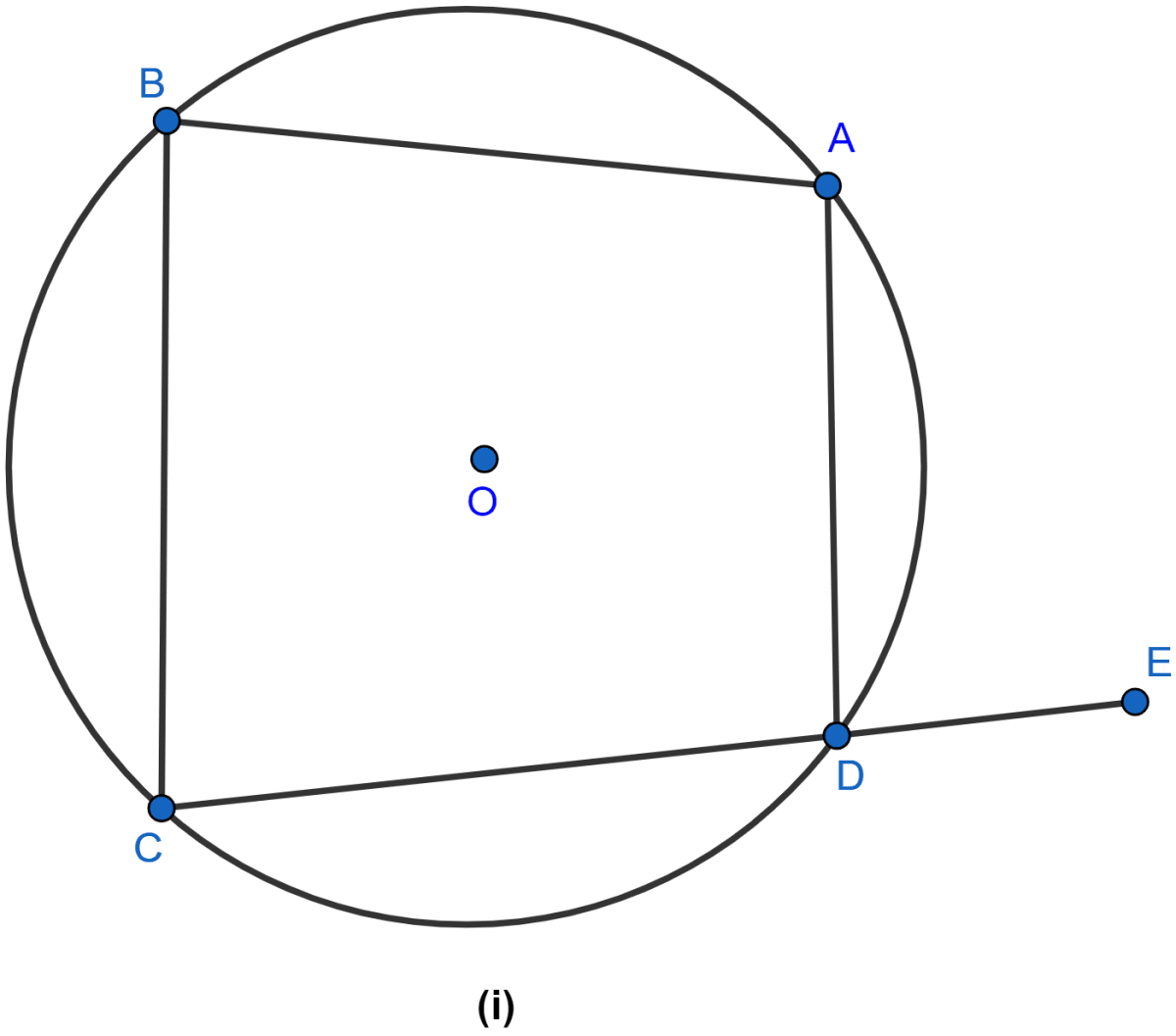
In the figure (i) given below, ABCD is a quadrilateral inscribed in a circle with centre O. CD is produced to E. If ∠ADE = 70° and ∠OBA = 45°, calculate
(i) ∠OCA
(ii) ∠BAC
In the figure (i) given below, PQRS is a cyclic quadrilateral in which PQ = QR and RS is produced to T. If ∠QPR = 52°, calculate ∠PST.

In the figure (ii) given below, O is the center of the circle. If ∠OAD = 50°, find the values of x and y.
