Mathematics
In cyclic quadrilateral ABCD, ∠A = 3∠C and ∠D = 5∠B. Find the measure of each angle of the quadrilateral.
Circles
2 Likes
Answer
Given, cyclic quadrilateral ABCD
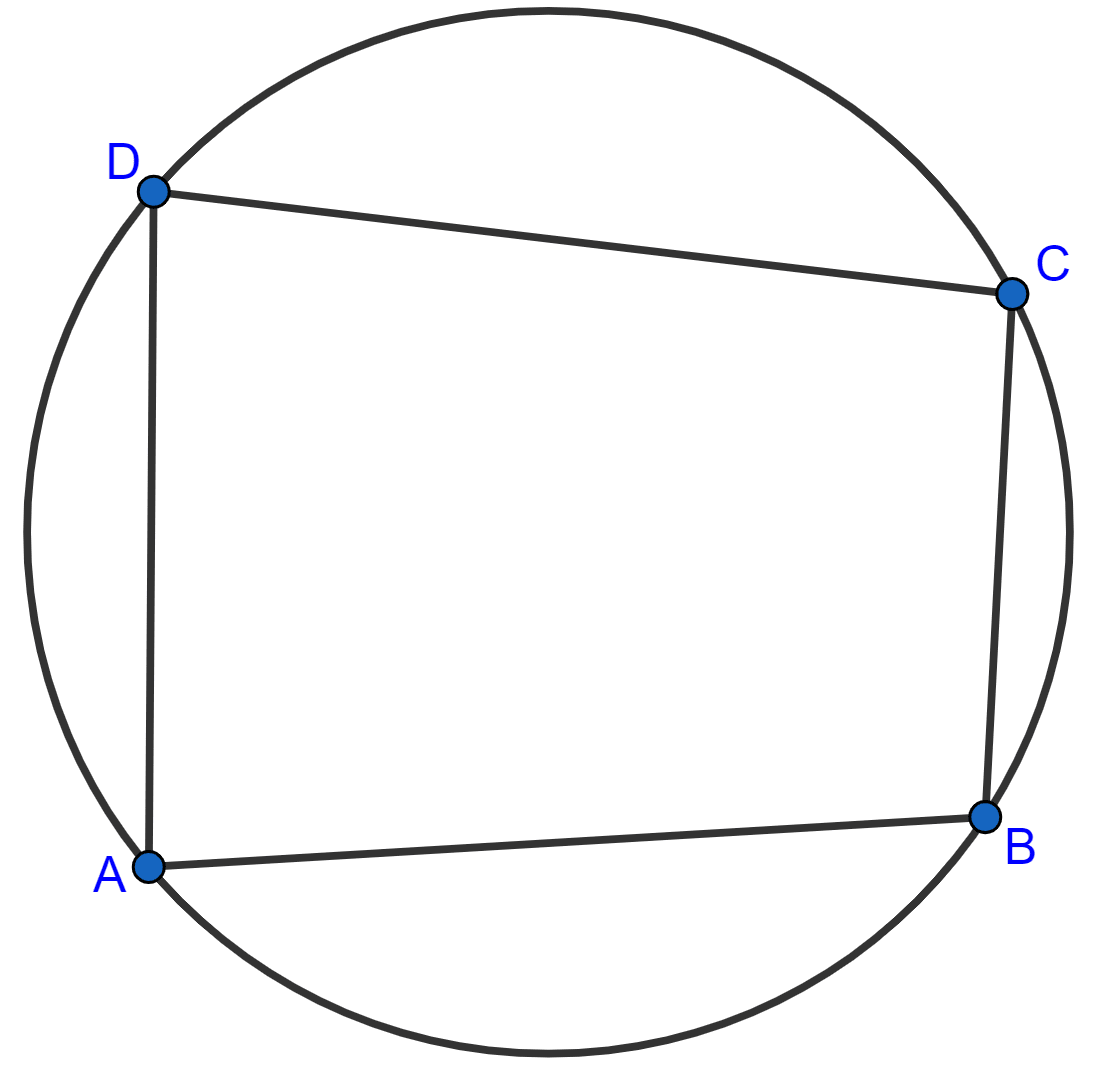
So, ∠A + ∠C = 180° [Opposite angles in a cyclic quadrilateral is supplementary]
⇒ 3∠C + ∠C = 180° [As ∠A = 3∠C]
⇒ 4∠C = 180°
⇒ ∠C =
⇒ ∠C = 45°.
Now,
⇒ ∠A = 3∠C = 3 x 45° = 135°.
Similarly,
⇒ ∠B + ∠D = 180°
⇒ ∠B + 5∠B = 180° [As, ∠D = 5∠B]
⇒ 6∠B = 180°
⇒ ∠B =
⇒ ∠B = 30°.
Now,
⇒ ∠D = 5∠B = 5 x 30° = 150°.
Hence, ∠A = 135°, ∠B = 30°, ∠C = 45° and ∠D = 150°.
Answered By
2 Likes
Related Questions
ABCD is a cyclic quadrilateral in which BC is parallel to AD, angle ADC = 110° and angle BAC = 50°. Find angle DAC and angle DCA.
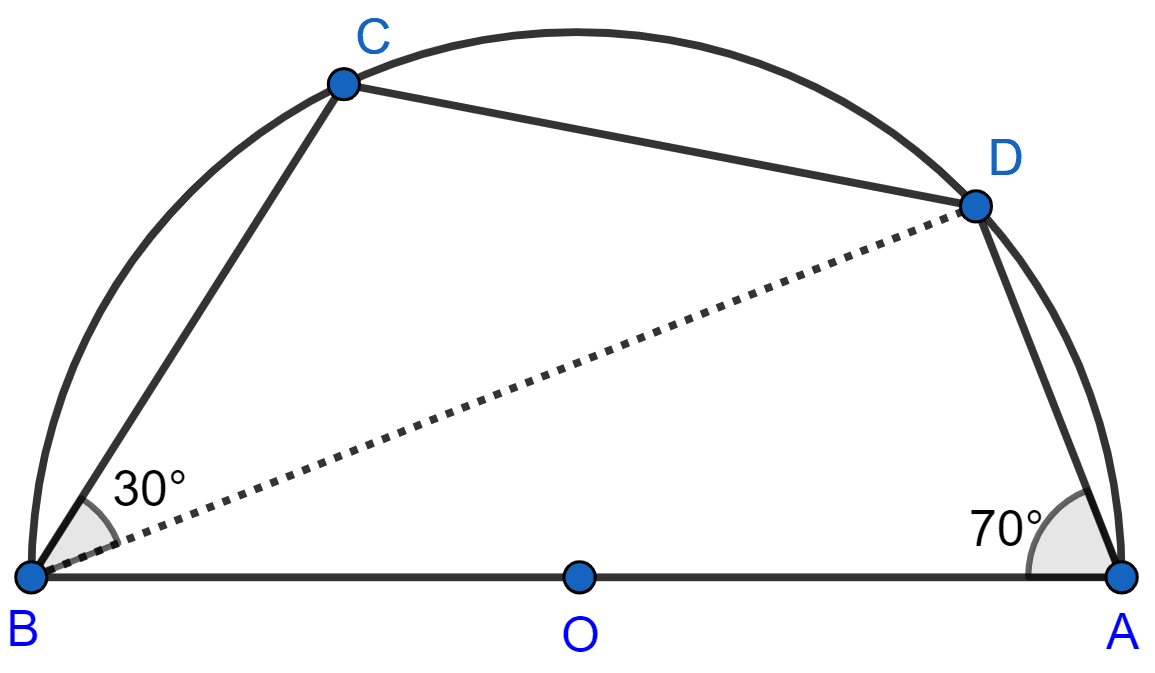
In the given figure, C and D are points on the semi-circle described on AB as diameter.
Given angle BAD = 70° and angle DBC = 30°, calculate angle BDC.
Show that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
Bisectors of vertex angles A, B and C of a triangle ABC intersect its circumcircle at points D, E and F respectively. Prove that angle EDF = 90° - ∠A.