Mathematics
Show that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
Circles
6 Likes
Answer
Let circle be drawn on one of the equal sides AB of the isosceles triangle ABC as shown in the figure below:
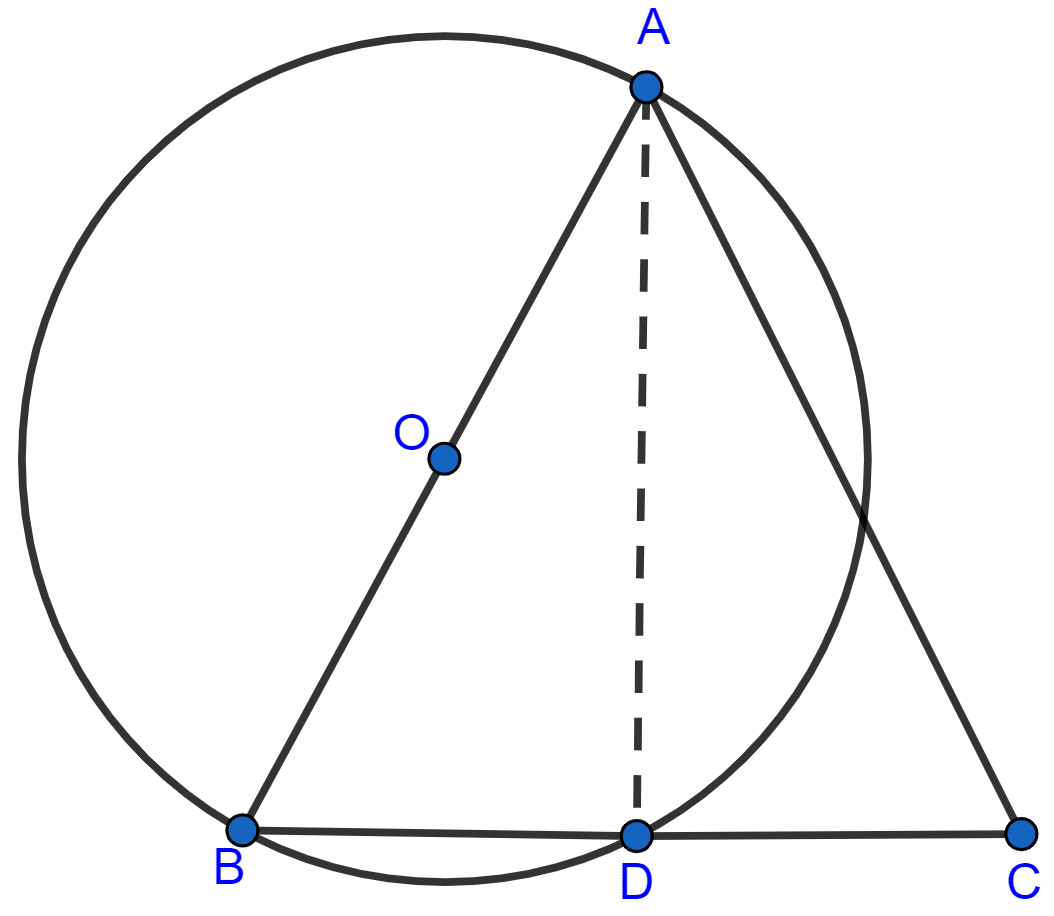
We have ∠ADB = 90° [Angle in a semi-circle is a right angle]
But,
⇒ ∠ADB + ∠ADC = 180° [Linear pair]
⇒ 90° + ∠ADC = 180°
⇒ ∠ADC = 180° - 90°
⇒ ∠ADC = 90°.
In ∆ABD and ∆ACD, we have
⇒ ∠ADB = ∠ADC [Each 90°]
⇒ AB = AC [Given]
⇒ AD = AD [Common]
Hence, ∆ABD ≅ ∆ACD by RHS congruence criterion.
By, C.P.C.T we get :
BD = DC
Hence, the circle bisects base BC at D.
Answered By
3 Likes
Related Questions
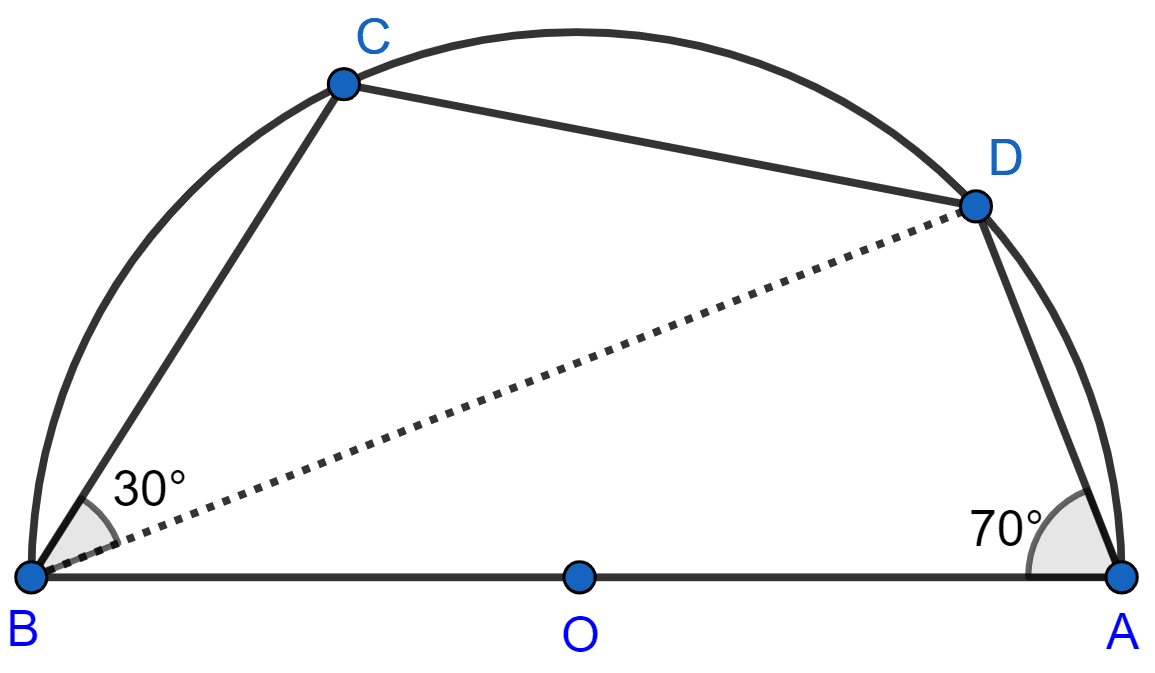
In the given figure, C and D are points on the semi-circle described on AB as diameter.
Given angle BAD = 70° and angle DBC = 30°, calculate angle BDC.
In cyclic quadrilateral ABCD, ∠A = 3∠C and ∠D = 5∠B. Find the measure of each angle of the quadrilateral.
Bisectors of vertex angles A, B and C of a triangle ABC intersect its circumcircle at points D, E and F respectively. Prove that angle EDF = 90° - ∠A.
In the figure, AB is the chord of a circle with centre O and DOC is a line segment such that BC = DO. If ∠C = 20°, find angle AOD.