Bisectors of vertex angles A, B and C of a triangle ABC intersect its circumcircle at points D, E and F respectively. Prove that angle EDF = 90° - $\dfrac{1}{2}$∠A.

BE is the bisector of ∠B.

⇒ ∠ABE = $\dfrac{∠B}{2}$

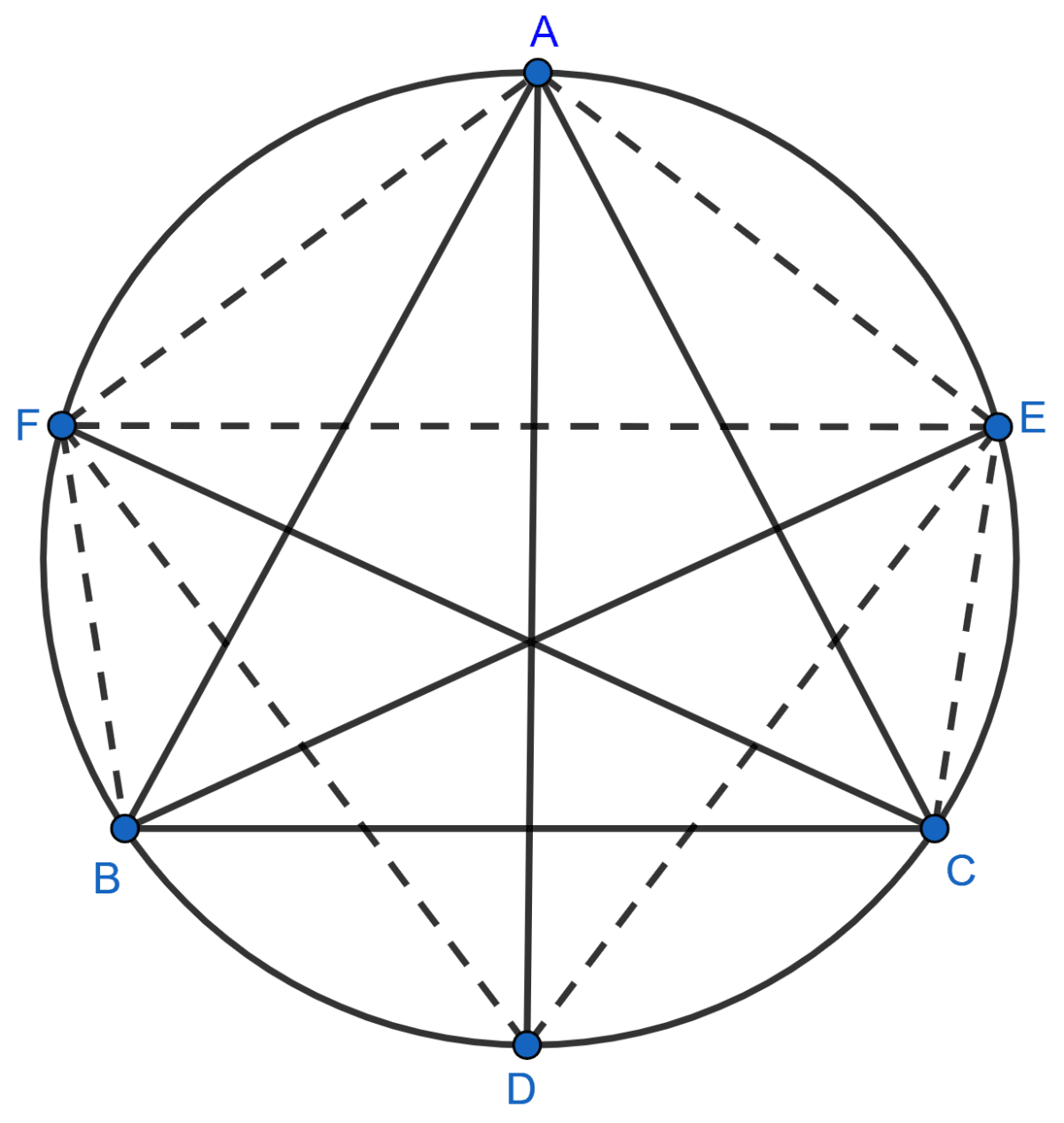
From figure,

⇒ ∠ADE = ∠ABE [Angles in same segment are equal]

⇒ ∠ADE = $\dfrac{∠B}{2}$ ………..(1)

Also,

FC is the bisector of ∠C.

⇒ ∠ACF = $\dfrac{∠C}{2}$

⇒ ∠ACF = ∠ADF [Angles in same segment are equal]

⇒ ∠ADF = $\dfrac{∠C}{2}$ ………….(2)

From figure,

⇒ ∠D = ∠ADE + ∠ADF

⇒ ∠D = $\dfrac{∠B + ∠C}{2}$ ………..(3)

In triangle ABC,

⇒ ∠A + ∠B + ∠C = 180°

⇒ ∠B + ∠C = 180° - ∠A

Substituting above value in (3), we get :

⇒ ∠D = $\dfrac{180° - ∠A}{2}$

⇒ ∠D = 90° - $\dfrac{1}{2}$∠A.

From figure,

∠EDF = ∠D.

Hence, proved that ∠EDF = $90° - \dfrac{1}{2}∠A$.