P is the midpoint of an arc APB of a circle. Prove that the tangent drawn at P will be parallel to the chord AB.

We know that,

The angle between a tangent and a chord through the point of contact is equal to the angle in the alternate segment, we have :

From figure,

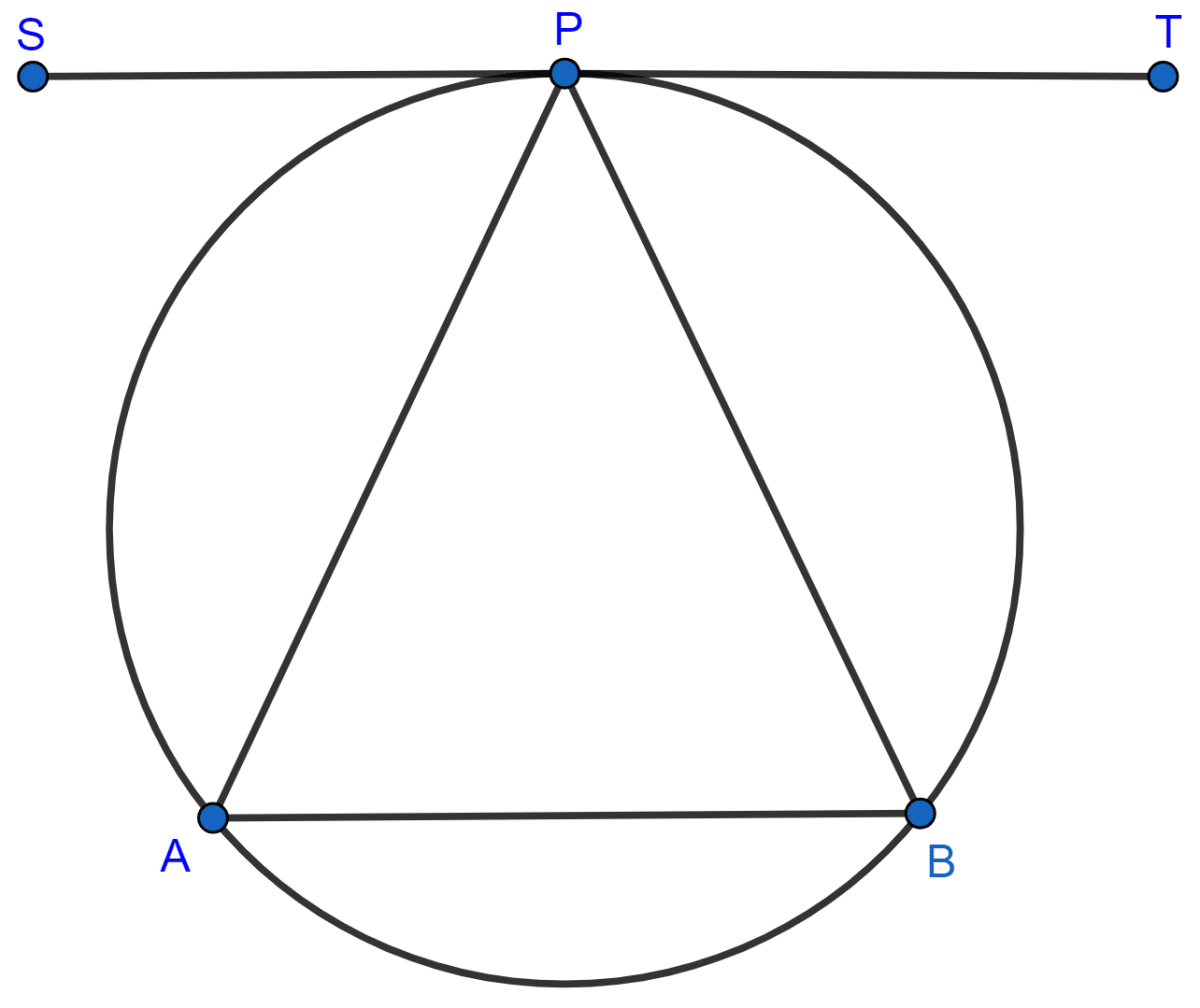
As, TPS is a tangent and PA is the chord of the circle.

∠BPT = ∠PAB [Angles in alternate segments are equal] ……….(1)

But,

∠PBA = ∠PAB [Since, PA = PB as P is mid-point of arc APB.] ……..(2)

From (1) and (2), we get :

∠BPT = ∠PBA

The above angles are alternate angles,

∴ TPS || AB

Hence, proved that the tangent drawn at P will be parallel to the chord AB.