Mathematics

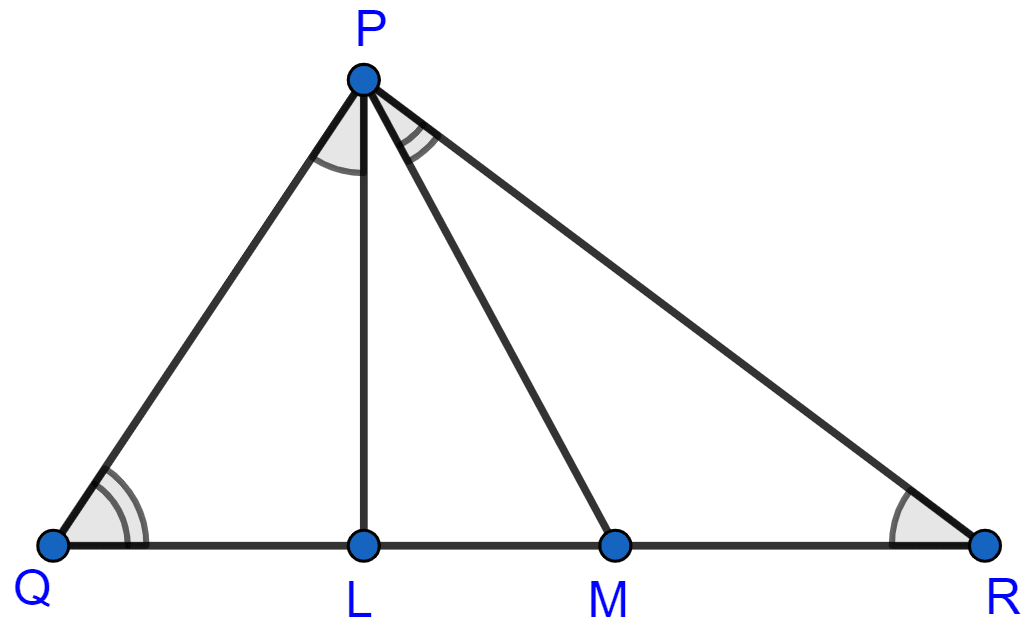
In a triangle PQR, L and M are two points on the base QR, such that ∠LPQ = ∠QRP and ∠RPM = ∠RQP. Prove that :
(i) △PQL ~ △RPM
(ii) QL × RM = PL × PM
(iii) PQ² = QR × QL

9 Likes

(i) In △PQL and △RPM

∠LPQ = ∠MRP (Given ∠LPQ = ∠QRP)

∠LQP = ∠RPM (Given ∠RPM = ∠RQP)

∴ △PQL ~ △RPM [By AA]

Hence, proved that △PQL ~ △RPM.

(ii) Since, △PQL ~ △RPM and corresponding sides of similar triangle are proportional to each other.

$\therefore \dfrac{QL}{PM} = \dfrac{PL}{RM} \\[1em] \Rightarrow QL \times RM = PL \times PM.$

Hence, proved that QL × RM = PL × PM.

(iii) In △PQL and △RQP

∠LPQ = ∠QRP (Given)

∠Q = ∠Q [Common]

∴ △PQL ~ △RQP [By AA]

Since, corresponding sides of similar triangle are proportional to each other.

$\therefore \dfrac{PQ}{RQ} = \dfrac{QL}{QP} \\[1em] \Rightarrow PQ^2 = QR \times QL.$

Hence, proved that PQ² = QR x QL.

Answered By

5 Likes