Mathematics
If }[r] x & 6 \ y & 8 \end{bmatrix} + 3 \times \begin{bmatrix}[r] 1 & -1 \ 0 & 2 \end{bmatrix} = 3 \times \begin{bmatrix}[r] 3 & 3 \ 4 & 6 \end{bmatrix}, find the values of x and y.
Matrices
2 Likes
Answer
Solving,
}[r] x & 6 \ y & 8 \end{bmatrix} + 3 \times \begin{bmatrix}[r] 1 & -1 \ 0 & 2 \end{bmatrix} = 3 \times \begin{bmatrix}[r] 3 & 3 \ 4 & 6 \end{bmatrix} \\[1em] \Rightarrow \begin{bmatrix}[r] 2x & 12 \ 2y & 16 \end{bmatrix} + \begin{bmatrix}[r] 3 & -3 \ 0 & 6 \end{bmatrix} = \begin{bmatrix}[r] 9 & 9 \ 12 & 18 \end{bmatrix} \\[1em] \Rightarrow \begin{bmatrix}[r] 2x + 3 & 12 + (-3) \ 2y + 0 & 16 + 6 \end{bmatrix} = \begin{bmatrix}[r] 9 & 9 \ 12 & 18 \end{bmatrix} \\[1em] \Rightarrow \begin{bmatrix}[r] 2x + 3 & 9 \ 2y & 22 \end{bmatrix} = \begin{bmatrix}[r] 9 & 9 \ 12 & 18 \end{bmatrix} \\[1em]
From above equation we get,
⇒ 2x + 3 = 9 and 2y = 12
⇒ 2x = 6 and y =
⇒ x = = 3 and y = 6.
Hence, x = 3 and y = 6.
Answered By
2 Likes
Related Questions
Solve the quadratic equation x2 - 3(x + 3) = 0 and give your answer correct to two significant figures.
The following table shows the distribution of the marks obtained by 50 students in an aptitude test.
Marks obtained No. of students 15 6 18 18 21 12 24 8 27 4 30 2 Find the mean, the median and the mode of the distribution.
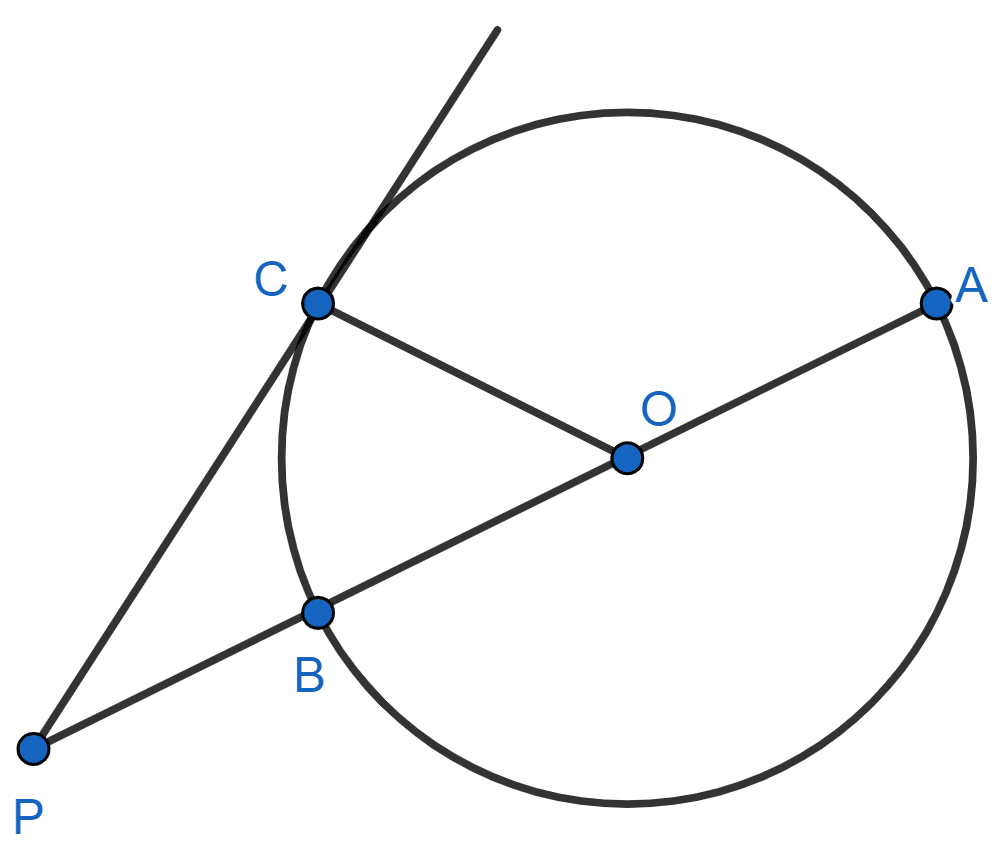
In the given figure, AB is diameter and PC is tangent to the circle with center O. If AP = 40 cm, CP = 20 cm, find the radius of the circle.
Using remainder theorem, factorise 6x3 - 11x2 - 3x + 2 completely.