Mathematics
The following table shows the distribution of the marks obtained by 50 students in an aptitude test.
| Marks obtained | No. of students |
|---|---|
| 15 | 6 |
| 18 | 18 |
| 21 | 12 |
| 24 | 8 |
| 27 | 4 |
| 30 | 2 |
Find the mean, the median and the mode of the distribution.
Statistics
1 Like
Answer
| Marks obtained (x) | No. of students (f) | Cumulative frequency | fx |
|---|---|---|---|
| 15 | 6 | 6 | 90 |
| 18 | 18 | 24 | 324 |
| 21 | 12 | 36 | 252 |
| 24 | 8 | 44 | 192 |
| 27 | 4 | 48 | 108 |
| 30 | 2 | 50 | 60 |
| Total | 50 | 1026 |
By formula,
Mean = = 20.52
From table,
n (no. of terms) = 50, which is even.
By formula,
Median = th term
= = 25th term.
From table,
Marks obtained from 25th to 36th student = 21.
∴ Median = 21.
Maximum students have scored 18 marks.
∴ Mode = 18.
Hence, mean = 20.52, median = 21 and mode = 18.
Answered By
2 Likes
Related Questions
For the inter-state supply of the following goods/services, find the amount of bill :
MRP (in ₹) GST % Discount % 2500 12 20 3000 18 30 4000 5 25 5000 12 40 Solve the quadratic equation x2 - 3(x + 3) = 0 and give your answer correct to two significant figures.
If }[r] x & 6 \ y & 8 \end{bmatrix} + 3 \times \begin{bmatrix}[r] 1 & -1 \ 0 & 2 \end{bmatrix} = 3 \times \begin{bmatrix}[r] 3 & 3 \ 4 & 6 \end{bmatrix}, find the values of x and y.
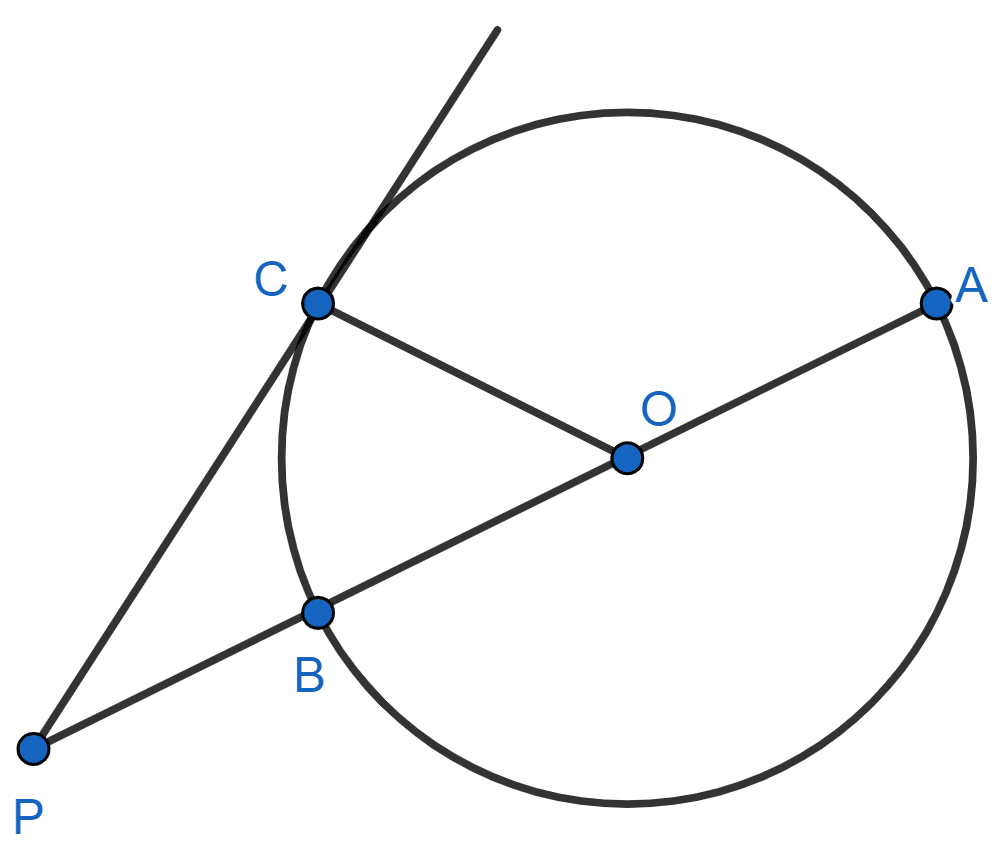
In the given figure, AB is diameter and PC is tangent to the circle with center O. If AP = 40 cm, CP = 20 cm, find the radius of the circle.