Mathematics
If E, F, G and H are mid-points of the sides AB, BC, CD and DA, respectively of a parallelogram ABCD, prove that area of the quad. EFGH = area of || gm ABCD.
Theorems on Area
Answer
Parallelogram ABCD with E, F, G and H as mid-points of the sides AB, BC, CD and DA, respectively is shown below:
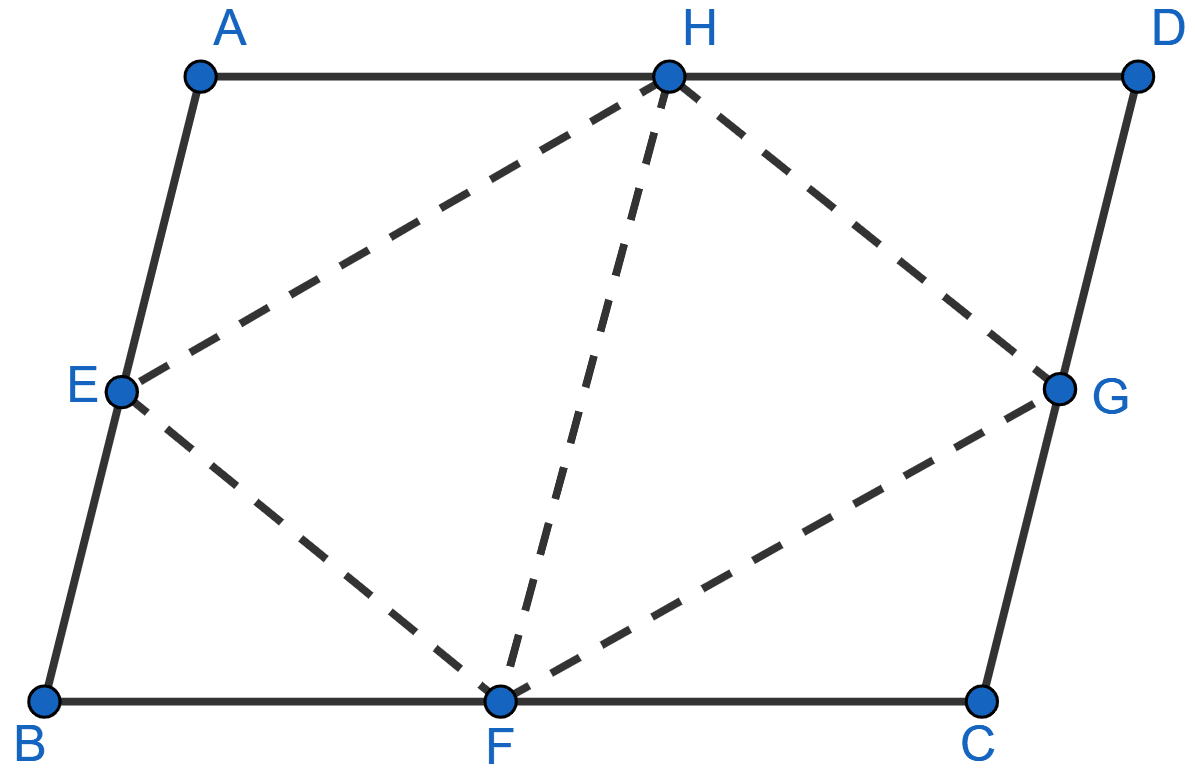
AH = AD (As H is the mid-point of AD)
BF = BC (As F is the mid-point of BC)
So, AH = BF and AH || BF (As AD || BC).
So, ABFH is a || gm.
Since, || gm ABFH and △EFH are on same base FH and between same parallel lines AB and FH so,
area of △EFH = area of || gm ABFH …….(i)
Similarly,
HD = AD (As H is the mid-point of AD)
FC = BC (As F is the mid-point of BC)
So, HD = FC and HD || FC (As AD || BC).
So, HFCD is a || gm.
Since, || gm HFCD and △HFG are on same base HF and between same parallel lines HF and DC so,
area of △HFG = area of || gm HFCD …….(ii)
Adding (i) and (ii) we get,
area of △EFH + area of △HFG = area of || gm ABFH + area of || gm HFCD
area of quad. EFGH = (area of || gm ABFH + area of || gm HFCD)
= (area of || gm ABCD).
Hence, proved that area of the quad. EFGH = area of || gm ABCD.
Answered By
Related Questions
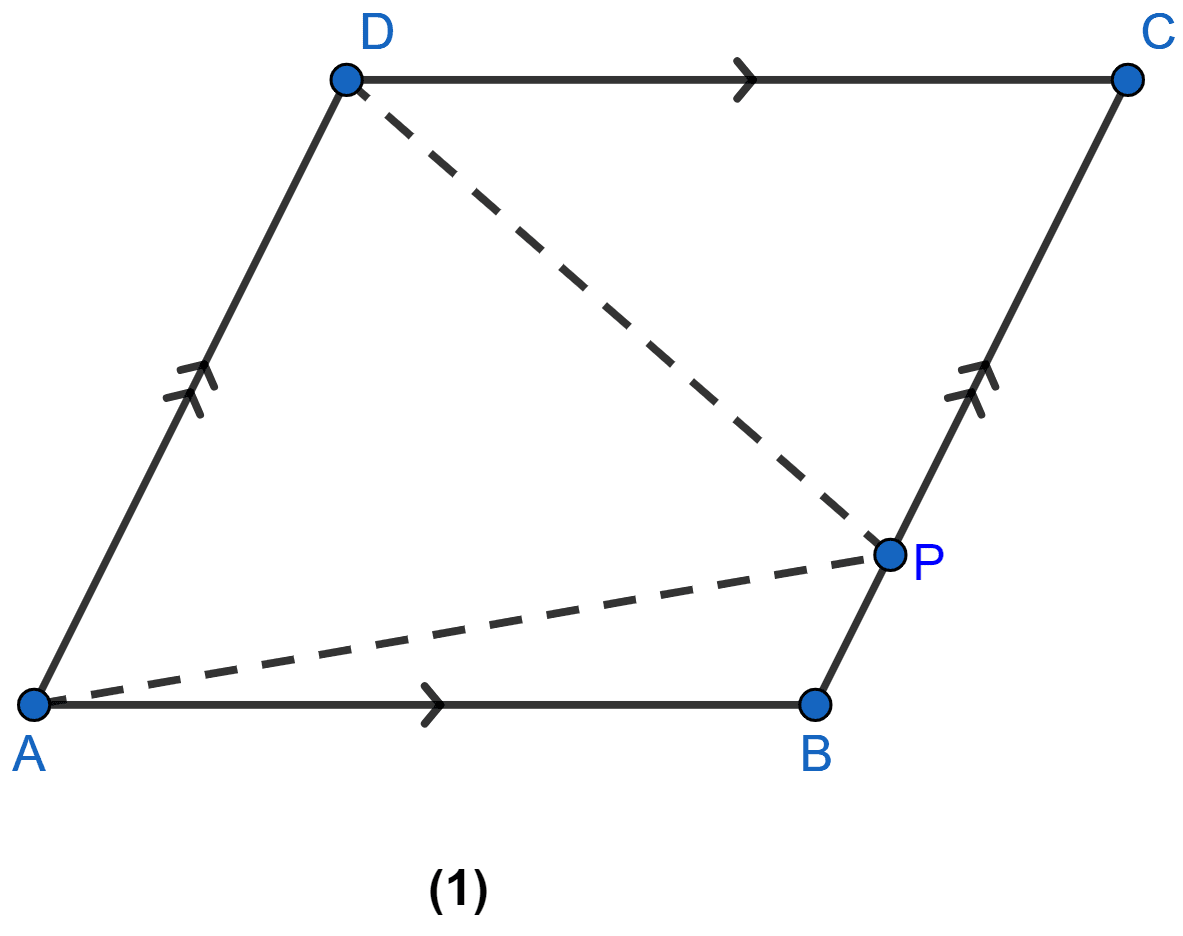
In figure (1) given below, ABCD is a parallelogram and P is any point in BC. Prove that, Area of ∆ABP + area of ∆DPC = Area of ∆APD.
In the figure (2) given below, O is any point inside a parallelogram ABCD. Prove that
(i) area of ∆OAB + area of ∆OCD = area of || gm ABCD.
(ii) area of ∆OBC + area of ∆OAD = area of || gm ABCD.

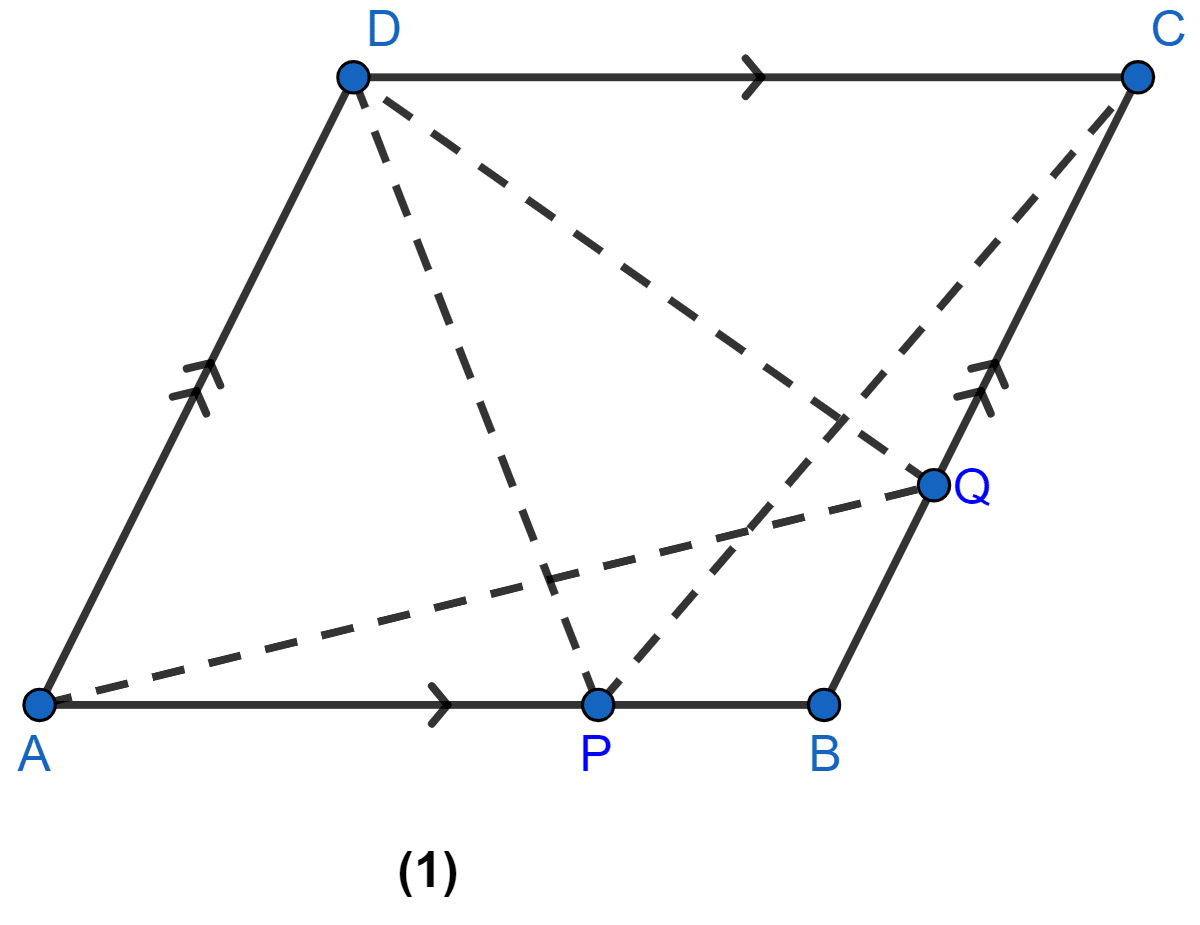
In figure (1) given below, ABCD is a parallelogram. P, Q are any two points on the sides AB and BC respectively. Prove that
area of ∆CPD = area of ∆AQD.
In the figure (2) given below, PQRS and ABRS are parallelograms, and X is any point on the side BR. Show that
area of ∆AXS = area of || gm PQRS.
