Mathematics
E is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that △ ABE ~ △ CFB.
Triangles
1 Like
Answer
Parallelogram ABCD is shown in the figure below:
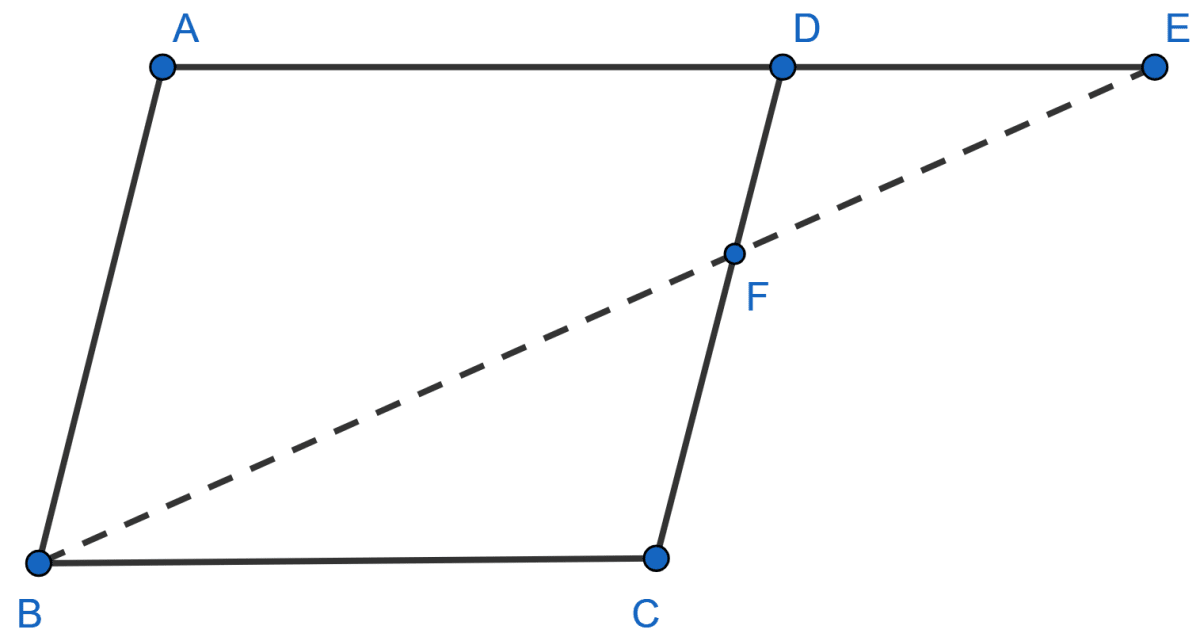
In Δ ABE and Δ CFB,
⇒ ∠BAE = ∠FCB (Opposite angles of a parallelogram are equal)
⇒ ∠AEB = ∠FBC [AE || BC and EB is a transversal, alternate interior angles]
∴ Δ ABE ~ Δ CFB (By A.A. axiom)
Hence, proved that △ ABE ~ △ CFB.
Answered By
1 Like
Related Questions
In given figure, if △ ABE ≅ Δ ACD, show that
△ ADE ∼ Δ ABC.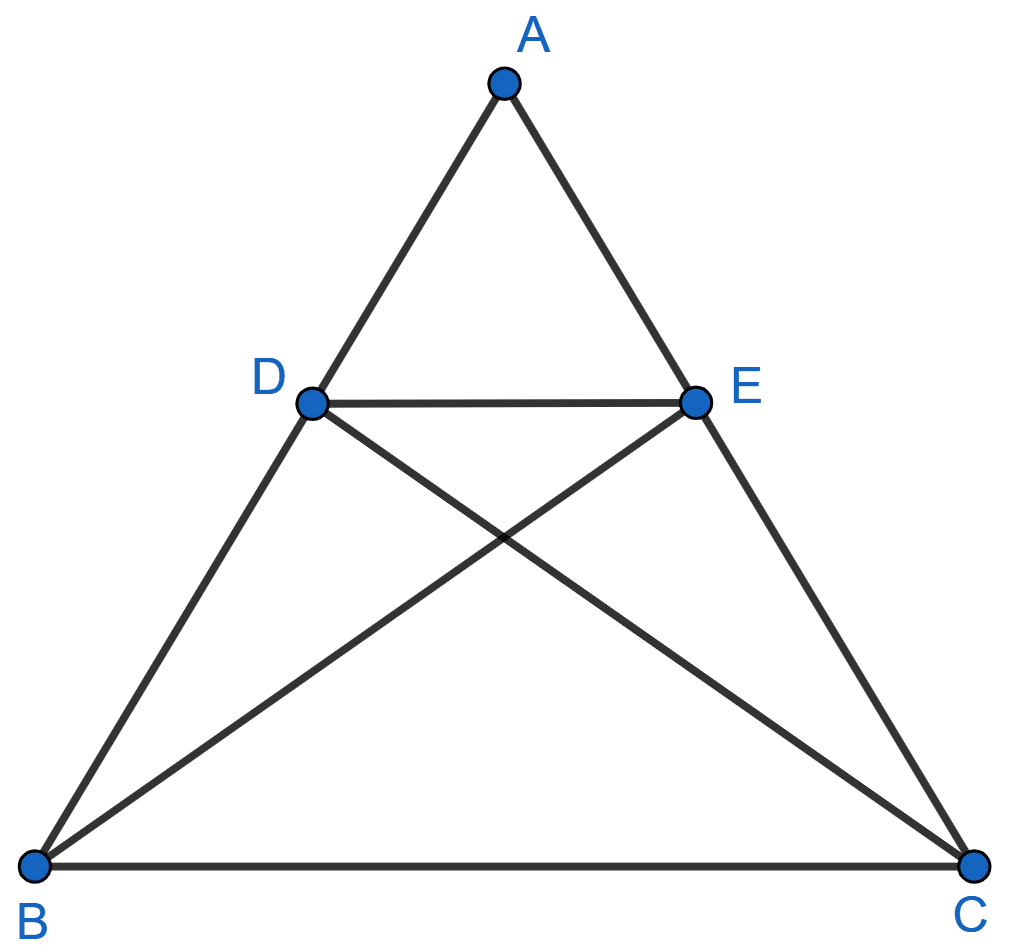
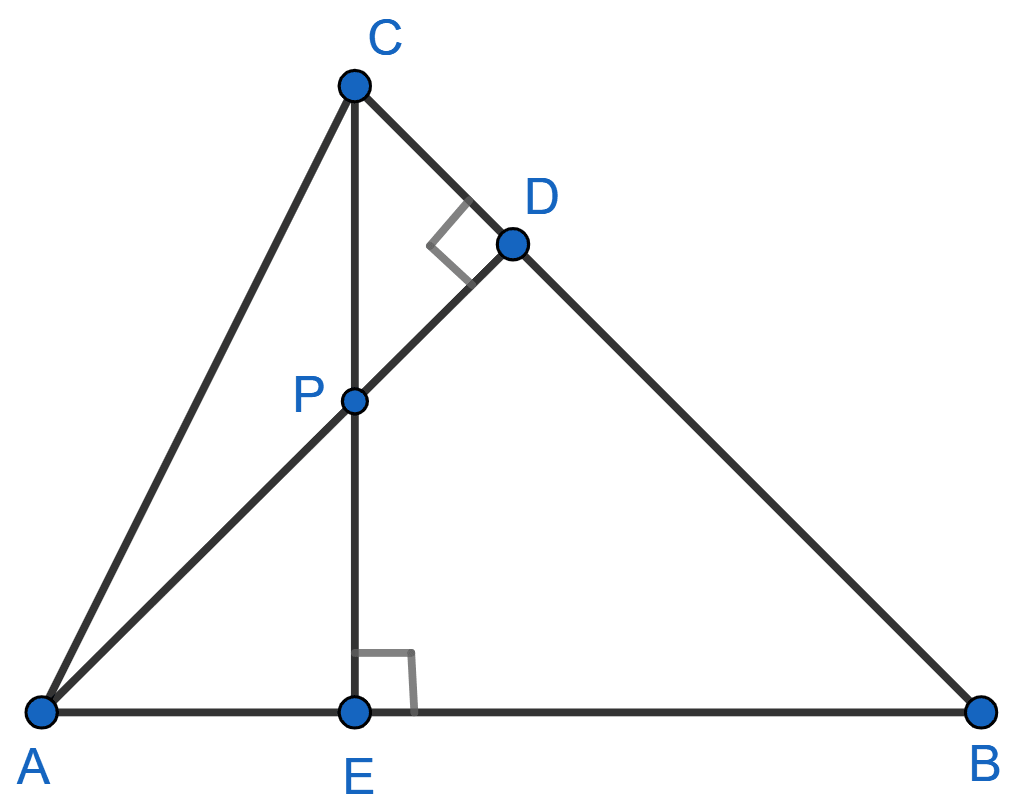
In the given figure, altitudes AD and CE of ∆ ABC intersect each other at the point P. Show that:
(i) ∆ AEP ~ ∆ CDP
(ii) ∆ ABD ~ ∆ CBE
(iii) ∆ AEP ~ ∆ ADB
(iv) ∆ PDC ~ ∆ BEC
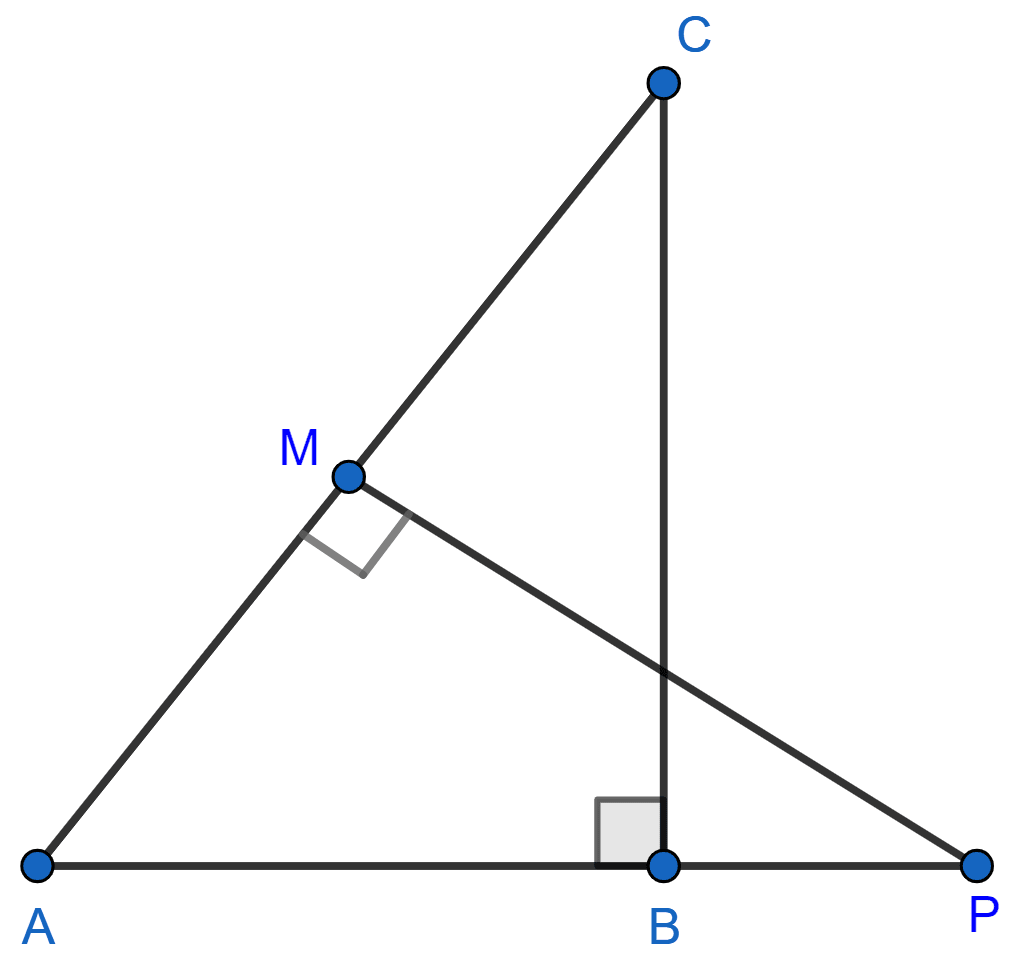
In the given figure, ABC and AMP are two right triangles, right angled at B and M respectively. Prove that :
(i) Δ ABC ~ Δ AMP
(ii)
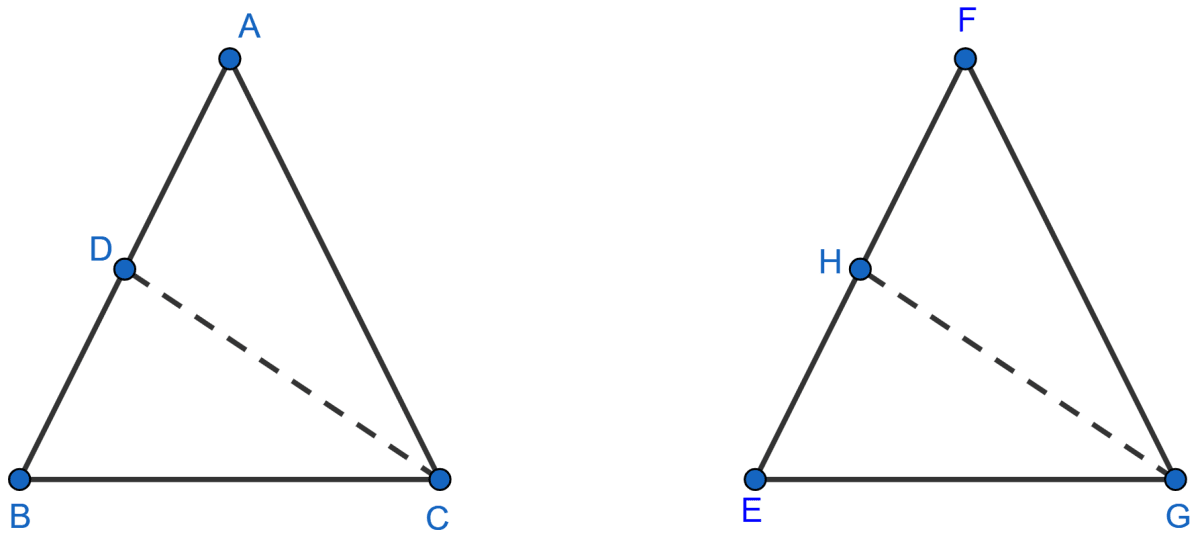
CD and GH are respectively the bisectors of Δ ACB and Δ EGF such that D and H lie on sides AB and FE of Δ ABC and Δ EFG respectively. If Δ ABC ~ Δ FEG, show that:
(i)
(ii) Δ DCB ~ Δ HGE
(iii) Δ DCA ~ Δ HGF