ABC is an isosceles triangle with AB = AC = 12 cm and BC = 8 cm. Find the altitude on BC and hence calculate its area.

Let AD be altitude on BC and BD = x cm and CD = (8 - x) cm.

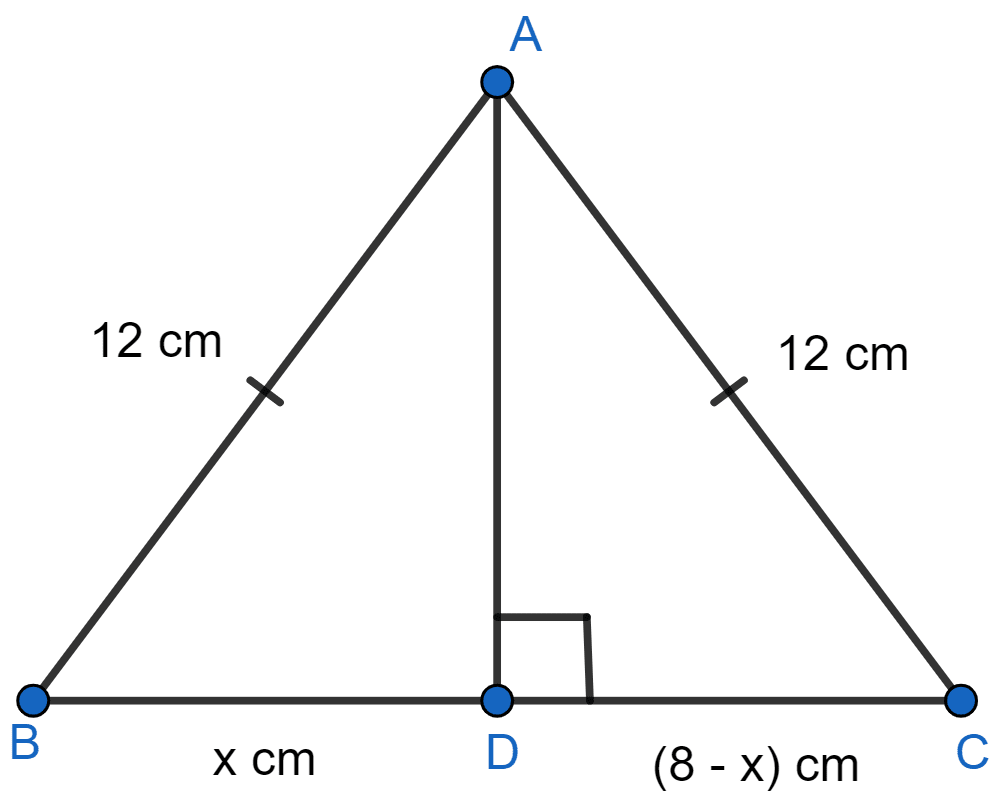
From figure,

In right angle △ABD,

By pythagoras theorem,

AB² = AD² + BD²

12² = AD² + x²

AD² = 12² - x² ……..(i)

In right angle △ADC,

By pythagoras theorem,

AC² = AD² + DC²

12² = AD² + (8 - x)²

AD² = 12² - (8 - x)² ……..(ii)

From (i) and (ii) we get,

12² - x² = 12² - (8 - x)²

144 - x² = 144 - (64 + x² - 16x)

144 - x² = 144 - 64 - x² + 16x

144 - 144 - x² + x² + 64 = 16x

16x = 64

x = 4.

Substituting value of x in (i) we get,

AD² = 12² - x² = 144 - (4)² = 144 - 16 = 128

AD² = 128

AD = $\sqrt{128} = 8\sqrt{2}$ cm.

Area = $\dfrac{1}{2} \times AD \times BC = \dfrac{1}{2} \times 8\sqrt{2} \times 8 = 32\sqrt{2}$ cm².

Hence, AD = $8\sqrt{2}$ cm and area = $32\sqrt{2}$ cm².