Mathematics
Three circles touch each other externally. A triangle is formed when the centers of these circles are joined together. Find the radii of the circles, if the sides of the triangle formed are 6 cm, 8 cm and 9 cm.
Circles
12 Likes
Answer
Let there be three circles with center A, B and C and ABC be the triangle formed when centres of 3 circles are joined.
Let,
AB = 6 cm, AC = 8 cm and BC = 9 cm.
Let the radii of the circles having centres A, B and C be r1, r2 and r3 respectively.
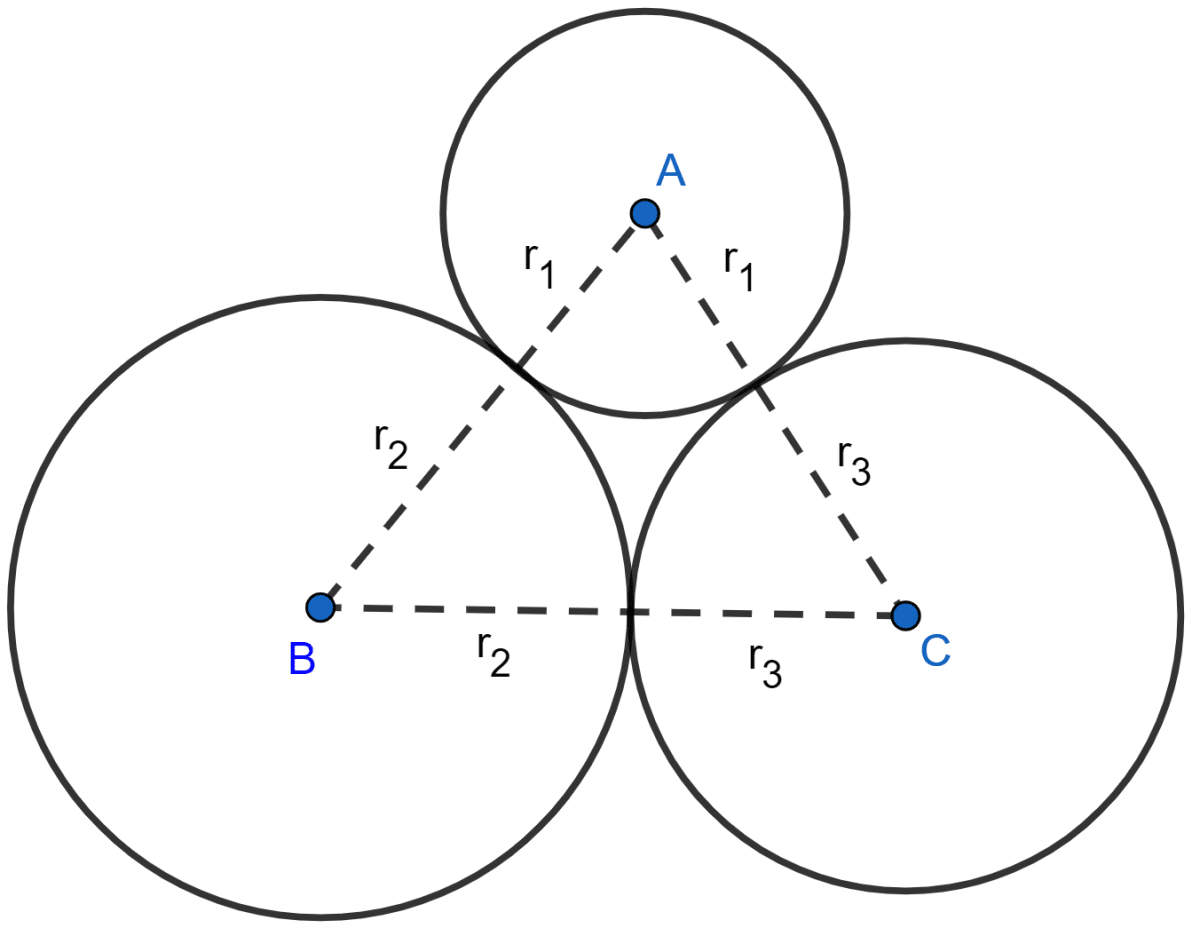
As,
⇒ AB = r1 + r2 = 6 …………(1)
⇒ AC = r1 + r3 = 8 …………(2)
⇒ BC = r2 + r3 = 9 ………….(3)
Adding all the above equations, we get
⇒ r1 + r2 + r1 + r3 + r2 + r3 = 6 + 8 + 9
⇒ 2(r1 + r2 + r3) = 23
⇒ r1 + r2 + r3 =
⇒ r1 + r2 + r3 = 11.5 cm ………..(4)
Substituting value of r2 + r3 = 9 in equation (4) we get :
⇒ r1 + 9 = 11.5
⇒ r1 = 11.5 - 9
⇒ r1 = 2.5 cm.
Substituting value of r1 + r2 = 6 in equation (4) we get :
⇒ 6 + r3 = 11.5
⇒ r3 = 11.5 - 6
⇒ r3 = 5.5 cm.
Substituting value of r1 + r3 = 8 in equation (4) we get :
⇒ 8 + r2 = 11.5
⇒ r2 = 11.5 - 8
⇒ r2 = 3.5 cm.
Hence, the radii of the circles are 2.5 cm, 3.5 cm and 5.5 cm.
Answered By
9 Likes
Related Questions
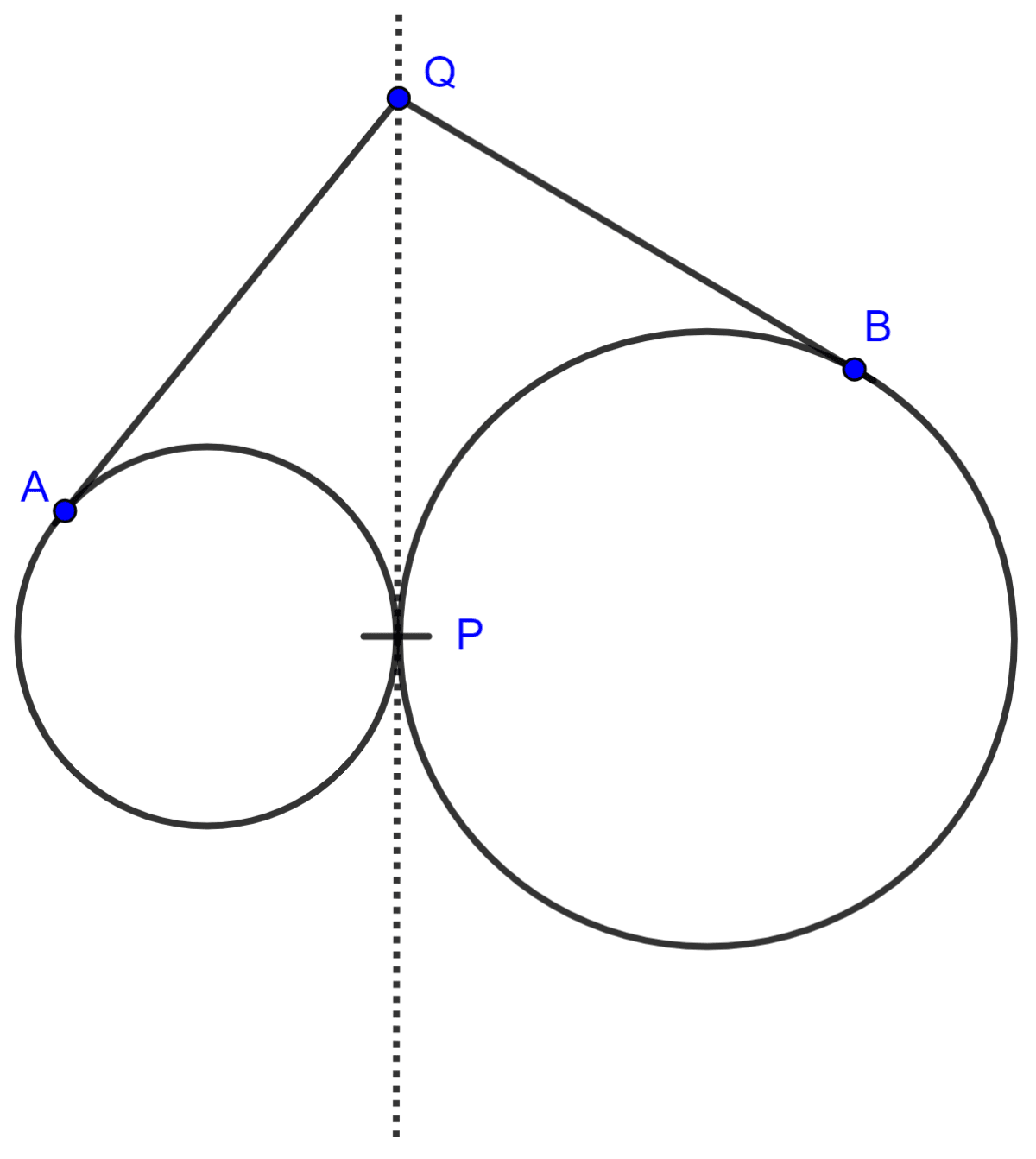
Two circles touch each other externally at point P. Q is a point on the common tangent through P. Prove that the tangents QA and QB are equal.
Two circles of radii 5 cm and 3 cm are concentric. Calculate the length of a chord of the outer circle which touches the inner.
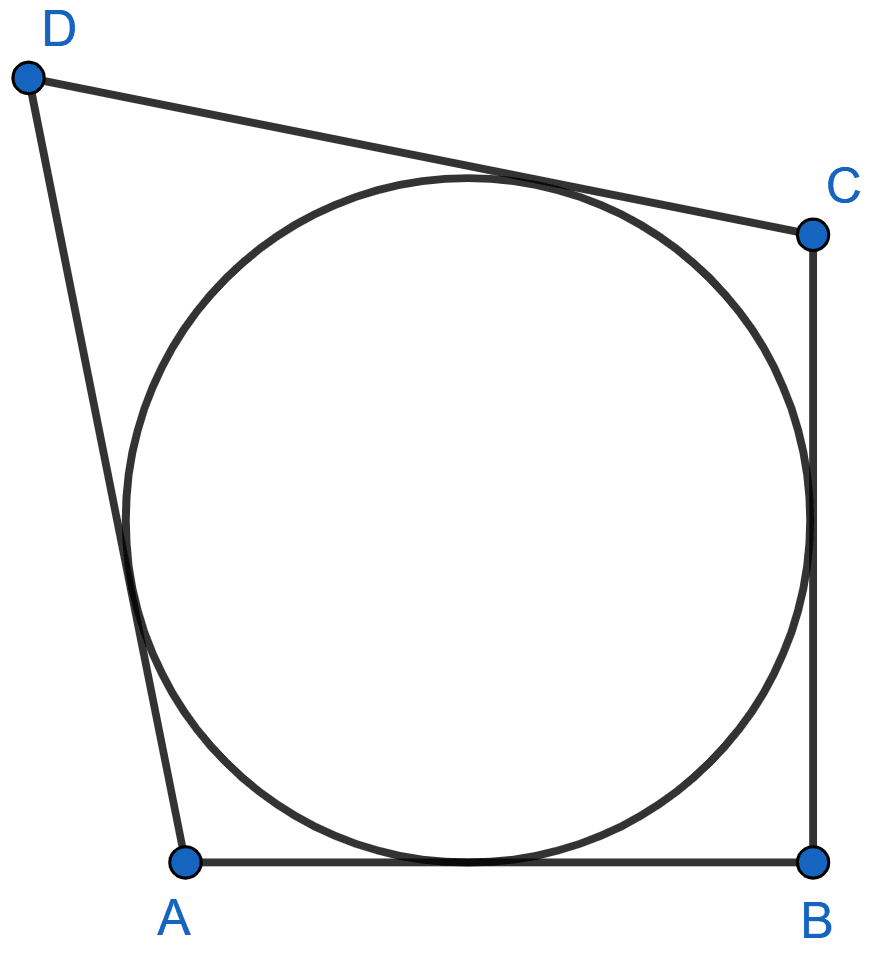
If the sides of a quadrilateral ABCD touch a circle, prove that AB + CD = BC + AD.
If the sides of a parallelogram touch a circle, prove that the parallelogram is a rhombus.