Mathematics
The sides of a right-angled triangle containing the right angle are 5x cm and (3x – 1) cm. Calculate the length of the hypotenuse of the triangle if its area is 60 cm2.
Mensuration
32 Likes
Answer
Consider △ABC as a right angled triangle.
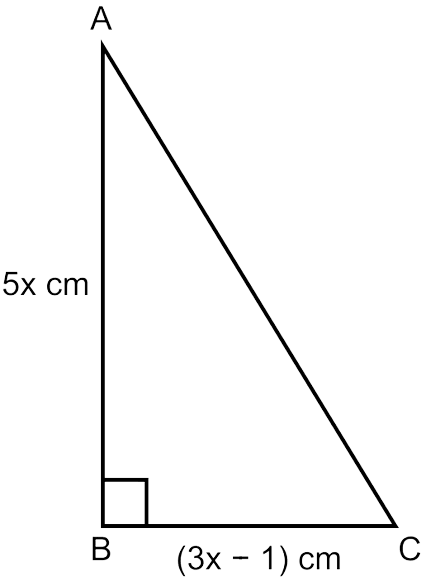
AB = 5x cm and BC = (3x – 1) cm
We know that,
Area of △ABC = × base × height = × BC × AB
Substituting the values we get,
⇒ 60 = × (3x – 1) × 5x
⇒ 120 = 5x(3x – 1)
⇒ 120 = 15x2 – 5x
⇒ 15x2 – 5x – 120 = 0
⇒ 5(3x2 – x – 24) = 0
⇒ 3x2 – x – 24 = 0
⇒ 3x2 – 9x + 8x – 24 = 0
⇒ 3x(x – 3) + 8(x - 3) = 0
⇒ (3x + 8)(x - 3) = 0
⇒ 3x + 8 = 0 or x - 3 = 0
⇒ 3x = -8 or x = 3
⇒ x = or x = 3
Since, x cannot be negative as length of a side cannot be negative. So, x = 3.
AB = 5 × 3 = 15 cm
BC = (3 × 3 – 1) = 9 – 1 = 8 cm
In right angled △ABC,
Using Pythagoras theorem,
⇒ AC2 = AB2 + BC2
Substituting the values we get,
⇒ AC2 = 152 + 82
⇒ AC2 = 225 + 64 = 289
⇒ AC2 = 172
So, AC = 17 cm.
Hence, the hypotenuse of the right angled triangle is 17 cm.
Answered By
9 Likes
Related Questions
Find the area of an isosceles triangle whose equal sides are 12 cm each and the perimeter is 30 cm.
Find the area of an isosceles triangle whose base is 6 cm and perimeter is 16 cm.
In △ABC, ∠B = 90°, AB = (2x + 1) cm and BC = (x + 1) cm. If the area of the △ABC is 60 cm2, find its perimeter.
If the perimeter of a right angled triangle is 60 cm and its hypotenuse is 25 cm, find its area.