Mathematics
The shadow of a vertical tower on a level ground increases by 10 m when the altitude of the sun changes from 45° to 30°. Find the height of the tower, correct to two decimal places.
Heights & Distances
51 Likes
Answer
Let the height of the tower BD be h metres and the length of its shadow be d metres when the sun's altitude is 45°. When the sun's altitude is 30°, then the length of shadow of tower is 10 m longer,
i.e., BD = h meters, AB = d meters and CA = 10 metres.
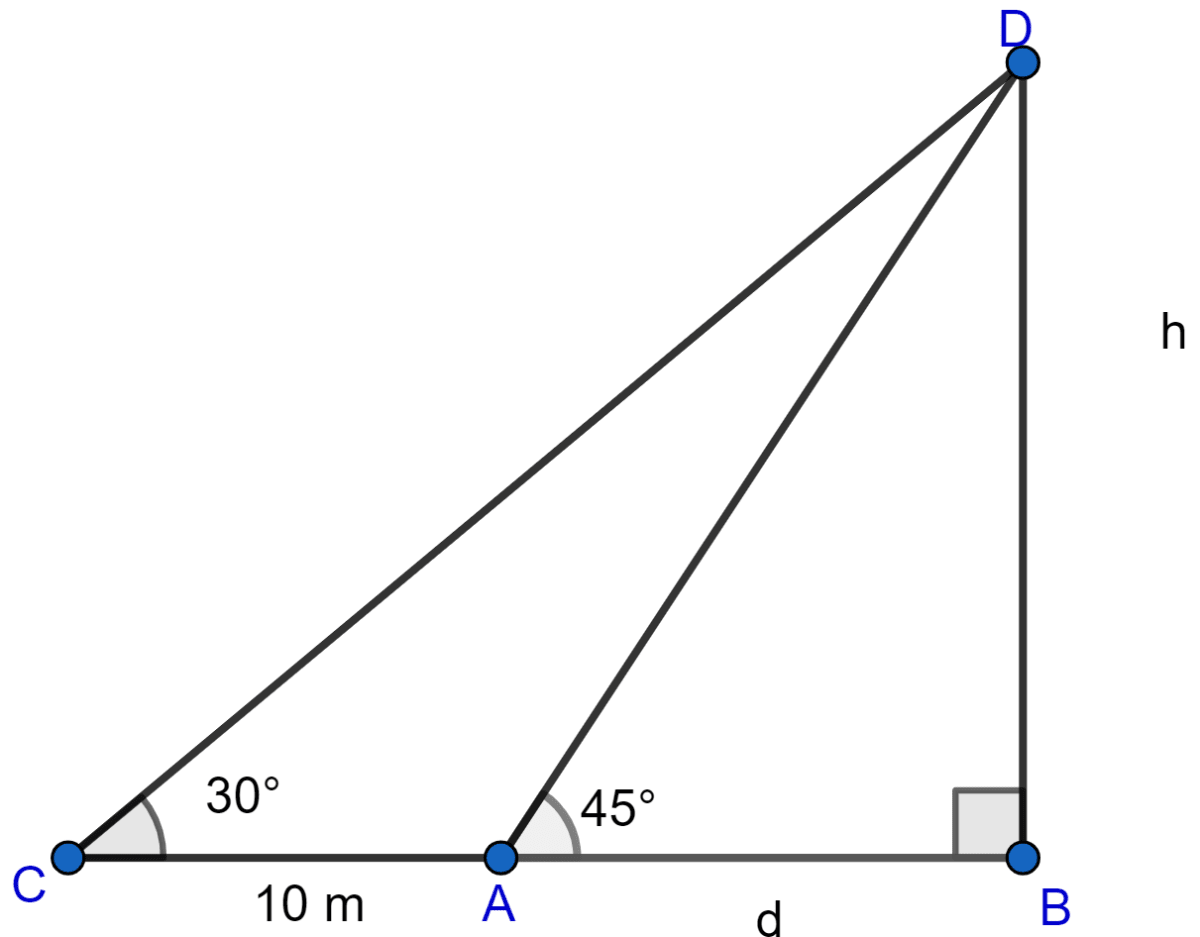
From right angled △ABD, we get
From right angled △BCD, we get
Hence, the height of the tower is 13.66 meters.
Answered By
27 Likes
Related Questions
A man observes the angle of elevation of the top of a tower to be 45°. He walks towards it in a horizontal line through its base. On covering 20 m, the angle of elevation changes to be 60°. Find the height of the tower correct to 2 significant figures.
A man observes the angle of elevation of the top of a building to be 30°. He walks towards it in a horizontal line through its base. On covering 60 m, the angle of elevation changes to 60°. Find the height of the building correct to the nearest metre.
An aeroplane when flying at a height of 3125 m from the ground passes vertically below another plane at an instant when the angles of elevation of the two planes from the same point on the ground are 30° and 60° respectively. Find the distance between the two planes at the instant.
From the top of a hill, the angles of depression of two consecutive kilometer stones, due east are found to be 30° and 45° respectively. Find the distance of two stones from the foot of the hill.