From the top of a hill, the angles of depression of two consecutive kilometer stones, due east are found to be 30° and 45° respectively. Find the distance of two stones from the foot of the hill.

Let R be the top of the tower and Q the foot. P and T be two consecutive kilometer stones with depression angles 30° and 45° respectively.

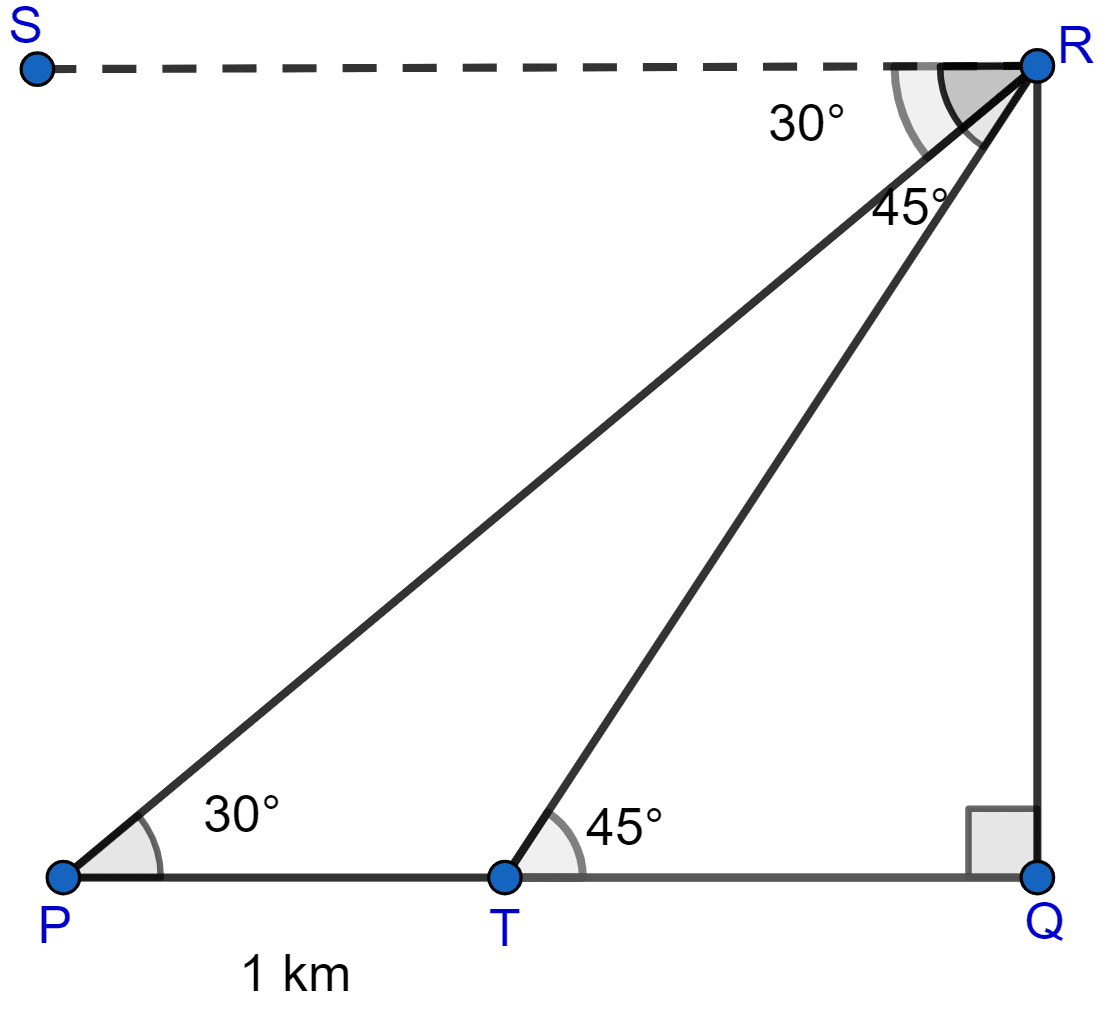
Since stones are consecutive kilometer stones hence distance between them = 1 km.

From figure,

∠RPQ = ∠SRP = 30°      (Alternate angles are equal)
∠RTQ = ∠SRT = 45°      (Alternate angles are equal)
PT = 1 km
TQ = PQ - PT = PQ - 1      (Eq 1)

From right angled △PQR, we get

$\Rightarrow \text{tan 30°} = \dfrac{QR}{PQ} \\[1em] \Rightarrow \dfrac{1}{\sqrt{3}} = \dfrac{QR}{PQ} \\[1em] \Rightarrow PQ = \sqrt{3} \text{ QR} \\[1em] \Rightarrow QR = \dfrac{PQ}{\sqrt{3}}\text{ ……(Eq 2)}$

From right angled △TQR, we get

$\Rightarrow \text{tan 45°} = \dfrac{QR}{TQ} \\[1em] \Rightarrow 1 = \dfrac{QR}{TQ} \\[1em] \Rightarrow TQ = QR \\[1em] \Rightarrow PQ - 1 = QR \text{ (Using Eq 1)}\\[1em] \Rightarrow PQ - 1 = \dfrac{PQ}{\sqrt{3}} \text{ (Using Eq 2)}\\[1em] \Rightarrow \sqrt{3}PQ - \sqrt{3} = PQ \\[1em] \Rightarrow \sqrt{3}PQ - PQ = \sqrt{3} \\[1em] \Rightarrow 0.732PQ = 1.732 \\[1em] \Rightarrow PQ = \dfrac{1.732}{0.732} \\[1em] \Rightarrow PQ = 2.366$

Using Eq 1,

TQ = PQ - 1 = 2.366 - 1 = 1.366.

Hence, the distance of two stones from hill are 1.366 km and 2.366 km.