Mathematics
The probability of selecting a blue marble and a red marble from a bag containing red, blue and green marbles is and respectively. If the bag contains 14 green marbles, then find :
(a) number of red marbles.
(b) total number of marbles in the bag.
Probability
49 Likes
Answer
As the bag contains red, blue and green marbles.
∴ Probability of selecting a red marble + Probability of selecting a blue marble + Probability of selecting a green marble = 1
⇒ + Probability of selecting a green marble = 1
⇒ + Probability of selecting a green marble = 1
⇒ Probability of selecting a green marble =
⇒ Probability of selecting a green marble =
⇒ Probability of selecting a green marble = .
(a) Given,
⇒ Probability of selecting a red marble = .
Hence, no. of red marbles = 6.
(b) Hence, total no. of marbles in the bag = 30.
Answered By
29 Likes
Related Questions
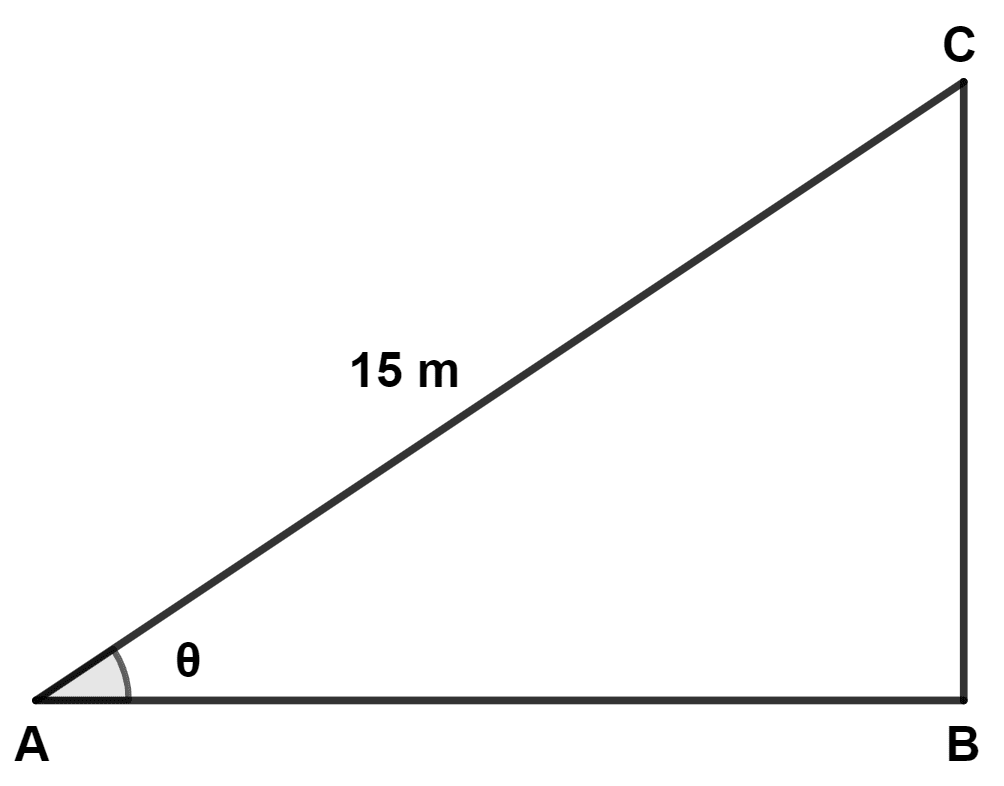
An inclined plane AC is prepared with its base AB which is √3 times its vertical height BC. The length of the inclined plane is 15 m. Find:
(a) value of θ.
(b) length of its base AB, in nearest metre.
Prove that :
tan2 θ + cos2 θ - 1 = tan2 θ. sin2 θ
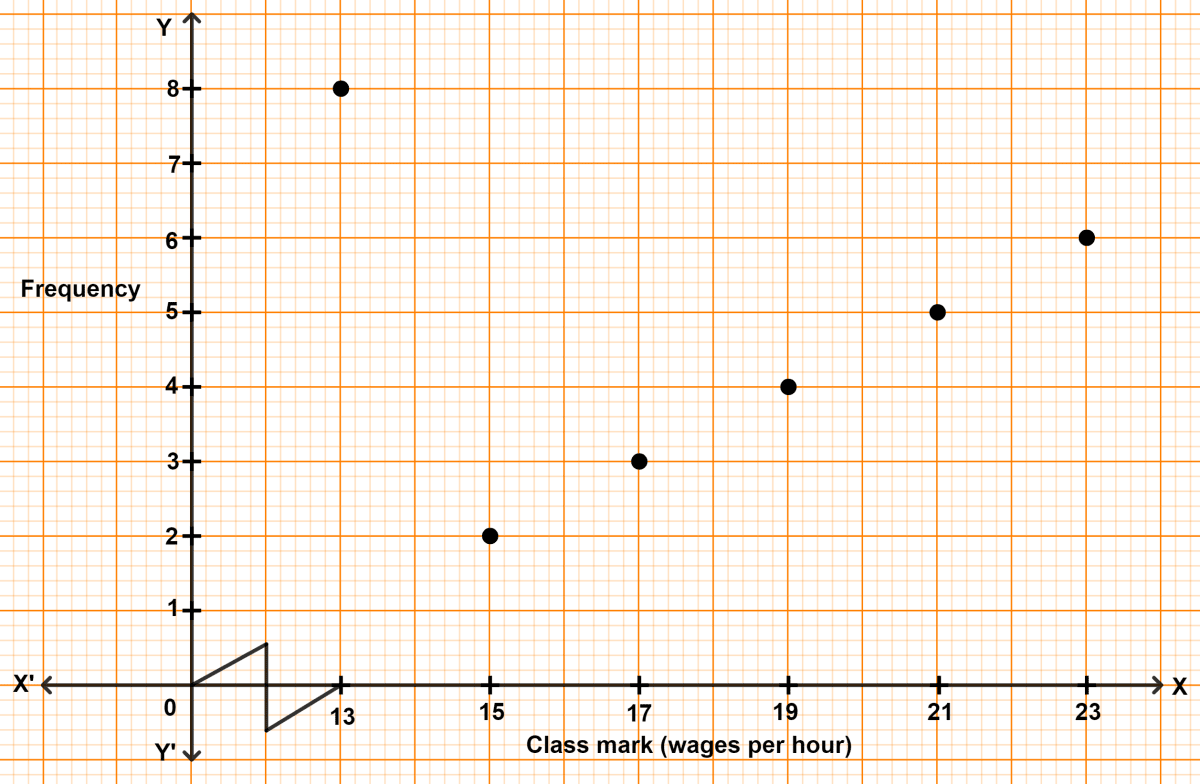
The class mark and frequency of a data is given in the graph. From the graph, Find:
(a) the table showing the class interval and frequency.
(b) the mean
The mean of 5, 7, 8, 4 and m is n and the mean of 5, 7, 8, 4, m and n is m. Find the values of m and n.