Mathematics
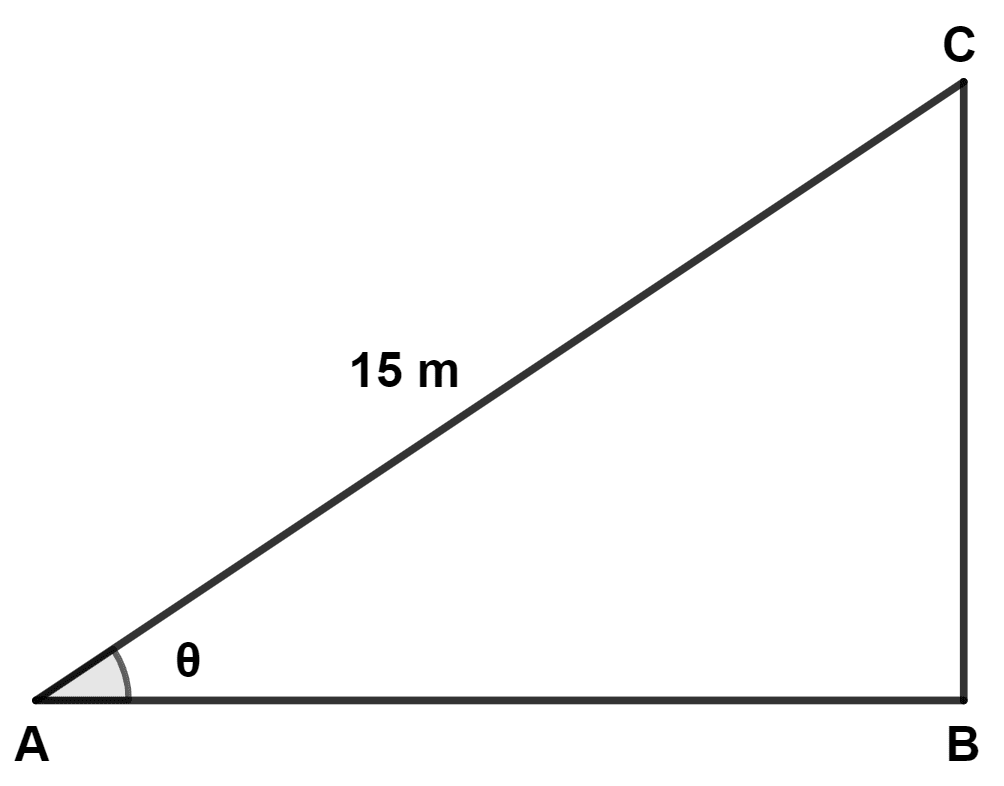
An inclined plane AC is prepared with its base AB which is √3 times its vertical height BC. The length of the inclined plane is 15 m. Find:
(a) value of θ.
(b) length of its base AB, in nearest metre.
Heights & Distances
43 Likes
Answer
(a) According to question,
⇒ AB =
⇒ AB =
⇒ BC =
From figure,
⇒ tan θ =
⇒ tan θ =
⇒ tan θ =
⇒ tan θ = tan 30°
⇒ θ = 30°.
Hence, θ = 30°.
(b) In right angle triangle ABC,
By pythagoras theorem,
⇒ AC2 = BC2 + AB2
⇒ 152 = + AB2
⇒ 225 = + AB2
⇒ 225 =
⇒ 225 =
⇒ AB2 =
⇒ AB2 =
⇒ AB2 = 168.75
⇒ AB =
⇒ AB = 12.99 m
Rounding off,
AB = 13 m.
Hence, AB = 13 m.
Answered By
29 Likes
Related Questions
A famous sweet shop “Madanlal Sweets” sells tinned rasgullas. The tin container is cylindrical in shape with diameter 14 cm, height 16 cm, and it can hold 20 spherical rasgullas of diameter 6 cm and sweetened liquid such that the can is filled and then sealed. Find out how much sweetened liquid the can contains. Take π = 3.14.
The ratio of the radius and the height of a solid metallic right circular cylinder is 7 : 27. This is melted and made into a cone of diameter 14 cm and slant height 25 cm. Find the height of the :
(a) cone
(b) cylinder
Prove that :
tan2 θ + cos2 θ - 1 = tan2 θ. sin2 θ
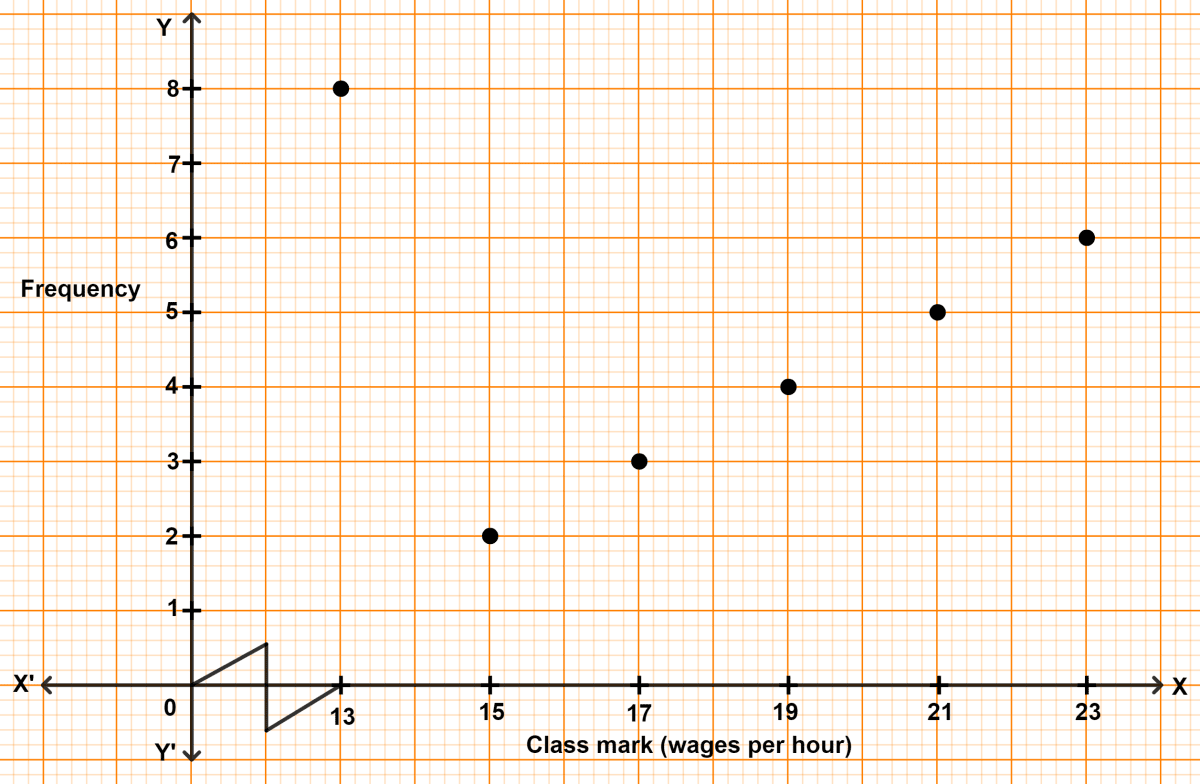
The class mark and frequency of a data is given in the graph. From the graph, Find:
(a) the table showing the class interval and frequency.
(b) the mean