Mathematics
The horizontal distance between two towers is 75 m and the angular depression of the top of the first tower as seen from the top of the second, which is 160 m high, is 45°. Find the height of the first tower.
Heights & Distances
7 Likes
Answer
Let AB be the first tower and CD be the second tower and ∠EDA = 45° is the angle of depression.
Given, angle of depression of the top of the first tower as seen from the top of the second tower is 45°.
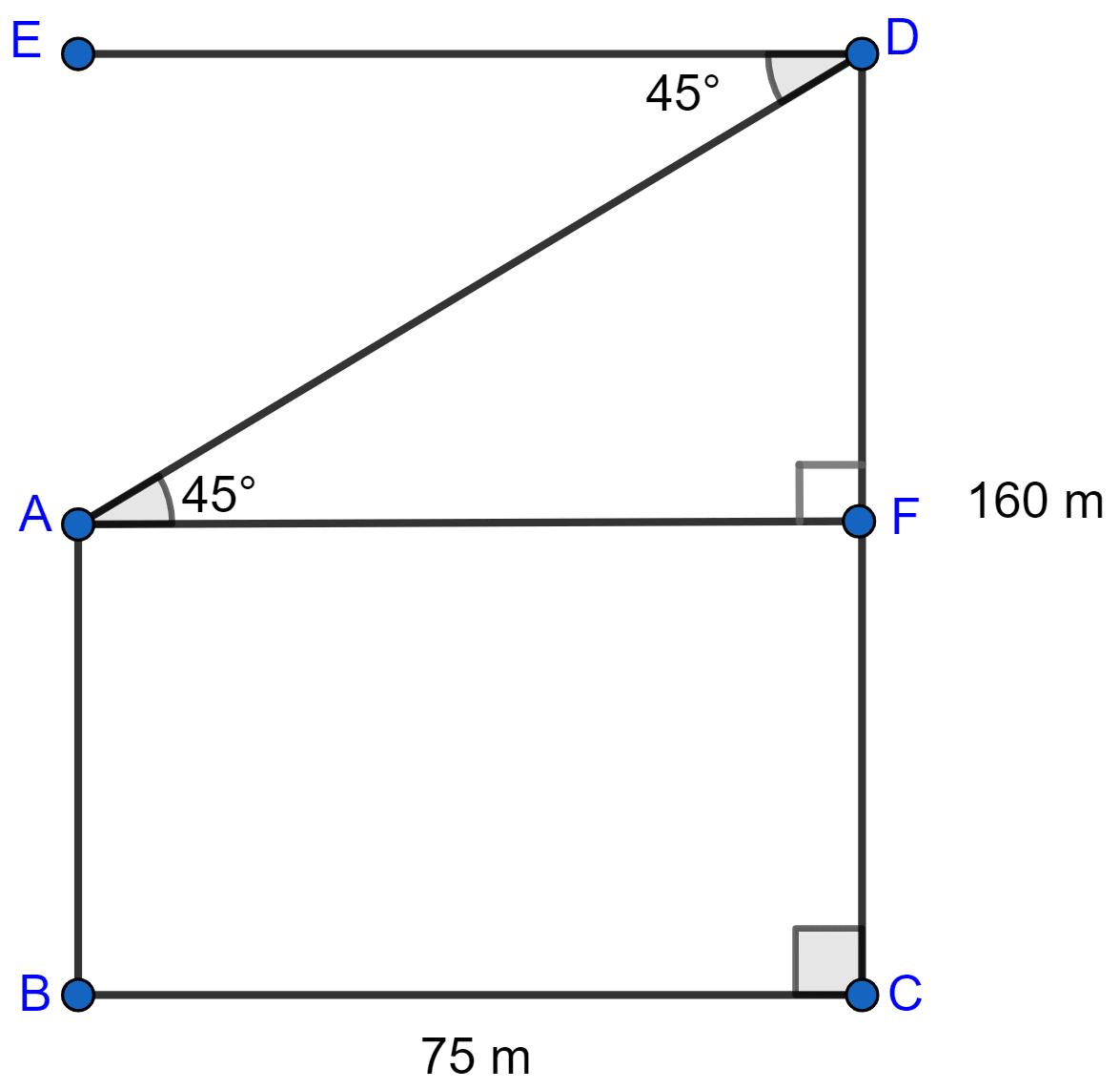
We know that,
Alternate angles are equal.
∴ ∠DAF = ∠EDA = 45°.
From figure,
AF = BC = 75 m.
In △ADF,
From figure,
AB = FC = CD - DF = 160 - 75 = 85 m.
Hence, height of first tower = 85 m.
Answered By
3 Likes
Related Questions
The length of the shadow of a tower standing on level plane is found to be 2y meters longer when the sun's altitude is 30° than when it was 45°. Prove that the height of the tower is meters.
A person standing on the bank of a river observes that the angle of elevation of the top of a tree standing on the opposite bank is 60°. When he moves 40 m away from the bank, he finds the angle of elevation to be 30°. Find :
(i) the height of the tree, correct to 2 decimal places.
(ii) the width of the river.
A man in a boat rowing away from a lighthouse 150 m high, takes 2 minutes to change the angle of elevation of the top of the lighthouse from 60° to 45°. Find the speed of the boat.
An aeroplane flying horizontally 1 km above the ground and going away from the observer is observed at an elevation of 60°. After 10 seconds, its elevation is observed to be 30°; find the uniform speed of the aeroplane in km per hour.