Mathematics
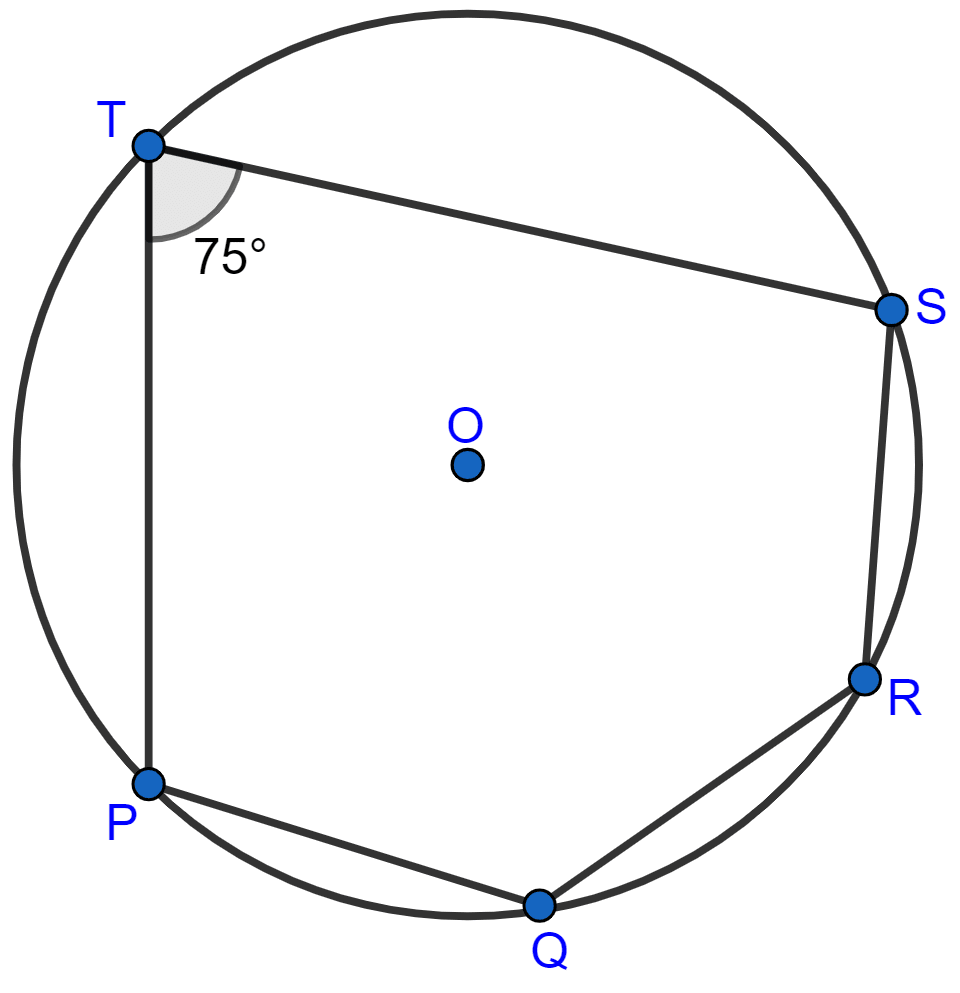
The given figure show a circle with centre O. Also, PQ = QR = RS and ∠PTS = 75°.
Calculate:
(i) ∠POS,
(ii) ∠QOR,
(iii) ∠PQR.
Circles
10 Likes
Answer
Join OP, OQ, OR and OS.
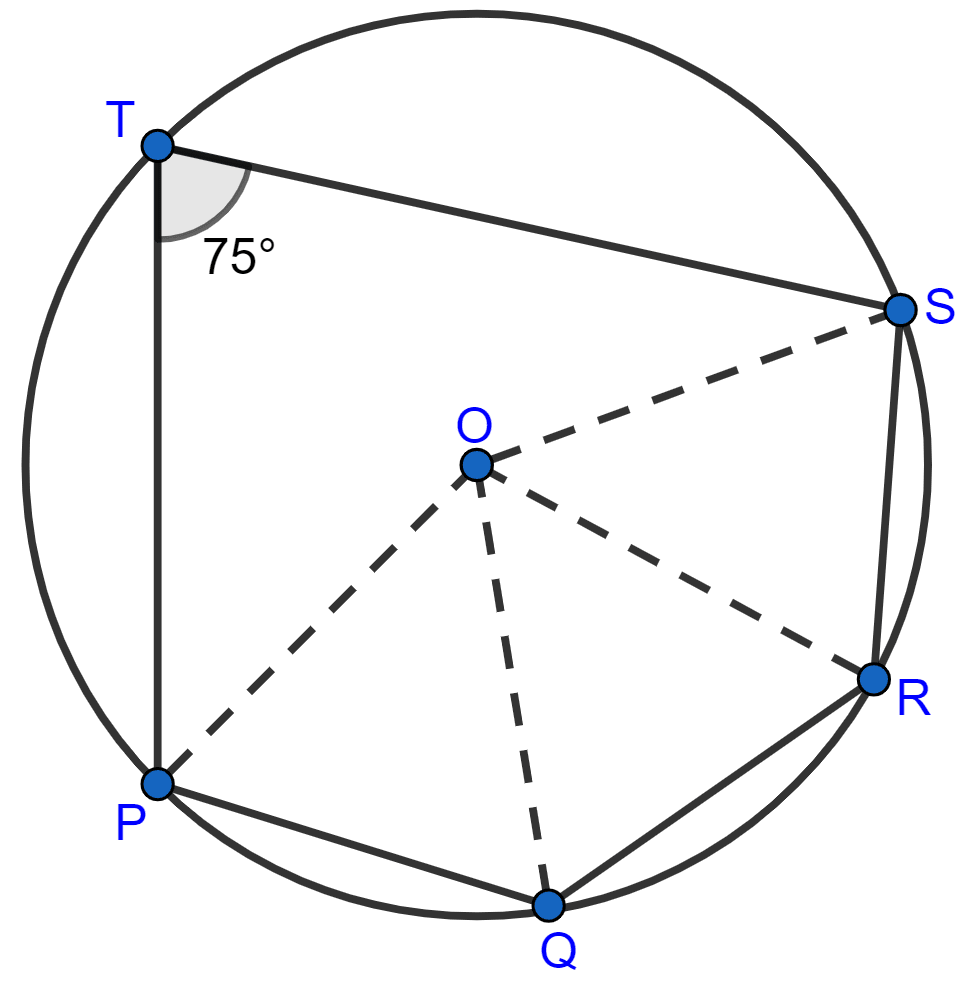
Given, PQ = QR = RS
∴ ∠POQ = ∠QOR = ∠ROS = x (let) [Equal chords subtends equal angles at the centre]
Arc PQRS subtends ∠POS at the centre and ∠PTS at the circumference of the circle.
Thus,
∠POS = 2 x ∠PTS = 2 x 75° = 150° [As angle subtended at the centre by the arc is double that it subtends at any point on the circumference of the circle.]
⇒ ∠POQ + ∠QOR + ∠ROS = 150°
⇒ x + x + x = 150°
⇒ 3x = 150°
⇒ x = = 50°.
In ∆OPQ we have,
⇒ OP = OQ [Radii of the same circle]
⇒ ∠OPQ = ∠OQP = y (let) [Angles opposite to equal sides are equal]
In ∆OPQ,
⇒ ∠OPQ + ∠OQP + ∠POQ = 180°
⇒ ∠OPQ + ∠OQP + 50° = 180°
⇒ ∠OPQ + ∠OQP = 180° - 50°
⇒ ∠OPQ + ∠OQP = 130°
⇒ 2y = 130°
⇒ y = = 65°
⇒ ∠OPQ = ∠OQP = y = 65°.
In ∆OQR we have,
⇒ OQ = OR [Radii of the same circle]
⇒ ∠OQR = ∠ORQ = z (let) [Angles opposite to equal sides are equal]
In ∆OQR
⇒ ∠OQR + ∠ORQ + ∠QOR = 180°
⇒ z + z + 50° = 180°
⇒ 2z = 180° - 50°
⇒ 2z = 130°
⇒ z = = 65°
⇒ ∠OQR = ∠ORQ = z = 65°.
(i) Hence, ∠POS = 150°.
(ii) Hence, ∠QOR = 50°.
(iii) From figure,
∠PQR = ∠PQO + ∠OQR = 65° + 65° = 130°.
Hence, ∠PQR = 130°.
Answered By
7 Likes
Related Questions
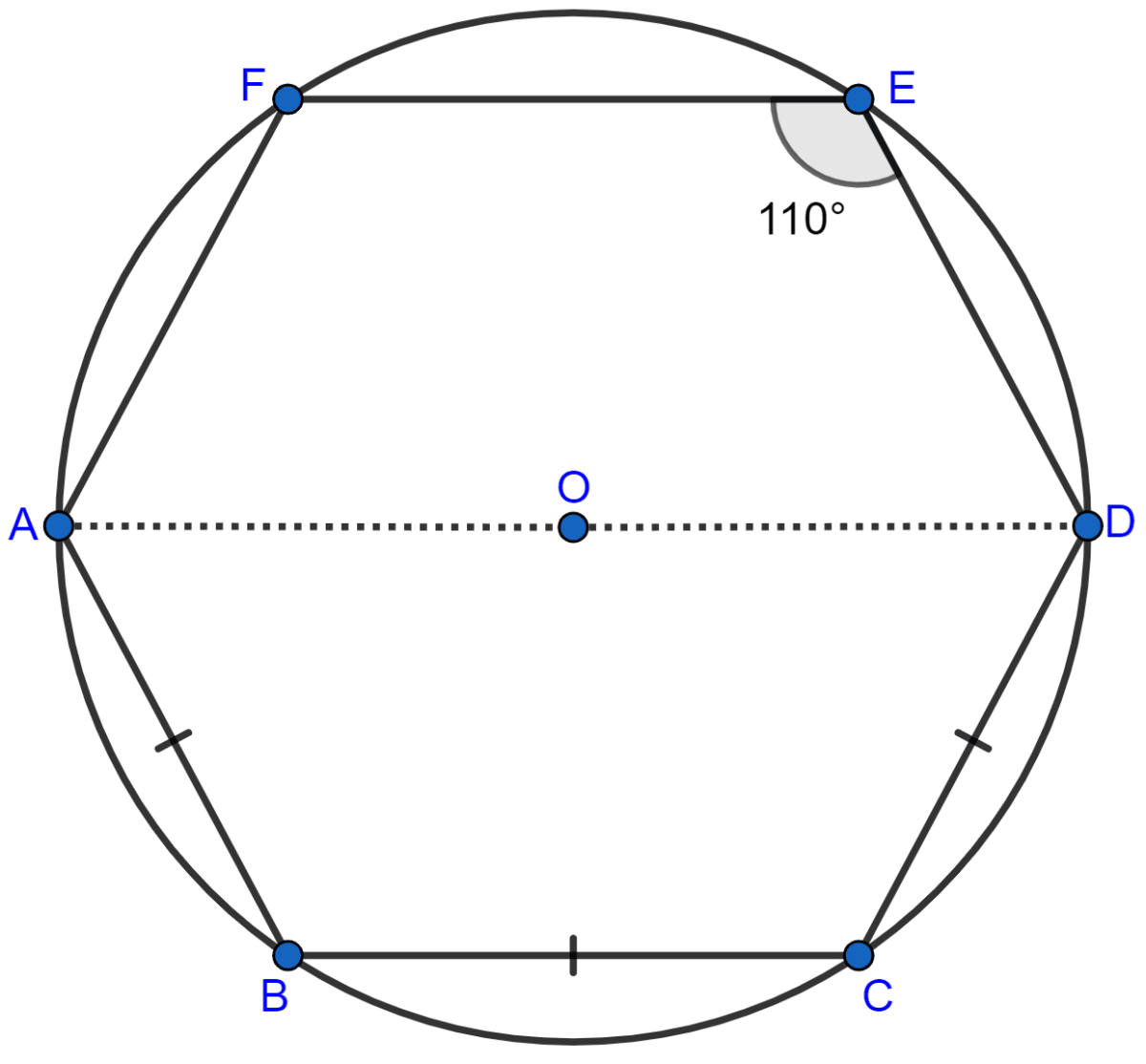
In the following figure, AD is the diameter of the circle with centre O. Chords AB, BC and CD are equal. If ∠DEF = 110°, calculate :
(i) ∠AEF, (ii) ∠FAB.
If two sides of a cyclic-quadrilateral are parallel; prove that :
(i) its other two sides are equal.
(ii) its diagonals are equal.
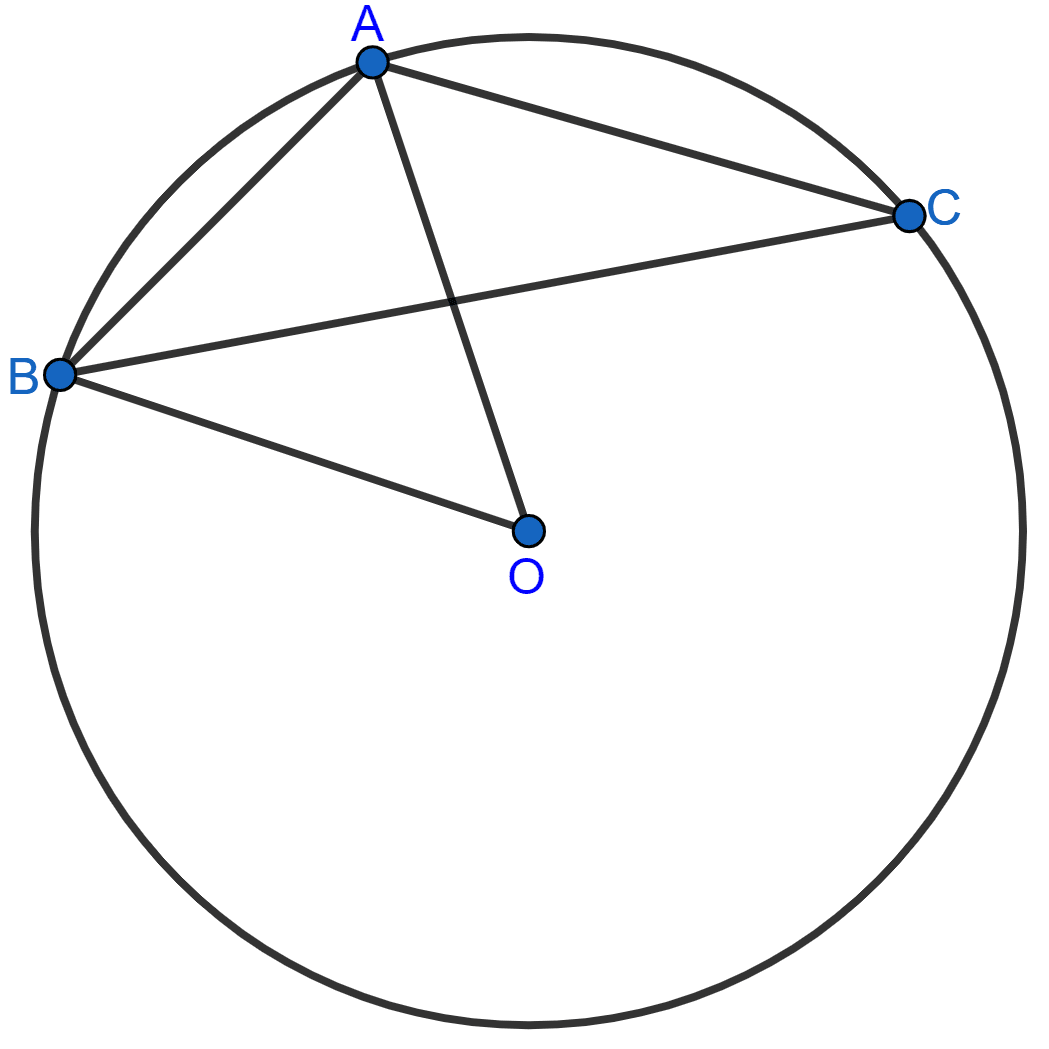
In the given figure, AB is a side of a regular six-sided polygon and AC is a side of a regular eight-sided polygon inscribed in the circle with centre O. Calculate the sizes of :
(i) ∠AOB,
(ii) ∠ACB,
(iii) ∠ABC.
In a regular pentagon ABCDE, inscribed in a circle; find the ratio between angle EDA and angle ADC.