Mathematics
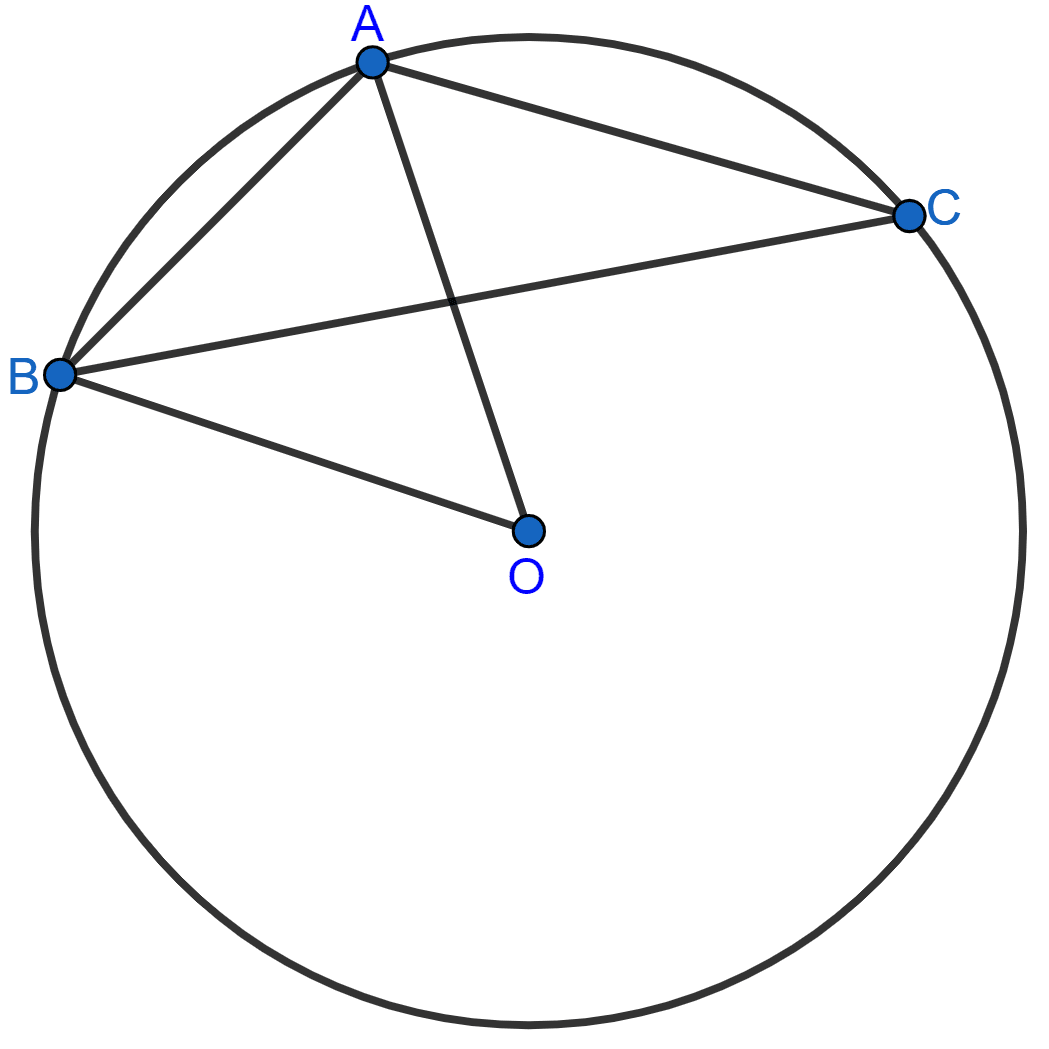
In the given figure, AB is a side of a regular six-sided polygon and AC is a side of a regular eight-sided polygon inscribed in the circle with centre O. Calculate the sizes of :
(i) ∠AOB,
(ii) ∠ACB,
(iii) ∠ABC.
Circles
10 Likes
Answer
Join OC.
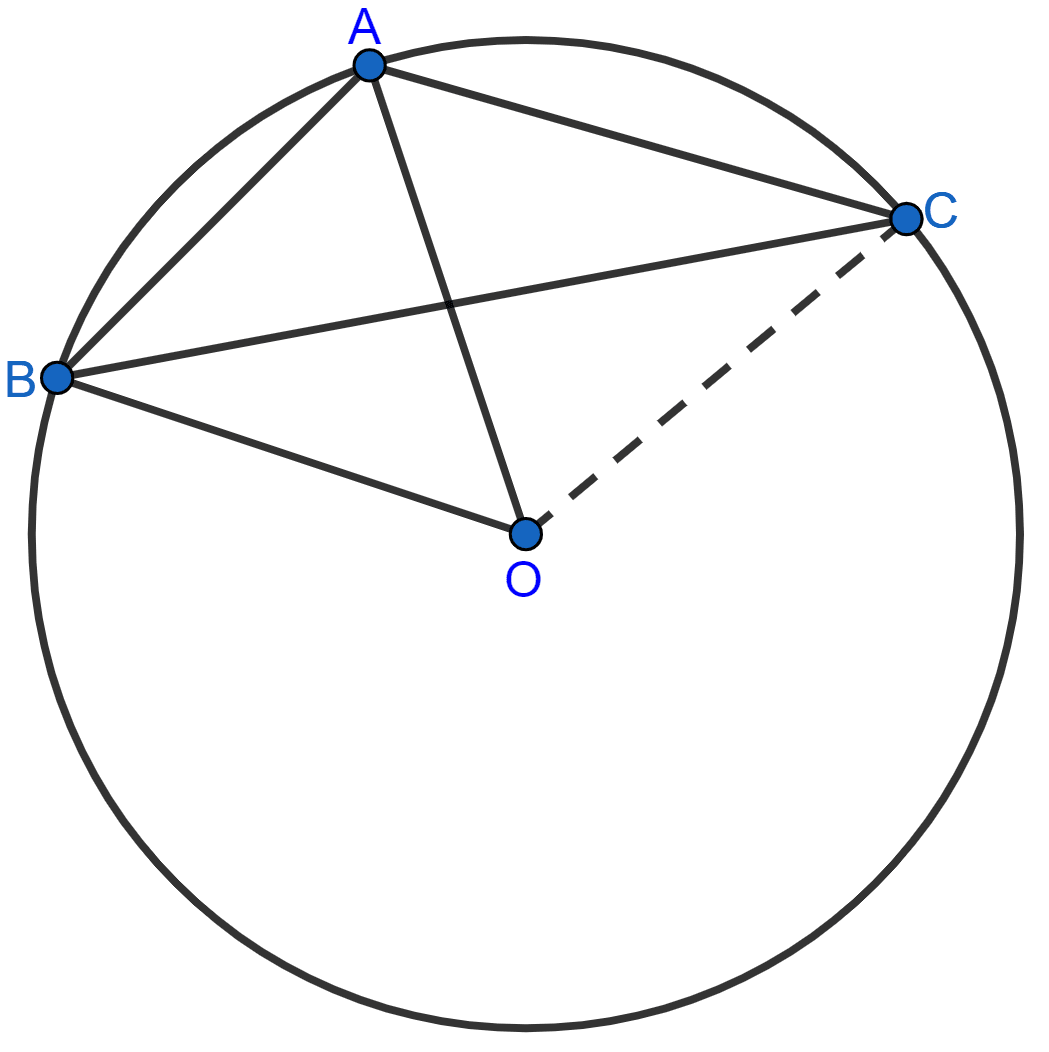
(i) We know that,
Each side of a regular hexagon, inscribed in a circle subtends an angle of 60° at the centre.
⇒ ∠AOB = 60°.
Hence, ∠AOB = 60°.
(ii) We know that,
Angle at the centre is twice the angle at remaining circumference.
∴ ∠AOB = 2∠ACB
⇒ ∠ACB = ∠AOB = = 30°.
Hence, ∠ACB = 30°.
(iii) Since AC is the side of a regular octagon,
∠AOC = = 45°.
Again, arc AC subtends ∠AOC at the centre and ∠ABC at the remaining part of the circle.
∴ ∠AOC = 2∠ABC
∴ ∠ABC = x ∠AOC = x 45° = 22.5°
Hence, ∠ABC = 22.5°.
Answered By
4 Likes
Related Questions
If two sides of a cyclic-quadrilateral are parallel; prove that :
(i) its other two sides are equal.
(ii) its diagonals are equal.
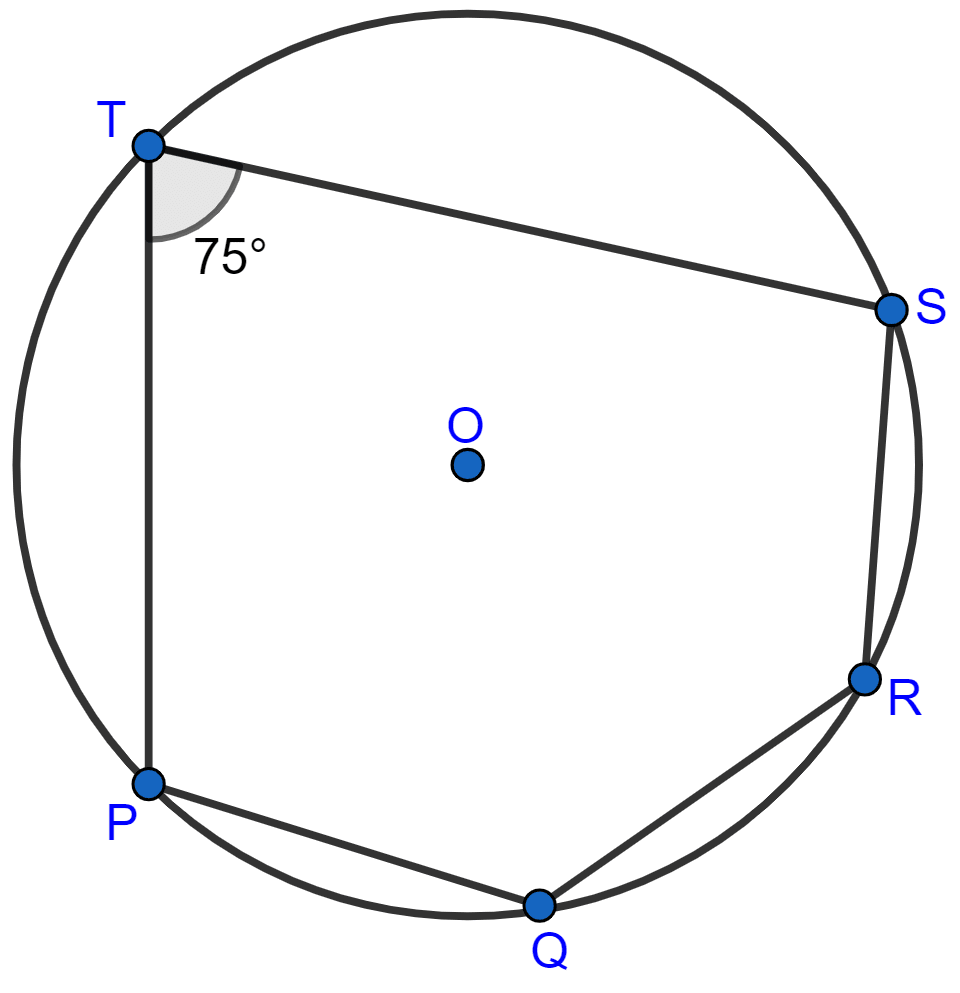
The given figure show a circle with centre O. Also, PQ = QR = RS and ∠PTS = 75°.
Calculate:
(i) ∠POS,
(ii) ∠QOR,
(iii) ∠PQR.
In a regular pentagon ABCDE, inscribed in a circle; find the ratio between angle EDA and angle ADC.
In the given figure, AB = BC = CD and ∠ABC = 132°. Calculate :
(i) ∠AEB,
(ii) ∠AED,
(iii) ∠COD.
