Mathematics
The given figure represents the lines y = x + 1 and y = Write down the angles which the lines make with the positive direction of the x-axis. Hence, determine θ.
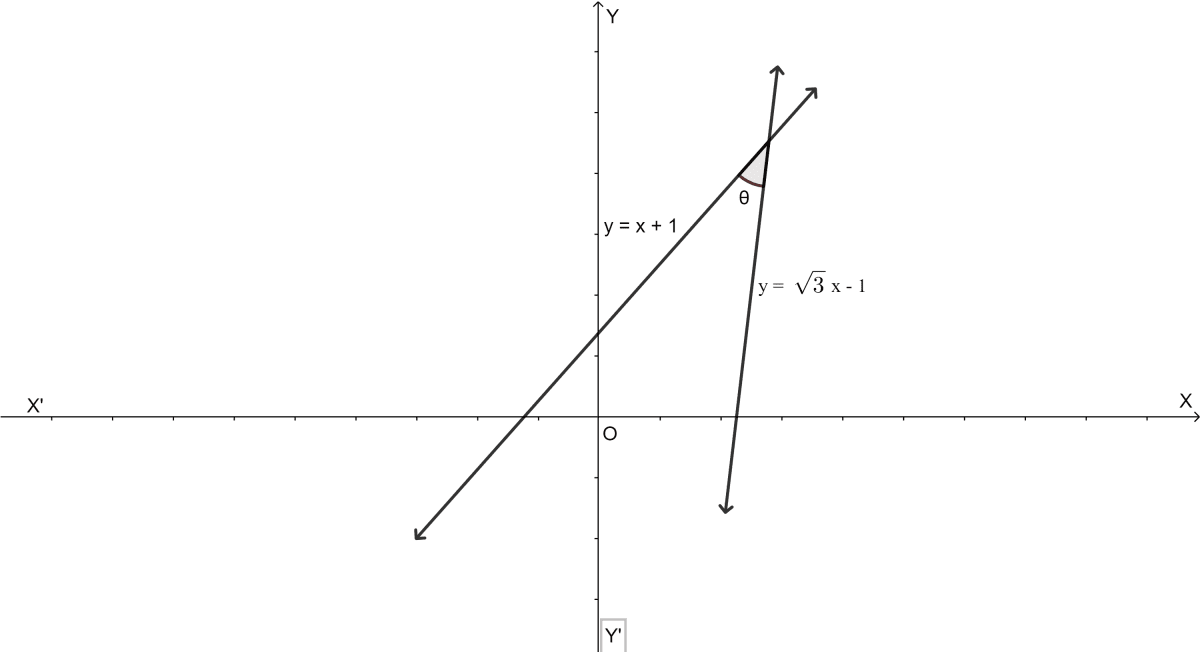
Straight Line Eq
69 Likes
Answer
Given,
y = x + 1 and y = .
Comparing equations with y = mx + c we get,
m1 = 1 and m2 = .
Let the first line make angle θ1 and second make θ2 with positive direction of x-axis.
The inclination that y = x + 1 makes is,
⇒ m1 = tan θ1 = 1
⇒ tan θ1 = 1 = tan 45°
⇒ tan θ1 = tan 45°
⇒ θ1 = 45°.
The inclination that y = - 1 makes is,
⇒ m2 = tan θ2 =
⇒ tan θ2 = = tan 60°
⇒ tan θ2 = tan 60°
⇒ θ2 = 60°.
From graph we get,
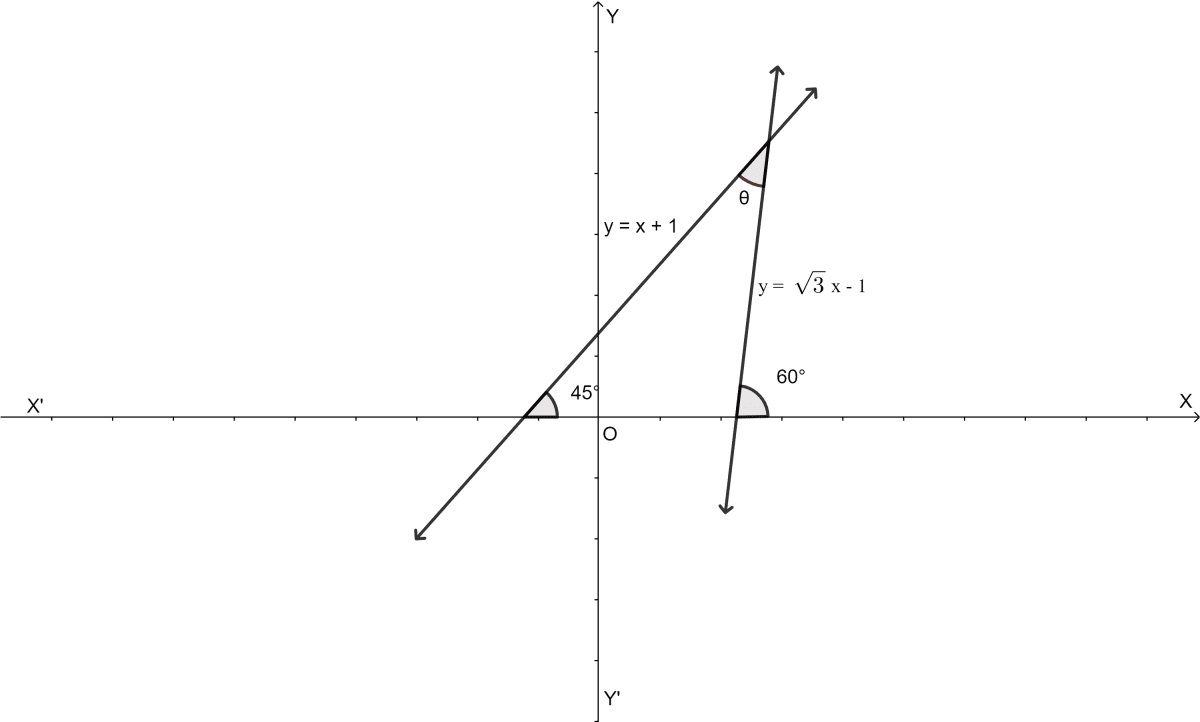
60° is the exterior angle. We know that,
Exterior angle = Sum of two opposite interior angles.
∴ 60° = θ + 45°
⇒ θ = 60° - 45°
⇒ θ = 15°
Hence, y = x + 1 makes 45° and y = makes 60° with the x-axis. The value of θ = 15°.
Answered By
32 Likes
Related Questions
Find the slope and y-intercept of the following lines :
(i) x - 2y - 1 = 0
(ii) 4x - 5y - 9 = 0
(iii) 3x + 5y + 7 = 0
(iv)
(v) y - 3 = 0
(vi) x - 3 = 0
The equation of the line PQ is 3y - 3x + 7 = 0.
(i) Write down the slope of the line PQ.
(ii) Calculate the angle that the line PQ makes with the positive direction of x-axis.
Find the value of p, given that the line passes through the point (-4, 4).
Given that (a, 2a) lies on the line , find the value of a.