KnowledgeBoat Logo

Mathematics

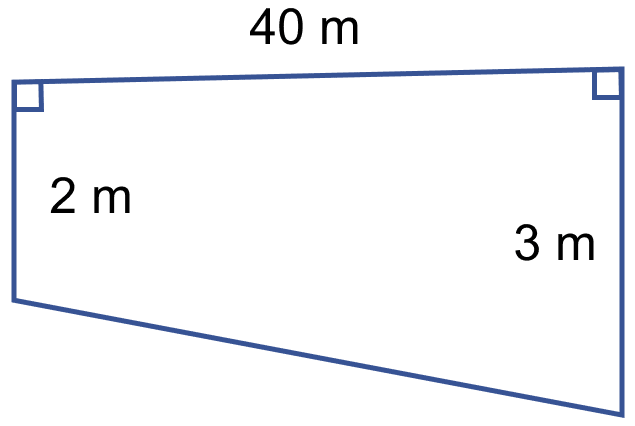
The figure (iii) given below show the cross-section of a swimming pool 10 m broad, 2 m deep at one end and 3 m deep at the other end. Calculate the volume of water it will hold when full, given that its length is 40 m.

The figure show the cross-section of a swimming pool 10 m broad, 2 m deep at one end and 3 m deep at the other end. Calculate the volume of water it will hold when full, given that its length is 40 m. Mensuration, ML Aggarwal Understanding Mathematics Solutions ICSE Class 9.

Mensuration

25 Likes

Answer

Figure (iii) is a trapezium with parallel sides 2 m and 3m.

By formula,

Area of cross section = 12×\dfrac{1}{2} \times (sum of parallel sides) × distance between them

= 12×\dfrac{1}{2} \times (2 + 3) × 40

= 12\dfrac{1}{2} × 5 × 40

= 100 m2.

So, the volume of water it will hold when full = area of cross section × width

= 100 × 10

= 1000 m3.

Hence, swimming pool will hold 1000 m3 of water.

Answered By

14 Likes

Related Questions