Mathematics
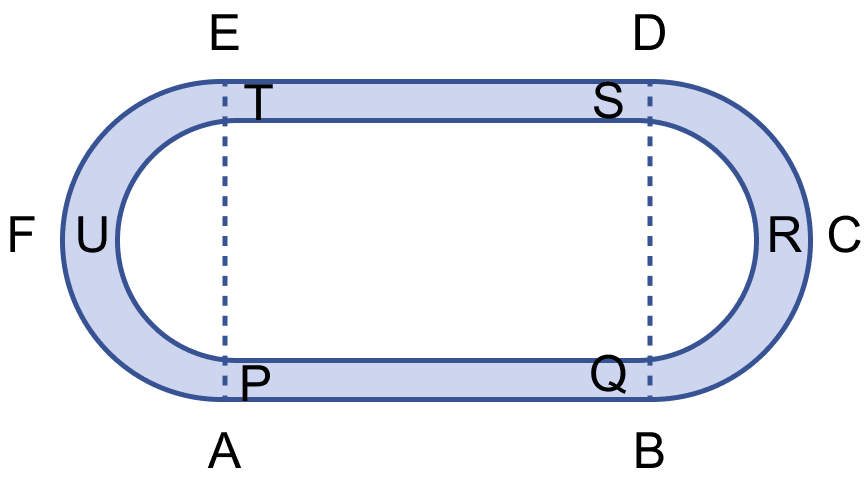
The figure (i) given below shows a running track surrounding a grassed enclosure PQRSTU. The enclosure consists of a rectangle PQST with a semicircular region at each end. PQ = 200 m; PT = 70 m.
(i) Calculate the area of the grassed enclosure in m2.
(ii) Given that the track is of constant width 7 m, calculate the outer perimeter ABCDEF of the track.
Mensuration
19 Likes
Answer
Given,
PQ = 200 m and PT = 70 m.
(i) By formula,
Area of rectangle PQST = l × b
= 200 × 70
= 14000 m2.
Radius of each semi-circular part on either side of rectangle = = 35 m.
Area of both semi-circular parts = 2 × = πr2
=
= 22 × 5 × 35
= 3850 m2.
So, the total area of grassed enclosure = 14000 + 3850 = 17850 m2.
Hence, area of glassed enclosure = 17850 m2.
(ii) Given,
Width of track around the enclosure = 7 m.
From figure,
AB = PQ = 200 m
ED = ST = 200 m
EA = PT + ET + AP = 70 + 7 + 7 = 84 m
BD = DS + QS + BQ = 70 + 7 + 7 = 84 m
Outer radius of semi-circle (R) = = 42 m.
Circumference of both semi-circular part = πR + πR = 2πR.
=
= 264 m.
From figure,
Outer perimeter = Circumference of both semi-circular part + ED + AB
= 264 + 200 + 200
= 664 m.
Hence, perimeter of outer track ABCDEF = 664 m.
Answered By
10 Likes
Related Questions
A circular field has perimeter 660 m. A plot in the shape of a square having its vertices on the circumference is marked in the field. Calculate the area of the square field.
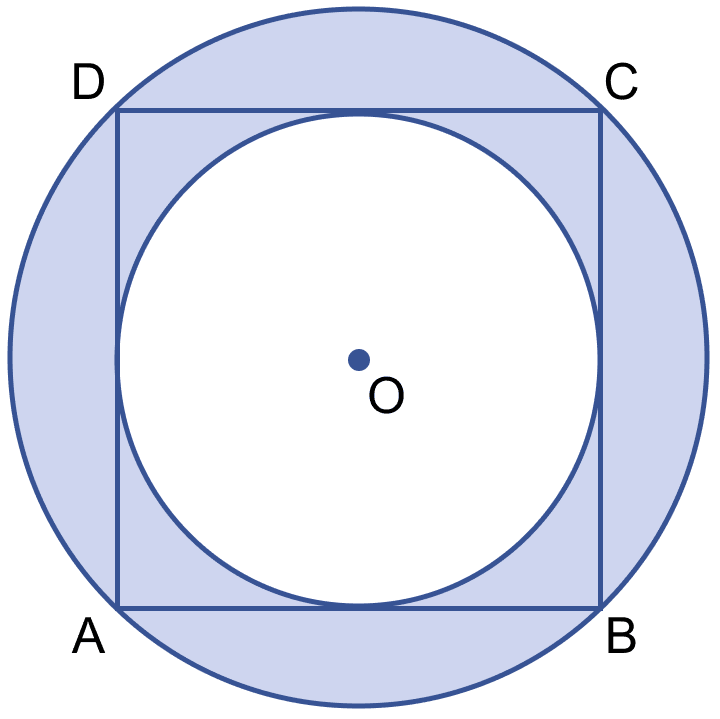
In the adjoining figure, ABCD is a square. Find the ratio between
(i) the circumferences
(ii) the areas of the incircle and the circumcircle of the square.
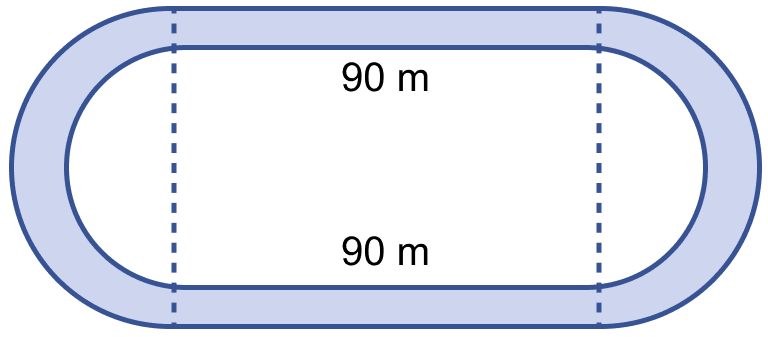
In the figure (ii) given below, the inside perimeter of a practice running track with semi-circular ends and straight parallel sides is 312 m. The length of the straight portion of the track is 90 m. If the track has a uniform width of 2 m throughout, find its area.
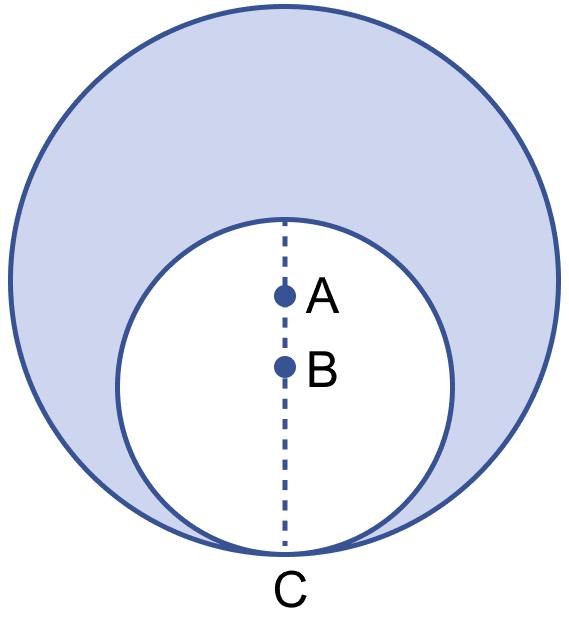
In the figure (i) given below, two circles with centres A and B touch each other at the point C. If AC = 8 cm and AB = 3 cm, find the area of the shaded region.