Mathematics
Tangents AP and AQ are drawn to a circle, with center O, from an exterior point A. Prove that :
∠PAQ = 2∠OPQ
Circles
61 Likes
Answer
We know that,
The tangent at any point of a circle and the radius through this point are perpendicular to each other.
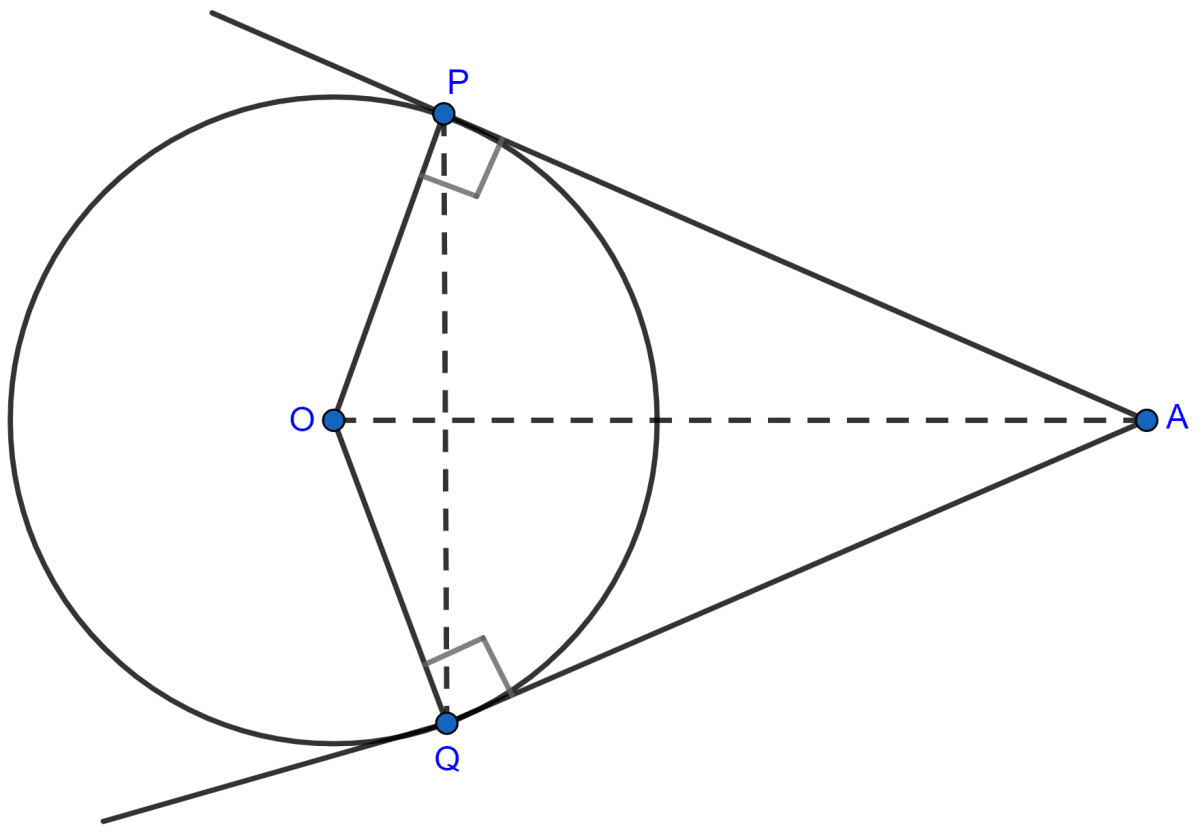
In quadrilateral OPAQ,
∠OPA = ∠OQA = 90°.
∠OPA + ∠OQA + ∠POQ + ∠PAQ = 360° [∵ Sum of angles in quadrilateral = 360°]
90° + 90° + ∠POQ + ∠PAQ = 360°
∠POQ + ∠PAQ = 360° - 180°
∠POQ + ∠PAQ = 180° ……….(1)
In △OPQ,
OP = OQ [Radius of same circle]
∴ ∠OPQ = ∠OQP [Angles opposite to equal sides are equal]
By angle sum property of triangle,
⇒ ∠OPQ + ∠OQP + ∠POQ = 180°
⇒ ∠OPQ + ∠OPQ + ∠POQ = 180°
⇒ 2∠OPQ + ∠POQ = 180° ………(2)
From (1) and (2) we get,
∠POQ + ∠PAQ = 2∠OPQ + ∠POQ
⇒ ∠PAQ = 2∠OPQ.
Hence, proved that ∠PAQ = 2∠OPQ.
Answered By
23 Likes
Related Questions
Radii of two circles are 6.3 cm and 3.6 cm. State the distance between their centers if :
(i) they touch each other externally,
(ii) they touch each other internally.
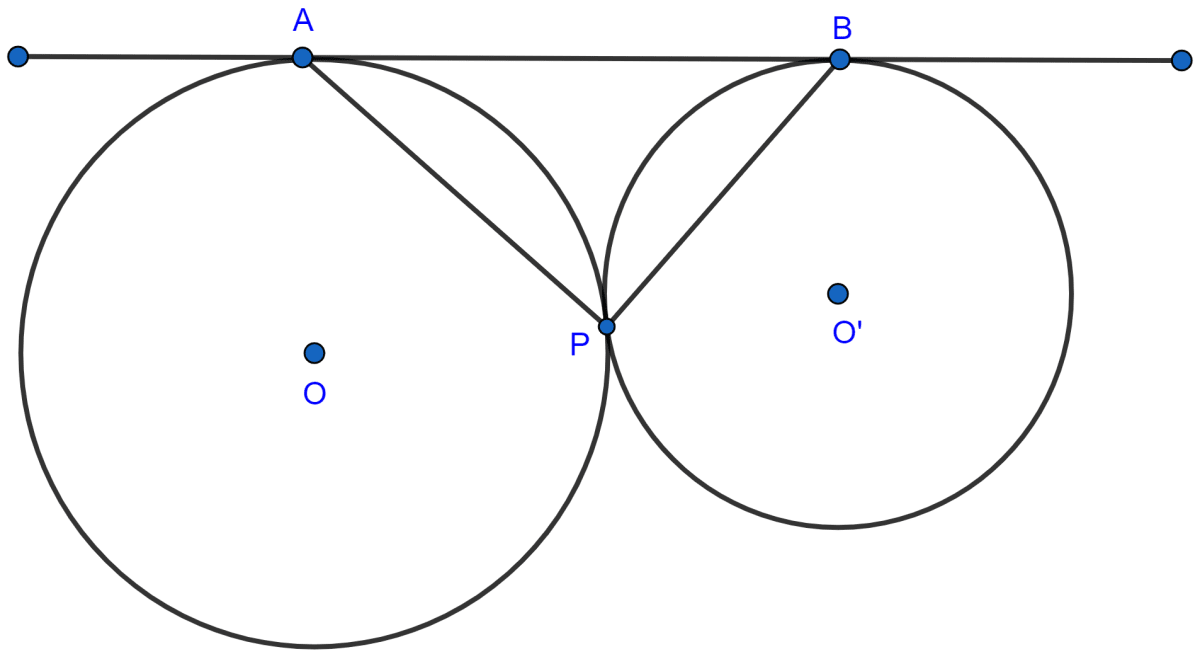
In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that :
(i) tangent at point P bisects AB.
(ii) angle APB = 90°.
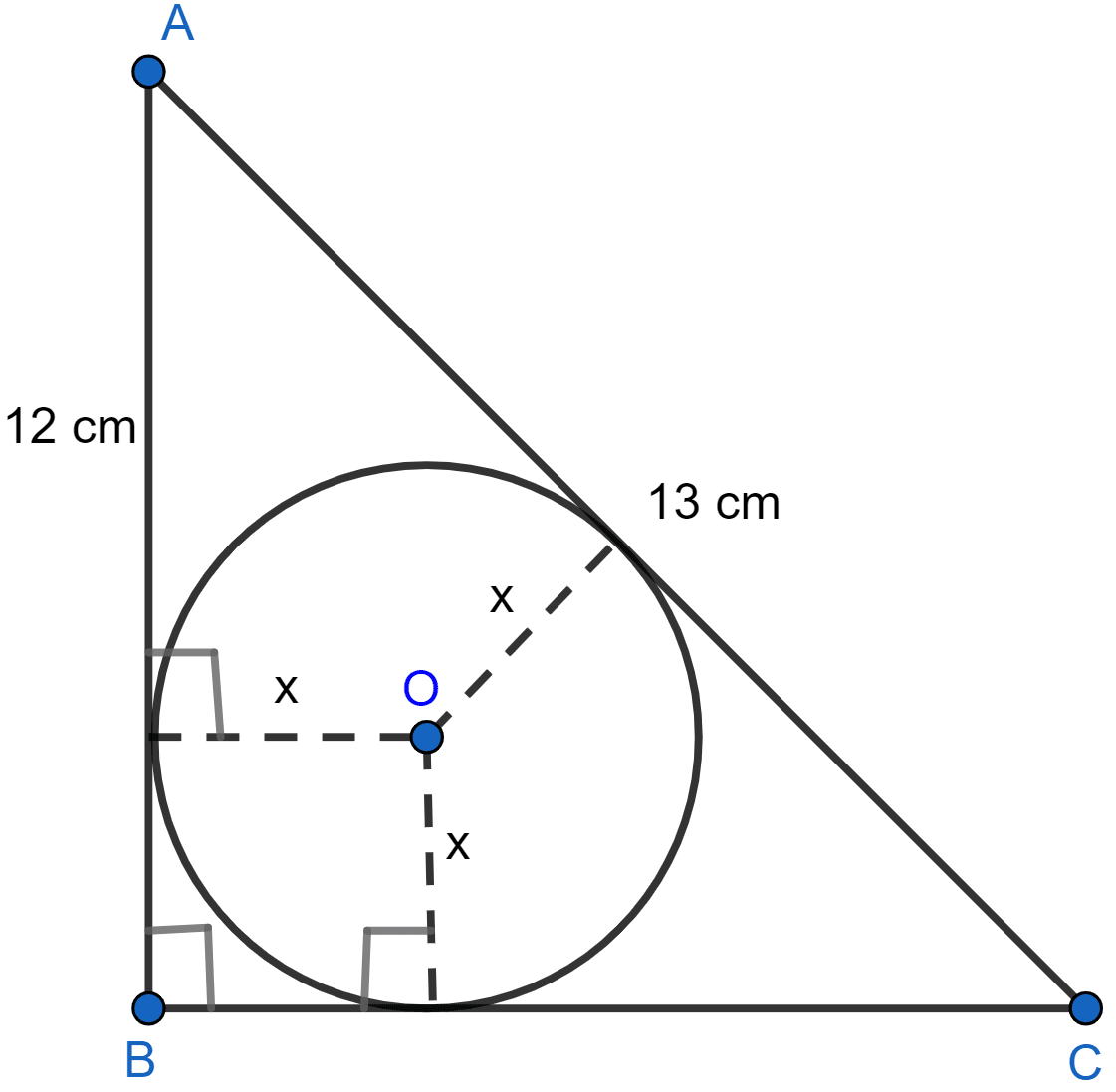
ABC is a right angled triangle with AB = 12 cm and AC = 13 cm. A circle, with center O, has been inscribed inside the triangle. Calculate the value of x, the radius of the inscribed circle.
In the given figure, PT touches the circle with center O at point R. Diameter SQ is produced to meet the tangent TR at P.
Given ∠SPR = x° and ∠QRP = y°;
prove that :
(i) ∠ORS = y°
(ii) write an expression connecting x and y.
