Mathematics
Prove that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.
Answer
Let D and d be the diameter of the circumcircle and incircle.
Also, let R and r be the radius of the circumcircle and incircle.
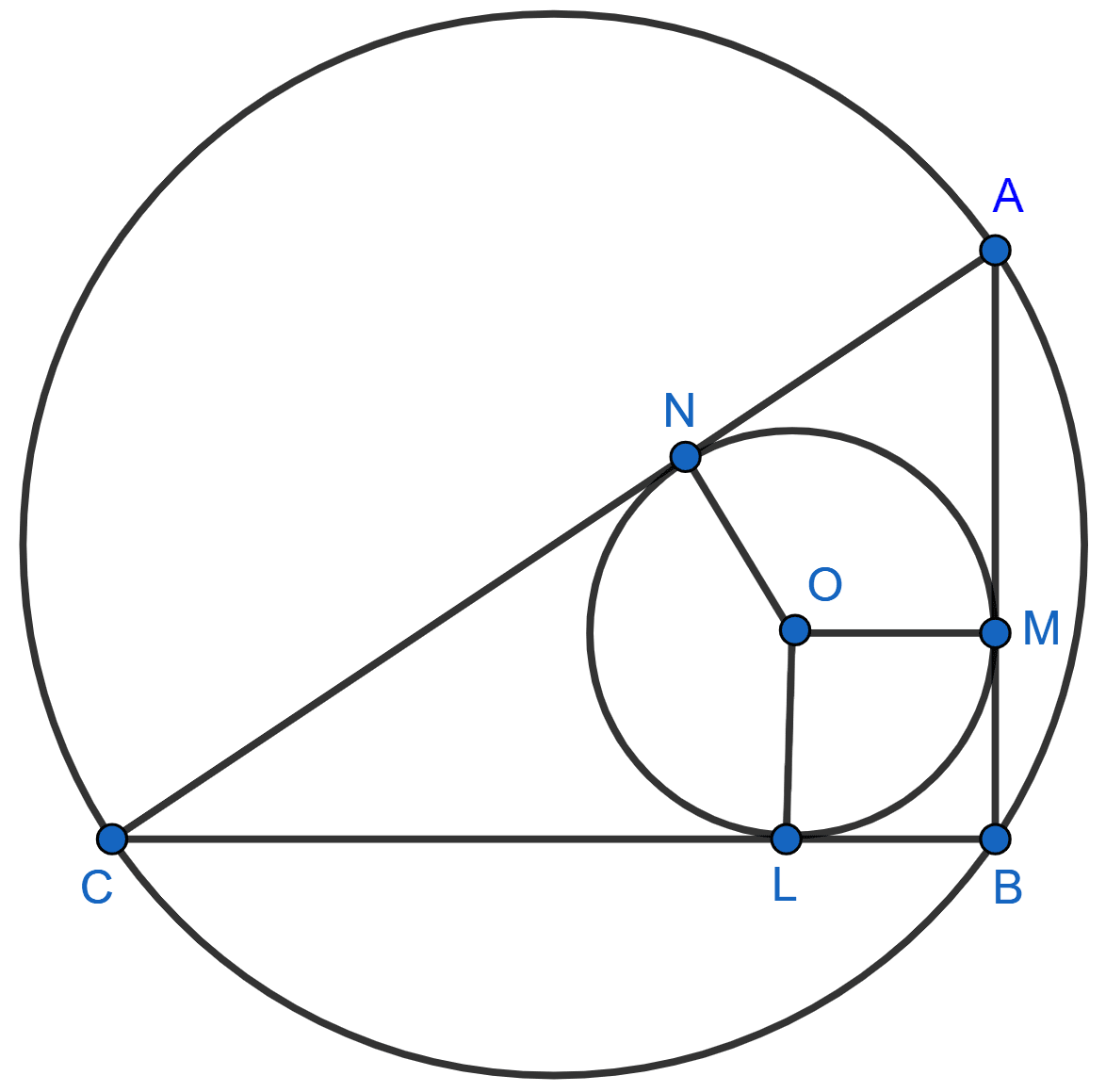
Now, in circumcircle of ∆ABC,
AC is the diameter of the circumcircle i.e. AC = D
From figure,
OL = OM = ON = r
As, tangents from an exterior point to a circle are equal.
Now, from B, BL and BM are the tangents to the incircle.
So, BL = BM = r
Similarly,
AM = AN ………..(1)
CL = CN ………..(2)
Perimeter of ∆ABC = AB + BC + CA.
⇒ AB + BC + CA = AM + BM + BL + CL + CA
= AN + r + r + CN + CA …………[From (1) and (2)]
= AN + CN + 2r + CA
= AC + AC + 2r
= 2AC + 2r
= 2D + d
Hence, proved that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.
Related Questions
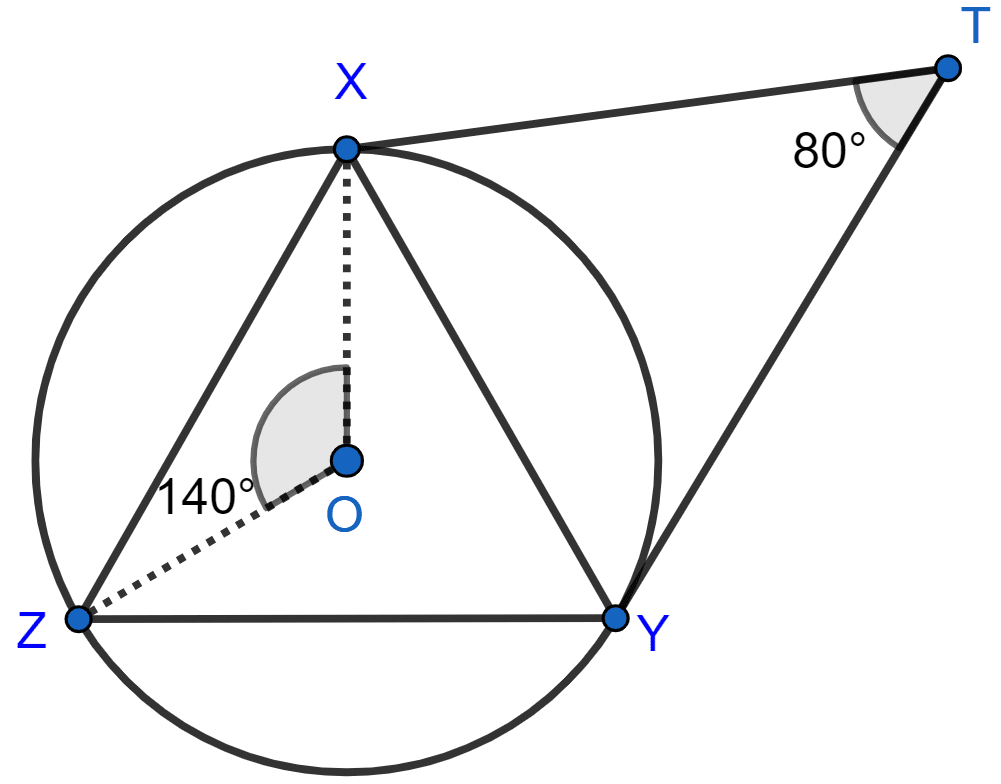
In the figure, given below, O is the center of the circumcircle of triangle XYZ. Tangents at X and Y intersect at point T. Given ∠XTY = 80° and ∠XOZ = 140°, calculate the value of ∠ZXY.
In the given figure, AE and BC intersect each other at point D. If ∠CDE = 90°, AB = 5 cm, BD = 4 cm and CD = 9 cm, find AE.

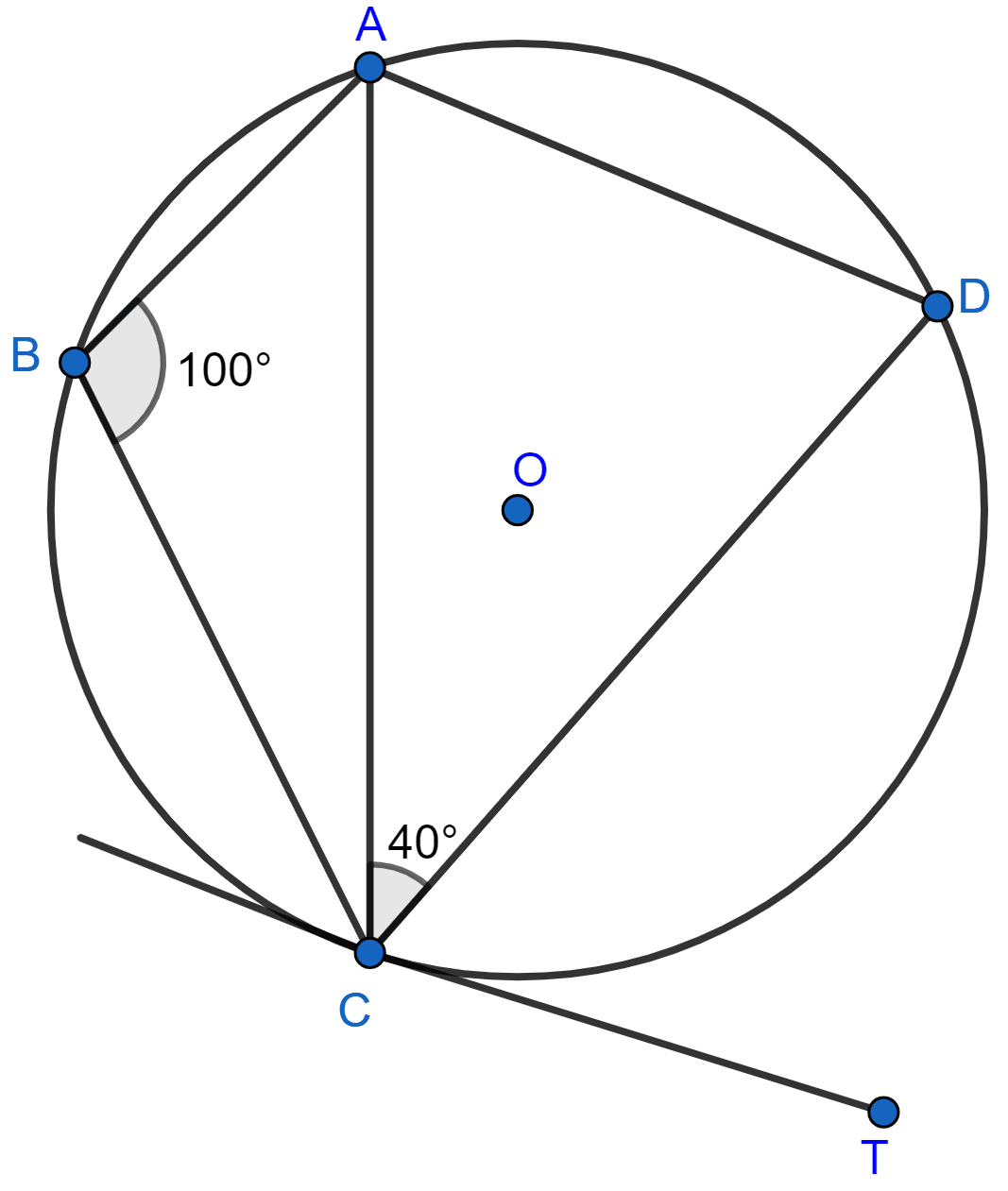
In the given circle with centre O, angle ABC = 100°, ∠ACD = 40° and CT is a tangent to the circle at C. Find ∠ADC and ∠DCT.
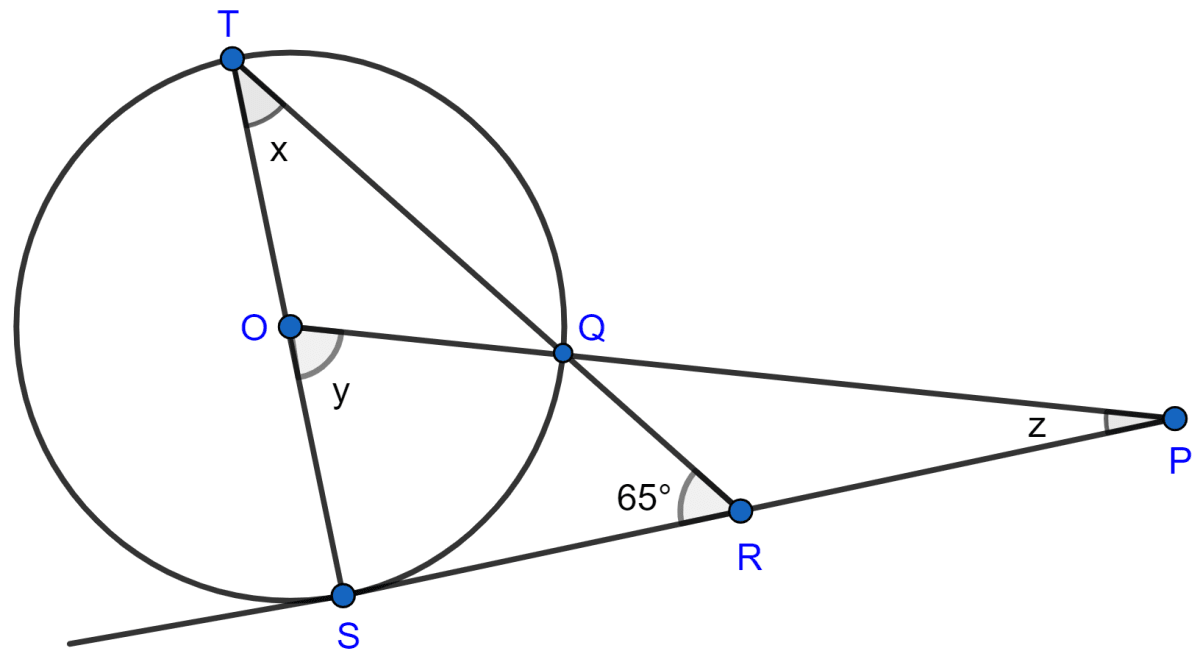
In the figure given below, O is the center of the circle and SP is a tangent. If ∠SRT = 65°, find the values of x, y and z.