Mathematics
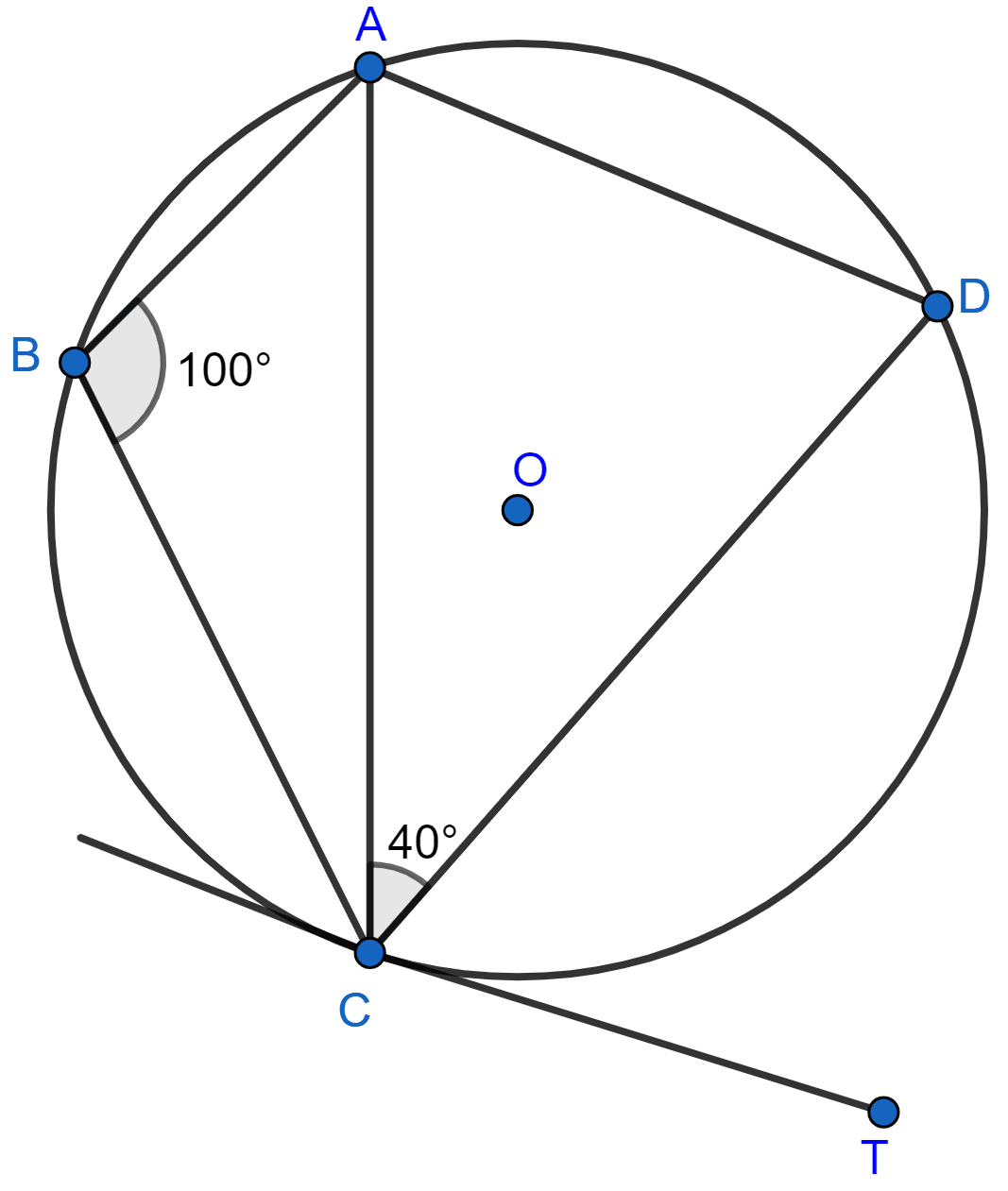
In the given circle with centre O, angle ABC = 100°, ∠ACD = 40° and CT is a tangent to the circle at C. Find ∠ADC and ∠DCT.
Circles
11 Likes
Answer
From figure,
⇒ ∠ADC + ∠ABC = 180° [Sum of opposite angles in a cyclic quadrilateral = 180°]
⇒ ∠ADC + 100° = 180°
⇒ ∠ADC = 180° - 100°
⇒ ∠ADC = 80°.
In △ADC,
⇒ ∠ADC + ∠CAD + ∠ACD = 180° [By angle sum property of triangle]
⇒ 80° + ∠CAD + 40° = 180°
⇒ ∠CAD = 180° - 120° = 60°.
From figure,
⇒ ∠DCT = ∠CAD = 60° [Angles in alternate segment are equal].
Hence, ∠DCT = 60° and ∠ADC = 80°.
Answered By
7 Likes
Related Questions
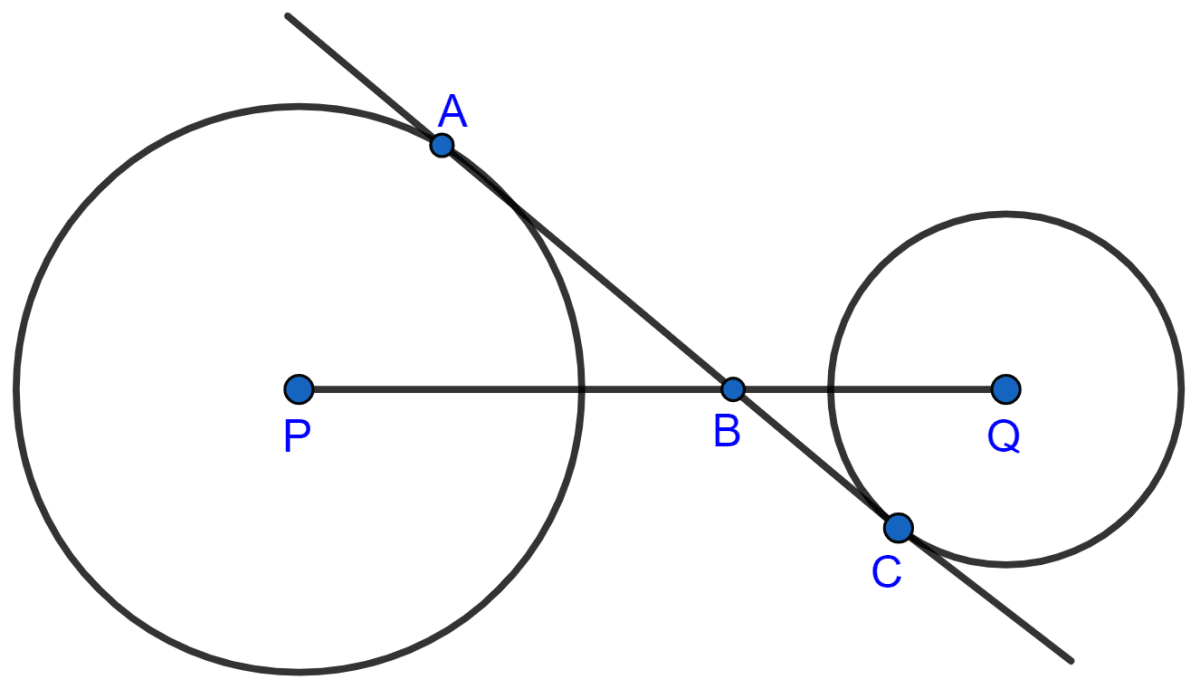
In the figure, given below, AC is a transverse common tangent to two circles with centers P and Q and of radii 6 cm and 3 cm respectively. Given that AB = 8 cm, calculate PQ.
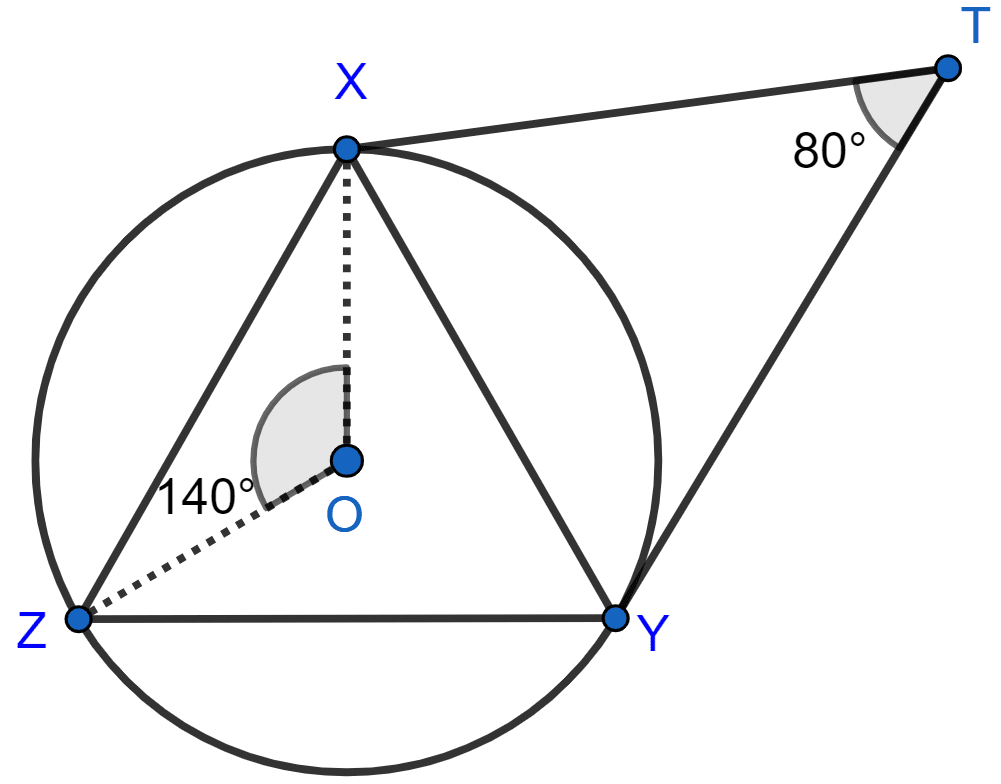
In the figure, given below, O is the center of the circumcircle of triangle XYZ. Tangents at X and Y intersect at point T. Given ∠XTY = 80° and ∠XOZ = 140°, calculate the value of ∠ZXY.
In the given figure, AE and BC intersect each other at point D. If ∠CDE = 90°, AB = 5 cm, BD = 4 cm and CD = 9 cm, find AE.

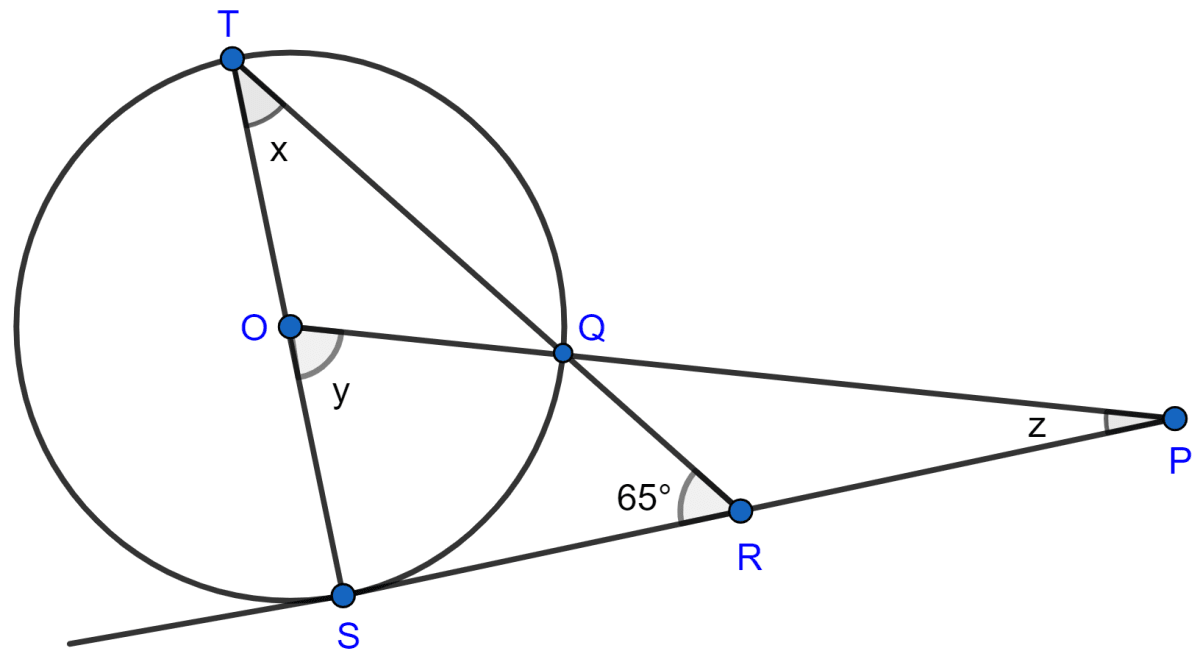
In the figure given below, O is the center of the circle and SP is a tangent. If ∠SRT = 65°, find the values of x, y and z.