Mathematics
Prove that the line segment joining the mid-points of a pair of opposite sides of a parallelogram divides it into two equal parallelograms.
Theorems on Area
58 Likes
Answer
Let us consider ABCD be a parallelogram in which E and F are mid-points of AB and CD. Join EF.
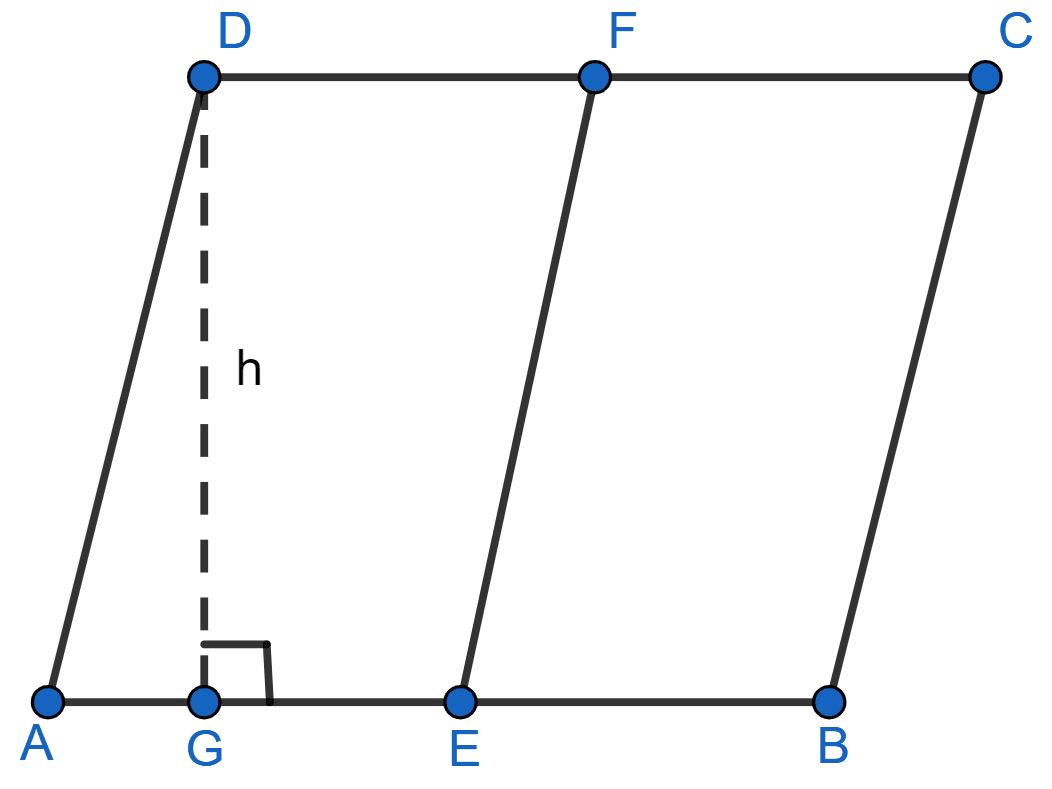
Let us construct DG ⊥ AB and let DG = h, where h is the altitude on side AB.
Area of ||gm ABCD = base × height = AB × h
Area of ||gm AEFD = AE × h = × h …….(i) [Since E is the mid-point of AB]
Area of ||gm EBCF = EB × h = × h …….(ii) [Since E is the mid-point of AB]
From (i) and (ii)
Area of ||gm AEFD = Area of ||gm EBCF.
Hence proved, that the line segment joining the mid-points of a pair of opposite sides of a parallelogram divides it into two equal parallelograms.
Answered By
40 Likes
Related Questions
Prove that the diagonals of a parallelogram divide it into four triangles of equal area.
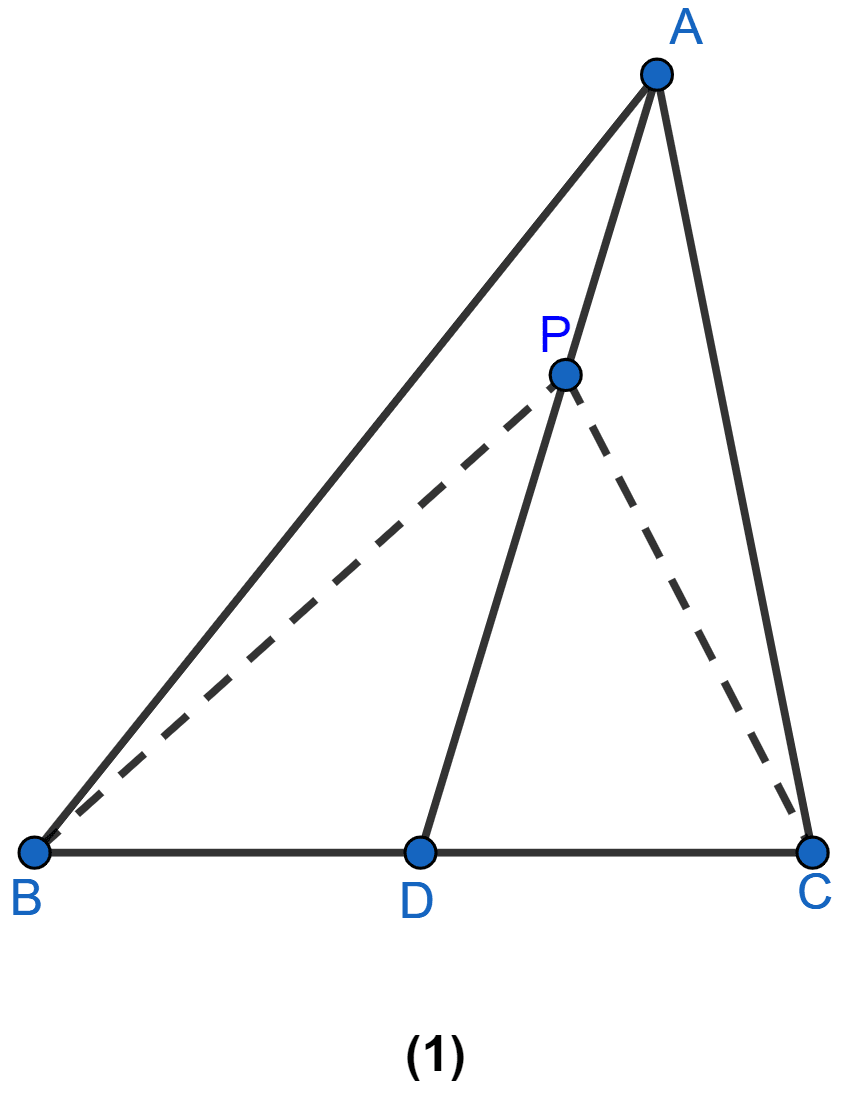
In figure (1) given below, AD is the median of ∆ABC and P is any point on AD. Prove that
(i) Area of ∆PBD = area of ∆PDC.
(ii) Area of ∆ABP = area of ∆ACP.
In the figure (2) given below, DE || BC. Prove that
(i) area of ∆ACD = area of ∆ABE
(ii) area of ∆OBD = area of ∆OCE.

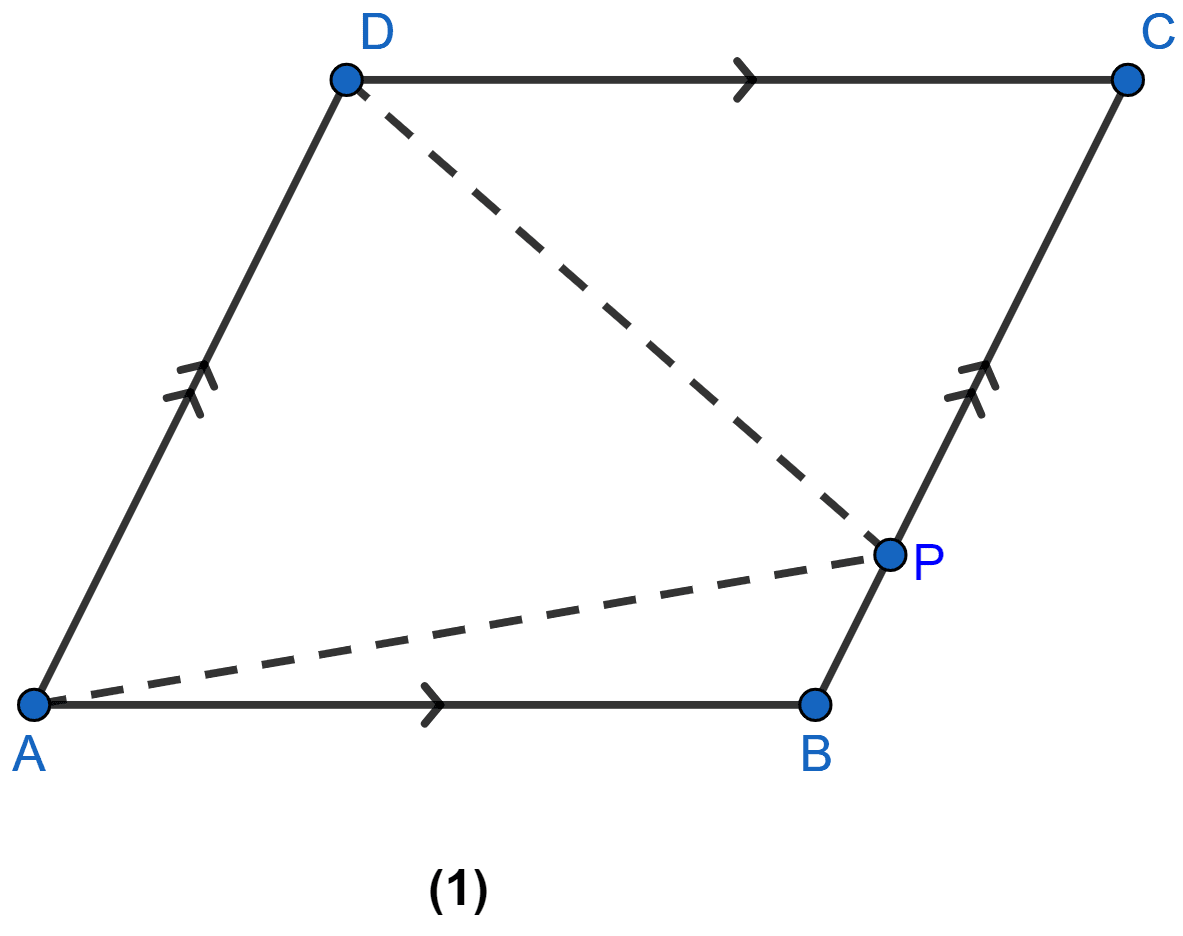
In figure (1) given below, ABCD is a parallelogram and P is any point in BC. Prove that, Area of ∆ABP + area of ∆DPC = Area of ∆APD.