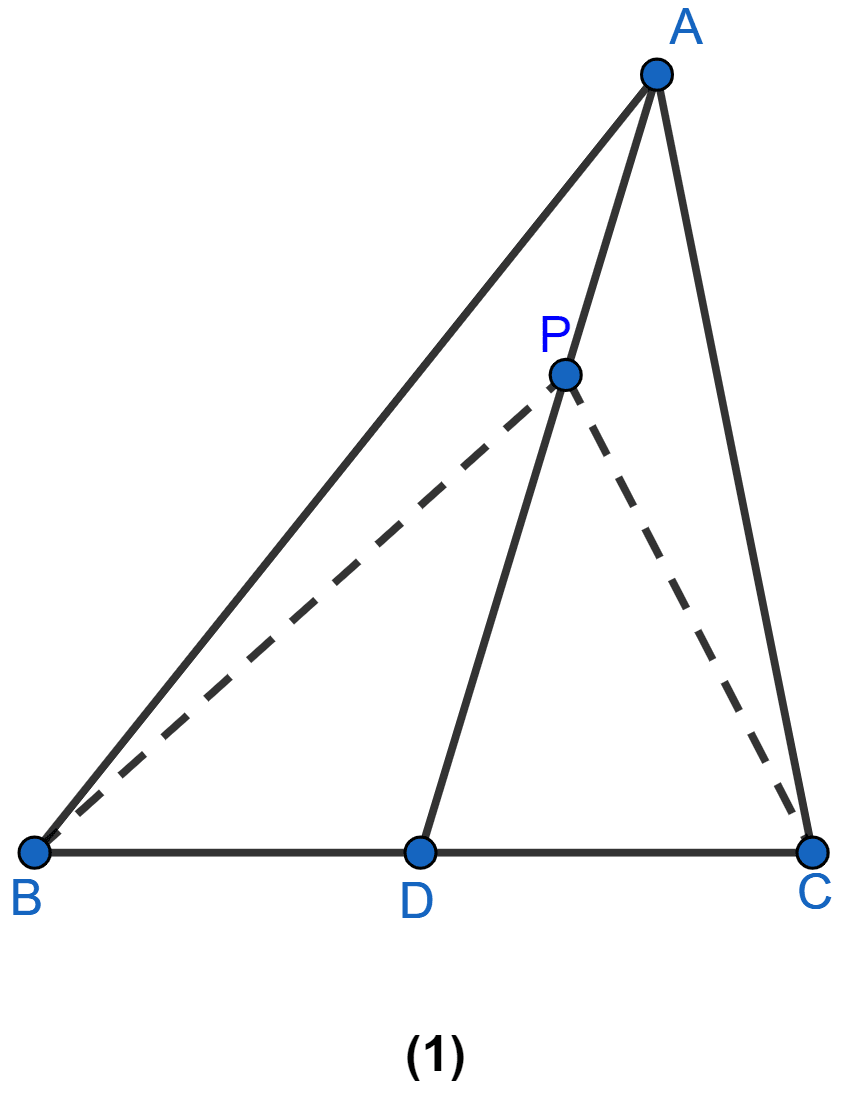
In figure (1) given below, AD is the median of ∆ABC and P is any point on AD. Prove that

(i) Area of ∆PBD = area of ∆PDC.

(ii) Area of ∆ABP = area of ∆ACP.

(i) In ∆ABC,

Area of ∆ABD = Area of ∆ADC [AD is the median] ……(1)

Since, PD is a straight line and base of ∆ABC and ∆PBC,

So PD is median of ∆PBC,

∴ Area of ∆PBD = Area of ∆PDC ……..(2)

Hence, proved that Area of ∆PBD = Area of ∆PDC.

(ii) Subtracting eq. 1 from 2 we get,

⇒ Area of ∆ABD - Area of ∆PBD = Area of ∆ADC - Area of ∆PDC

⇒ Area of ∆ABP = Area of ∆ACP.

Hence proved that Area of ∆ABP = Area of ∆ACP.