Mathematics
Prove that the bisectors of interior angles of a parallelogram form a rectangle.
Rectilinear Figures
1 Like
Answer
Let ABCD be the parallelogram.
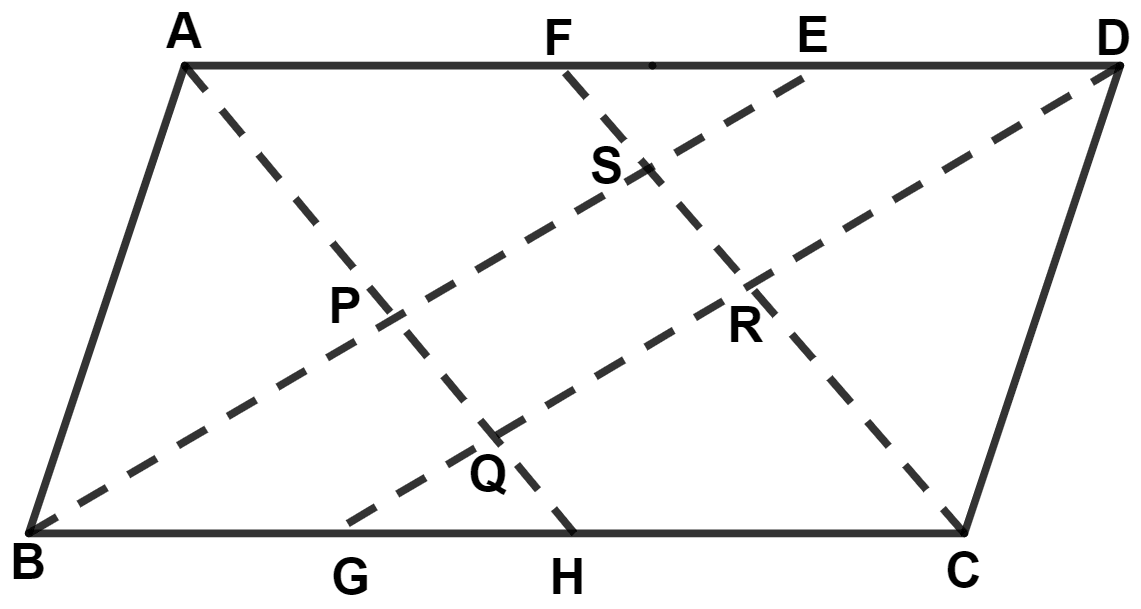
From figure,
AH, BE, CF and DG are bisectors of ∠A, ∠B, ∠C and ∠D respectively.
We know that,
Consecutive angles of parallelogram are supplementary.
Considering ∠A + ∠B = 180°,
⇒
⇒ = 90°
⇒ = 90° …………(1)
Considering ∠B + ∠C = 180°,
⇒
⇒ = 90°
⇒ = 90° …………(2)
Considering ∠C + ∠D = 180°,
⇒
⇒ = 90°
⇒ = 90° ………(3)
Considering ∠D + ∠A = 180°,
⇒
⇒ = 90°
⇒ = 90° ………(4)
In △ PAB,
By angle sum property of triangle,
⇒ ∠PAB + ∠ABP + ∠BPA = 180°
⇒ + ∠BPA = 180°
⇒ 90° + ∠BPA = 180° [From equation (1)]
⇒ ∠BPA = 180° - 90° = 90°.
From figure,
⇒ ∠SPQ = ∠BPA = 90° (Vertically opposite angles are equal)
In △ BSC,
By angle sum property of triangle,
⇒ ∠SBC + ∠SCB + ∠BSC = 180°
⇒ + ∠BSC = 180°
⇒ 90° + ∠BSC = 180° [From equation (2)]
⇒ ∠BSC = 180° - 90° = 90°.
From figure,
⇒ ∠PSR = ∠BSC = 90°.
In △ DRC,
By angle sum property of triangle,
⇒ ∠RCD + ∠CDR + ∠DRC = 180°
⇒ + ∠DRC = 180°
⇒ 90° + ∠DRC = 180° [From equation (3)]
⇒ ∠DRC = 180° - 90° = 90°.
From figure,
⇒ ∠SRQ = ∠DRC = 90° (Vertically opposite angles are equal)
In △ AQD,
By angle sum property of triangle,
⇒ ∠QAD + ∠ADQ + ∠DQA = 180°
⇒ + ∠DQA = 180°
⇒ 90° + ∠DQA = 180° [From equation (4)]
⇒ ∠DQA = 180° - 90° = 90°.
From figure,
⇒ ∠RQP = ∠DQA = 90°.
Since, all the interior angles of quadrilateral PQRS equals to 90°.
∴ PQRS is a rectangle.
Hence, proved that bisectors of interior angles of a parallelogram form a rectangle.
Answered By
1 Like
Related Questions
In the given figure, ABCD is a parallelogram. Prove that : AB = 2BC.

Prove that the bisectors of opposite angles of a parallelogram are parallel.
Prove that the bisectors of the interior angles of a rectangle form a square.
In parallelogram ABCD, the bisectors of angle A meets DC at P and AB = 2AD.
Prove that :
(i) BP bisects angle B.
(ii) Angle APB = 90°.