Mathematics
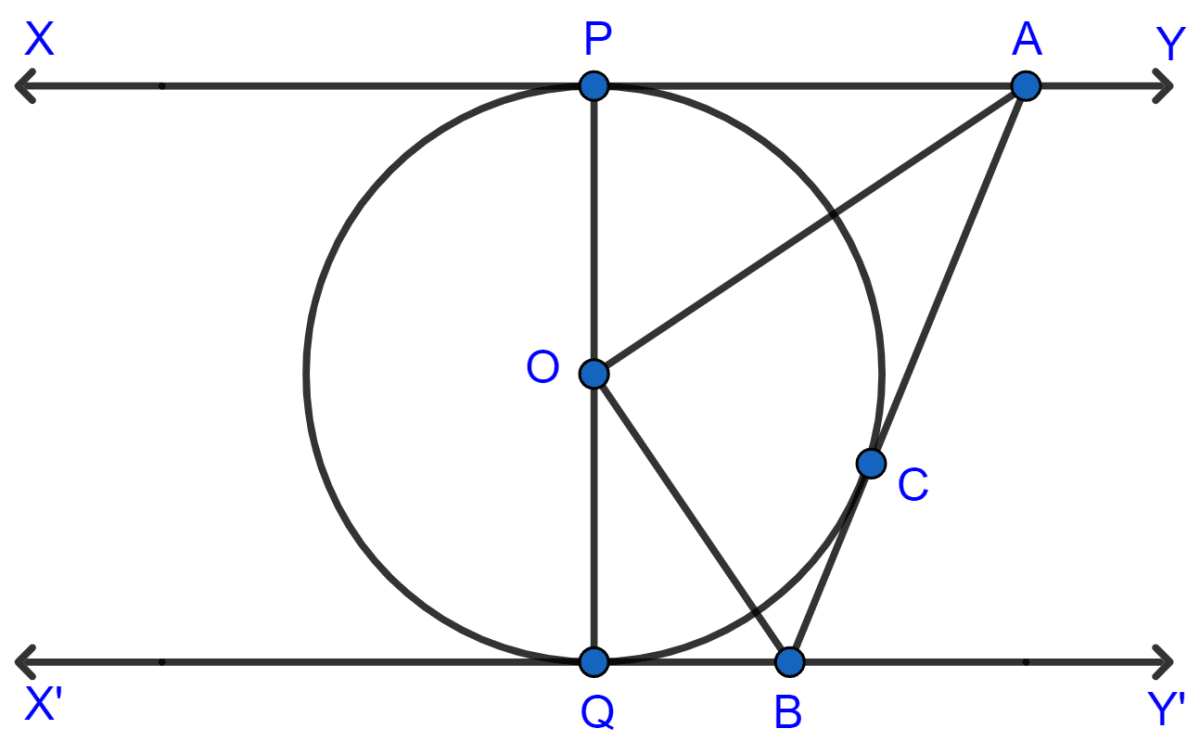
In the given figure, XY and X'Y' are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersecting XY at A and X'Y' at B. Prove that ∠AOB = 90°.
Answer
Draw a line between points O and C.
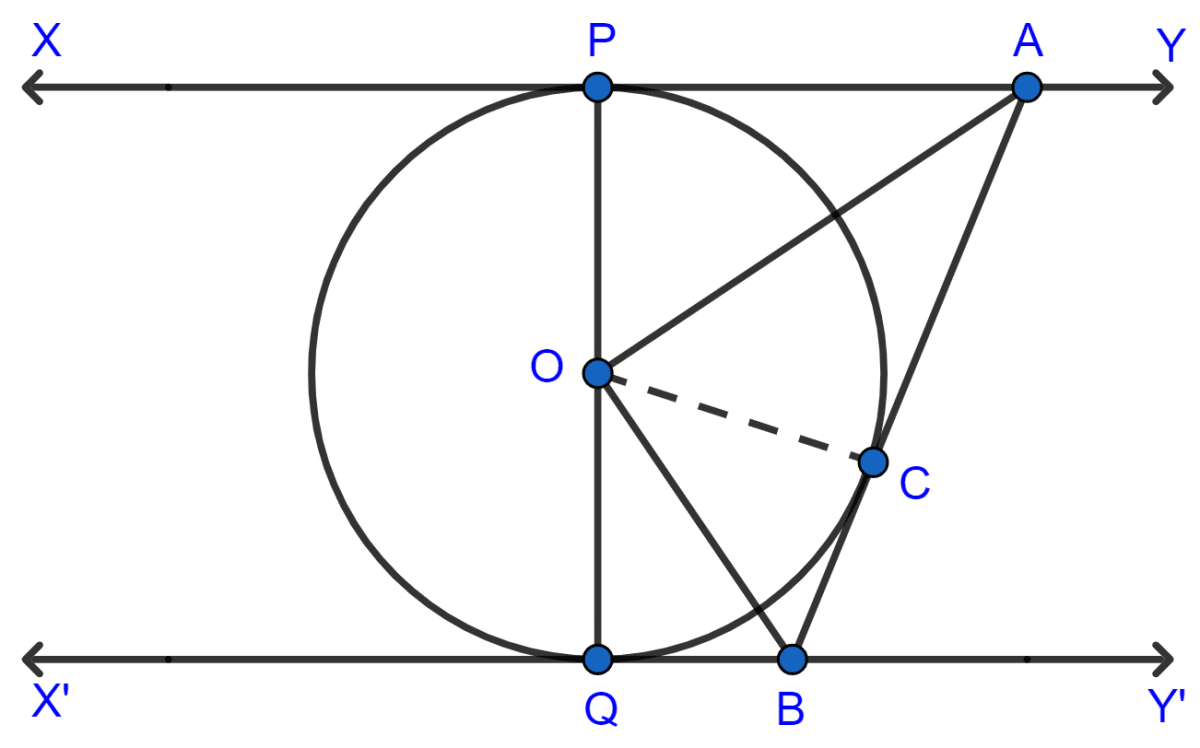
In Δ OPA and Δ OCA,
⇒ OP = OC (Radii of the circle)
⇒ AP = AC (The lengths of tangents drawn from an external point to a circle are always equal.)
⇒ AO = AO (Common)
∴ Δ OPA ≅ Δ OCA (By SSS axiom)
By C.P.C.T.,
∠POA = ∠AOC ……….. (1)
In Δ COB and Δ BOQ,
⇒ OQ = OC (Radii of the circle)
⇒ BC = BQ (The lengths of tangents drawn from an external point to a circle are always equal.)
⇒ OB = OB (Common)
∴ Δ COB ≅ Δ BOQ (By SSS axiom)
By C.P.C.T.
∴ ∠COB = ∠BOQ ……….. (2)
PQ is a diameter, hence a straight line and ∠POQ = 180°
From figure,
⇒ ∠POQ = ∠POA + ∠AOC + ∠COB + ∠BOQ
⇒ ∠POA + ∠AOC + ∠COB + ∠BOQ = 180°
⇒ ∠AOC + ∠AOC + ∠COB + ∠COB = 180° [From equation (1) and (2)]
⇒ 2∠AOC + 2∠COB = 180°
⇒ 2(∠AOC + ∠COB) = 180°
⇒ ∠AOC + ∠COB = 90°
From the figure,
⇒∠AOC + ∠COB = ∠AOB
∴ ∠AOB = 90°
Hence proved that ∠AOB = 90°.
Related Questions
Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the centre.
Prove that the parallelogram circumscribing a circle is a rhombus.
Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
A quadrilateral ABCD is drawn to circumscribe a circle. Prove that :
AB + CD = AD + BC