Mathematics
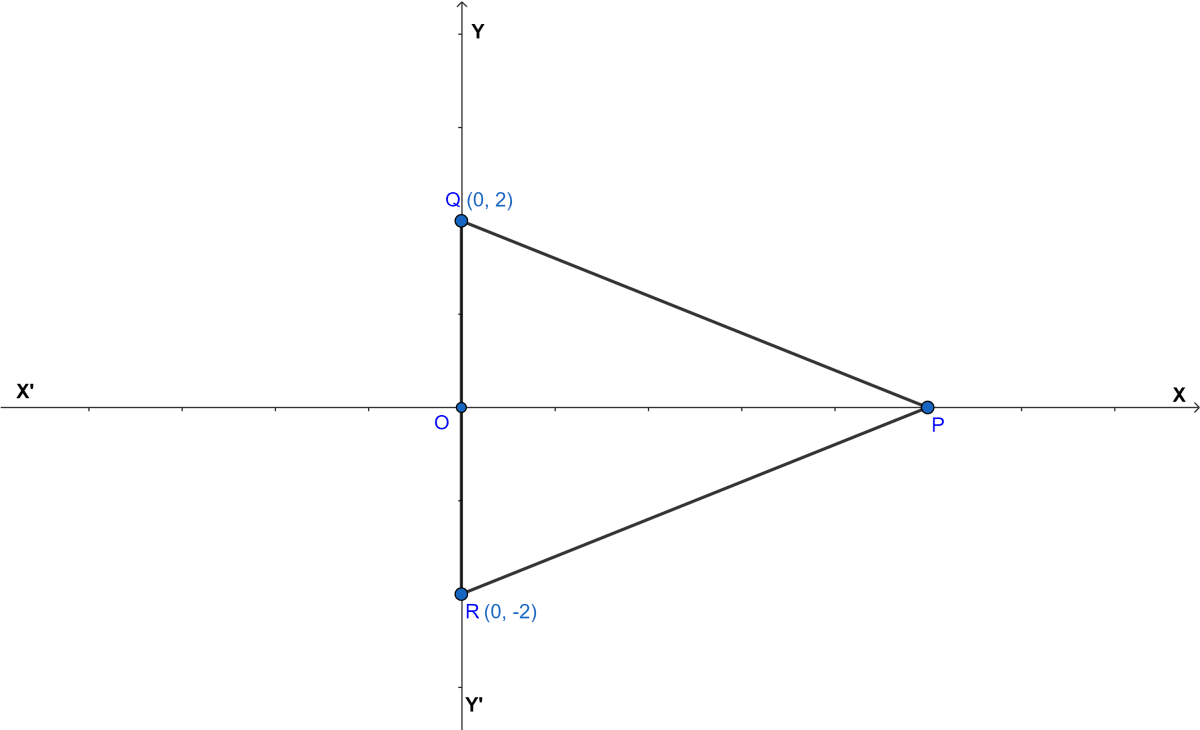
In the given figure, △PQR is equilateral. If the coordinates of the points Q and R are (0, 2) and (0, -2) respectively, find the coordinates of the point P.
Coordinate Geometry
35 Likes
Answer
Given, PQR is an equilateral triangle in which Q(0, 2) and R(0, -2) and O = (0, 0).
Let (x, 0) be the coordinates of P. [As, P lies on x axis, so y-coordinate is zero.]
By distance formula,
2 - x1)^2 + (y2 - y1)^2} \\[1em] = \sqrt{(0 - 0)^2 + (-2 - 2)^2} \\[1em] = \sqrt{0 + (-4)^2} \\[1em] = \sqrt{16} \\[1em] = 4 \text{ units}. \\[1em] \Rightarrow OQ = \sqrt{(x2 - x1)^2 + (y2 - y1)^2} \\[1em] = \sqrt{(0 - 0)^2 + (2 - 0)^2} \\[1em] = \sqrt{0 + (2)^2} \\[1em] = \sqrt{4} \\[1em] = 2 \text{ units}.
PQ = PR = QR = 4.
OQ = 2
In right angle triangle POQ,
⇒ PQ2 = OP2 + OQ2 [By pythagoras theorem]
⇒ 42 = OP2 + 22
⇒ 16 = OP2 + 4
⇒ OP2 = 16 - 4
⇒ OP2 = 12
⇒ OP = .
Since, P lies on x-axis and OP = .
Hence, the coordinates of P are (, 0).
Answered By
18 Likes
Related Questions
In the adjoining figure, ABCD is a rectangle with length 6 units and breadth 3 units. If O is the mid-point of AB, find the coordinates of A, B, C and D.

The adjoining figure shows an equilateral triangle OAB with each side = 2a units. Find the coordinates of the vertices.
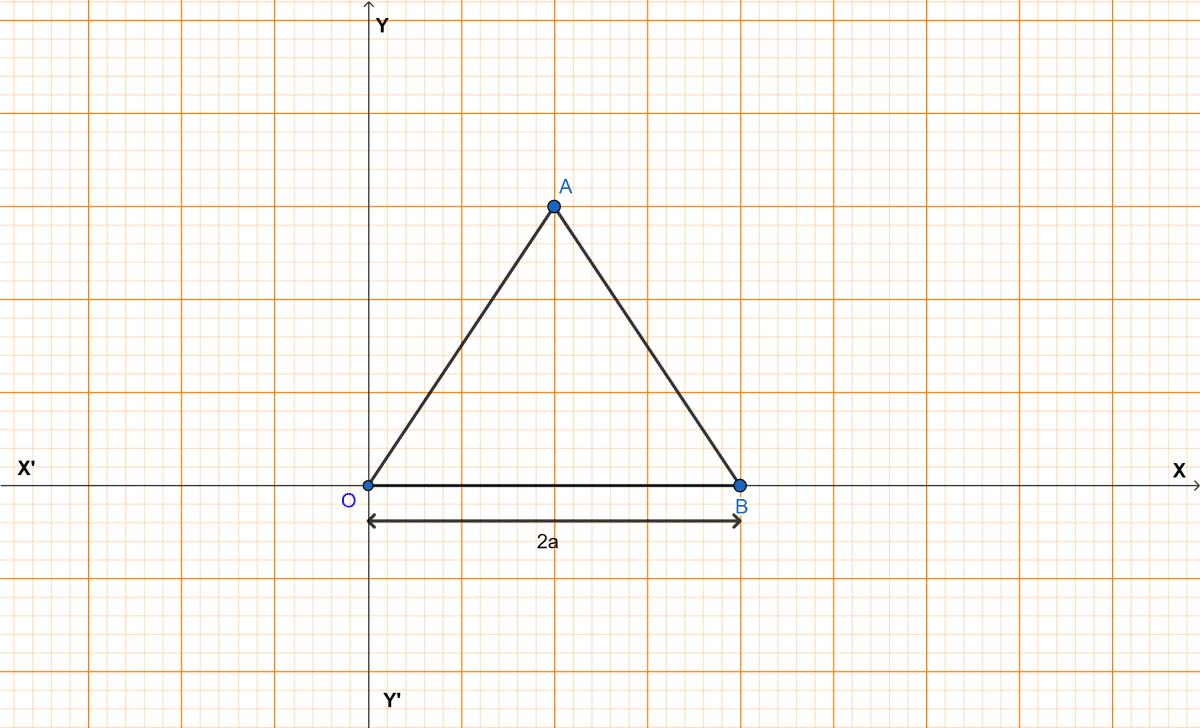
Draw the graph of the following linear equation :
2x + y + 3 = 0
Draw the graph of the following linear equation :
x - 5y - 4 = 0