Mathematics
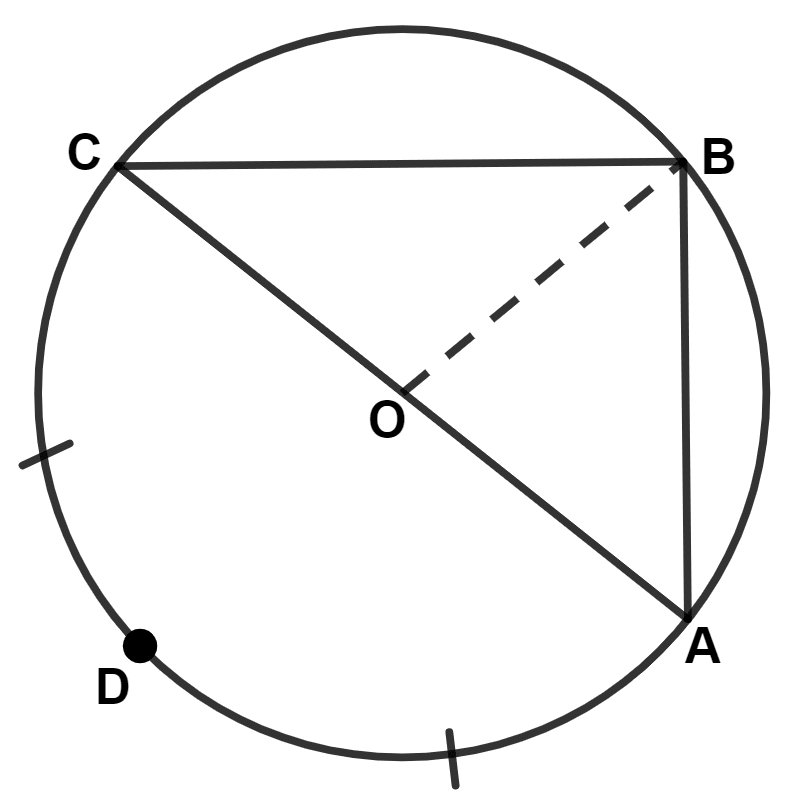
In the given figure, O is the center of a circle. AB is the side of a square and BC is side of a regular hexagon. Also arc AD = arc CD. Angle DOC is equal to :
150°
105°
130°
210°
Circles
9 Likes
Answer
Join OD.
Since, AB is the side of square.
∴ ∠AOB = = 90°.
Since, BC is the side of regular hexagon.
∴ ∠BOC = = 60°.
We know that,
Equal arcs subtends equal angles at the center.
Since, arc AD = arc CD
∴ ∠AOD = ∠COD = x (let)
From figure,
⇒ ∠AOD + ∠COD + ∠AOB + ∠BOC = 360°
⇒ x + x + 90° + 60° = 360°
⇒ 2x + 150° = 360°
⇒ 2x = 360° - 150°
⇒ 2x = 210°
⇒ x = = 105°.
Hence, Option 2 is the correct option.
Answered By
5 Likes
Related Questions
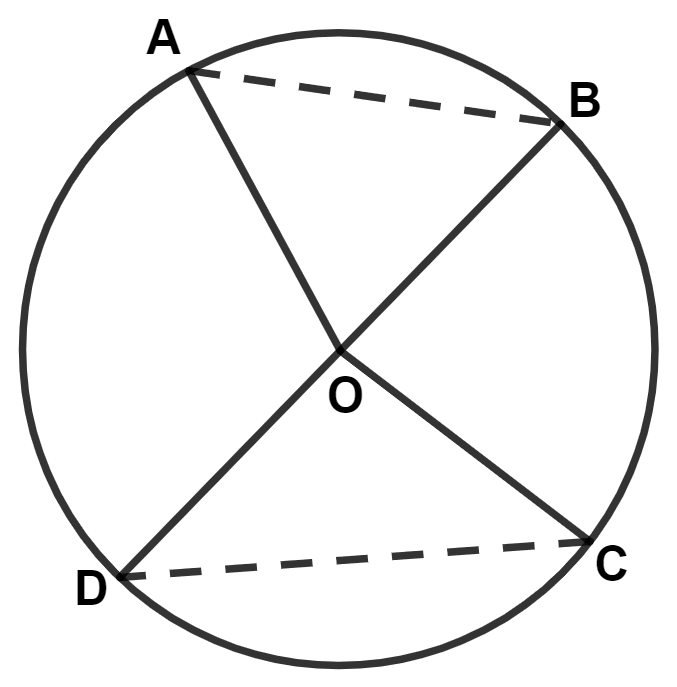
In the given figure, O is the center of the circle and chord AB : chord CD = 3 : 5. If angle AOB = 60°, angle COD is equal to :
60°
120°
90°
100°
In the given figure, O is the center of the circle and angle OAB = 55°, then angle ACB is equal to :
55°
35°
70°
30°
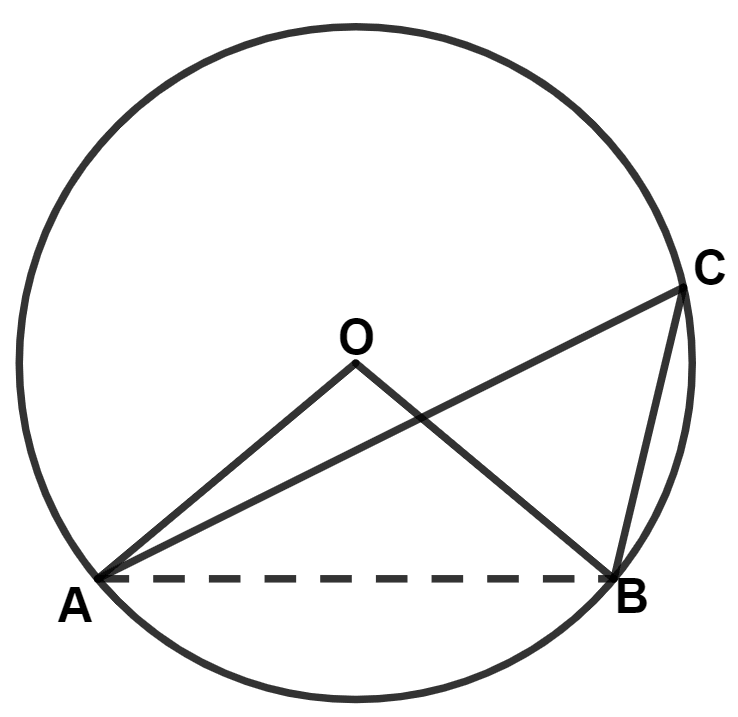
In the given figure, O is the center of the circle, AB is side of a regular pentagon, then angle ACB is equal to :
36°
72°
50°
40°

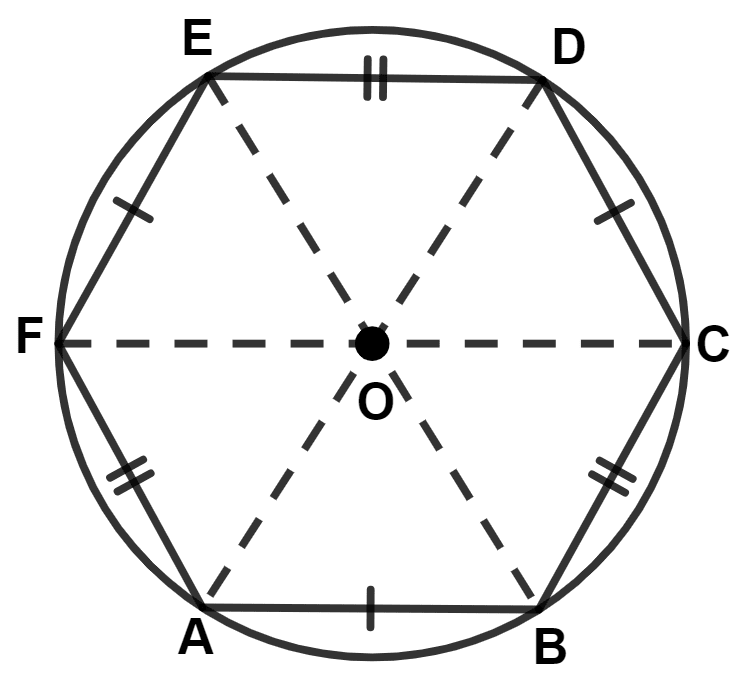
In the given figure, O is the center of the circle, chords AB, CD and EF are equal whereas chords BC, DE and FA are separately equal. The angle AOC is equal to :
80°
100°
90°
120°